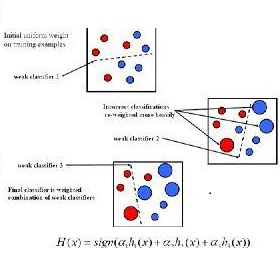
One of the most popular ML algorithms, AdaBoost, can be derived from the dual of a relative entropy minimization problem subject to the fact that the positive weights on the examples sum to one. Essentially, harder examples receive higher probabilities. We generalize this setup to the recently introduced {\it tempered exponential measure}s (TEMs) where normalization is enforced on a specific power of the measure and not the measure itself. TEMs are indexed by a parameter $t$ and generalize exponential families ($t=1$). Our algorithm, $t$-AdaBoost, recovers AdaBoost~as a special case ($t=1$). We show that $t$-AdaBoost retains AdaBoost's celebrated exponential convergence rate when $t\in [0,1)$ while allowing a slight improvement of the rate's hidden constant compared to $t=1$. $t$-AdaBoost partially computes on a generalization of classical arithmetic over the reals and brings notable properties like guaranteed bounded leveraging coefficients for $t\in [0,1)$. From the loss that $t$-AdaBoost minimizes (a generalization of the exponential loss), we show how to derive a new family of {\it tempered} losses for the induction of domain-partitioning classifiers like decision trees. Crucially, strict properness is ensured for all while their boosting rates span the full known spectrum. Experiments using $t$-AdaBoost+trees display that significant leverage can be achieved by tuning $t$.
翻译:暂无翻译