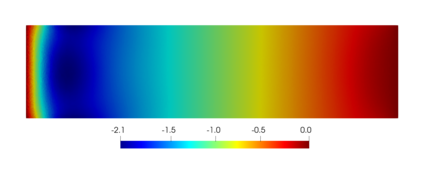
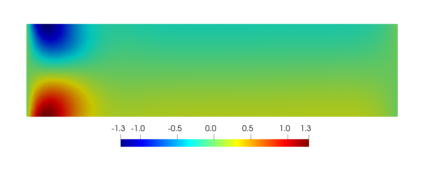
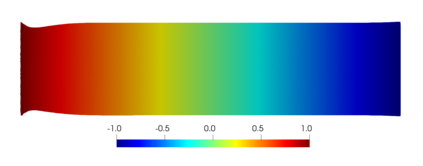
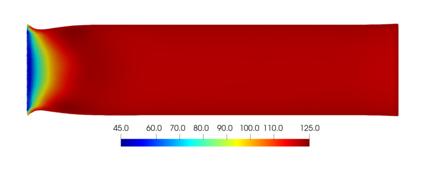
We present and analyze a discontinuous Galerkin method for the numerical modelling of the fully-coupled quasi-static thermo-poroelastic problem. In particular, for the space discretization we introduce a discontinuous Galerkin method over polygonal and polyhedral grids and we present the stability analysis via two different approaches: first exploiting the Poincar\`e's inequality and second using the generalized inf-sup condition. Error estimates are derived for the resulting semi-discrete formulation in a suitable mesh dependent energy norm. Numerical simulations are presented in order to validate the theoretical analysis and to show the application of the model to a realistic case test.
翻译:我们提出并分析一种不连续的Galerkin方法,用于对完全混合的准静态热聚氨酯问题进行数字建模。特别是,对于空间离散,我们采用一种不连续的Galerkin方法,对多边形和多面形网格进行,我们通过两种不同的方法提出稳定性分析:首先利用Poincar ⁇ e的不平等,其次是使用一般的内分泌条件;在适当的网状依附能源规范中,对由此产生的半分解配方得出错误估计。进行数字模拟是为了验证理论分析,并展示模型在现实案例测试中的应用情况。