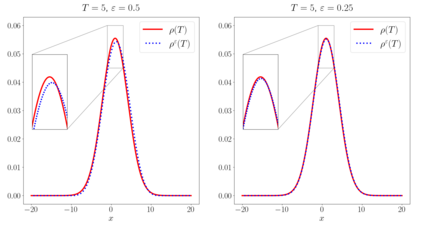
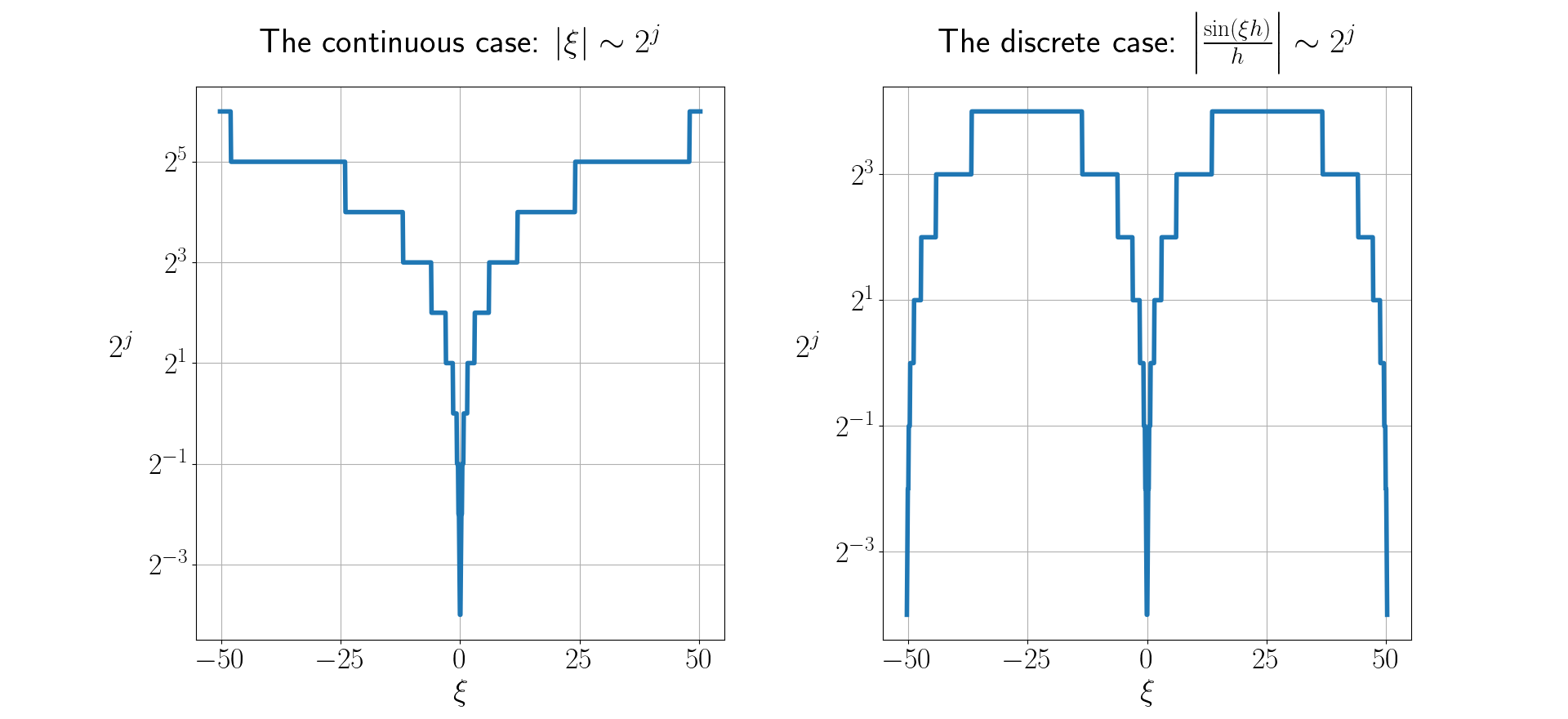
In this paper, we analyze the preservation of asymptotic properties of partially dissipative hyperbolic systems when switching to a discrete setting. We prove that one of the simplest consistent and unconditionally stable numerical methods - the central finite difference scheme - preserves both the asymptotic behaviour and the parabolic relaxation limit of one-dimensional partially dissipative hyperbolic systems which satisfy the Kalman rank condition. The large time asymptotic-preserving property is achieved by conceiving time-weighted perturbed energy functionals in the spirit of the hypocoercivity theory. For the relaxation-preserving property, drawing inspiration from the observation that solutions in the continuous case exhibit distinct behaviours in low and high frequencies, we introduce a novel discrete Littlewood-Paley theory tailored to the central finite difference scheme. This allows us to prove Bernstein-type estimates for discrete differential operators and leads to a new relaxation result: the strong convergence of the discrete linearized compressible Euler system with damping towards the discrete heat equation, uniformly with respect to the mesh parameter.
翻译:暂无翻译