Consistency of MLE for partially observed diffusions, with application in market microstructure modeling

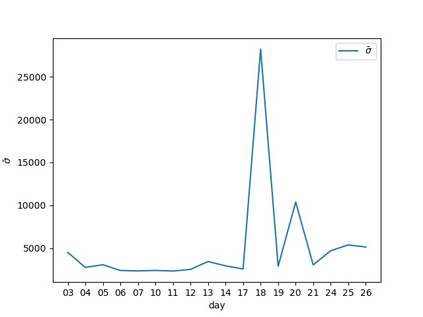
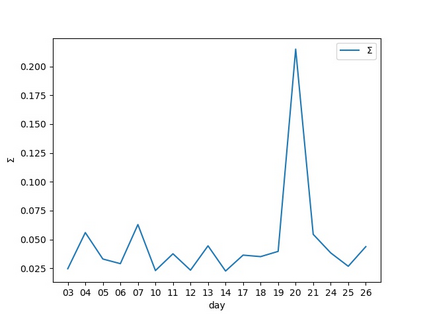
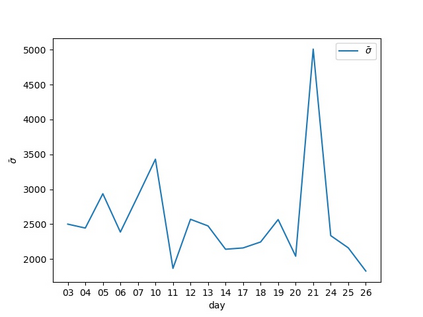
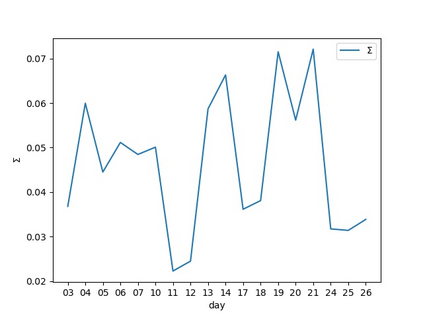
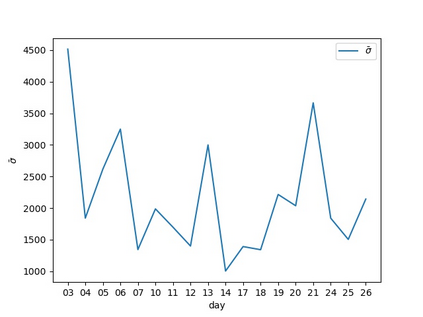
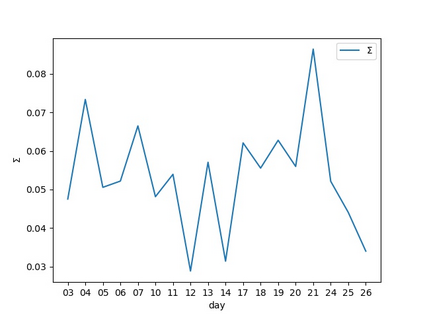
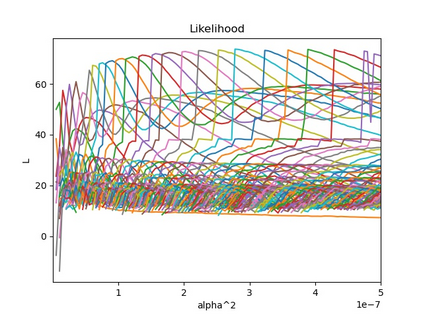
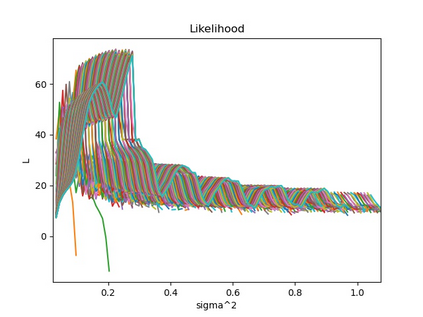
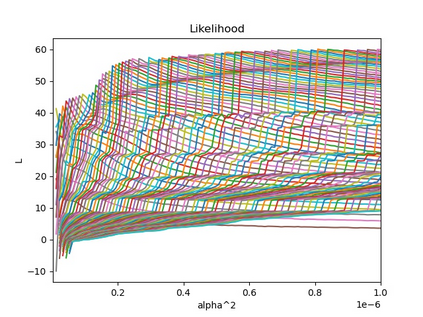
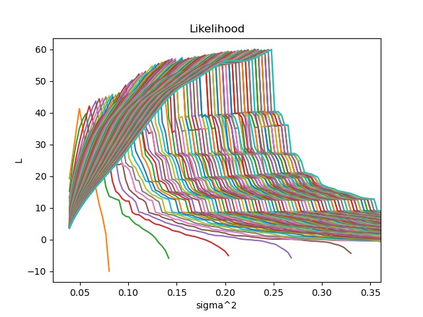
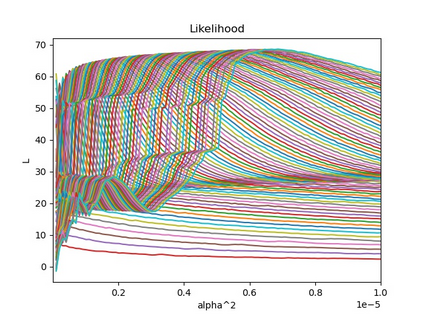
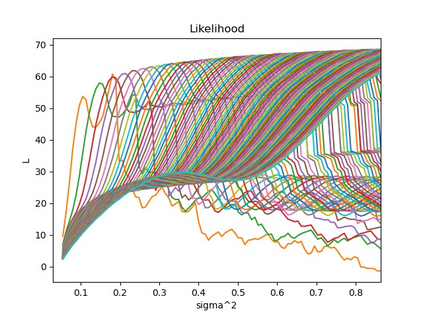
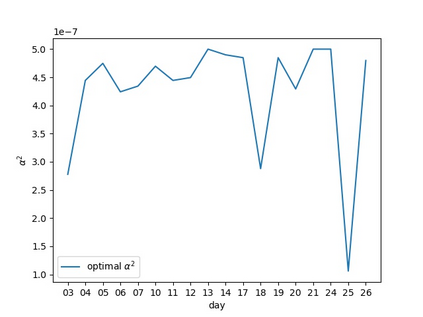
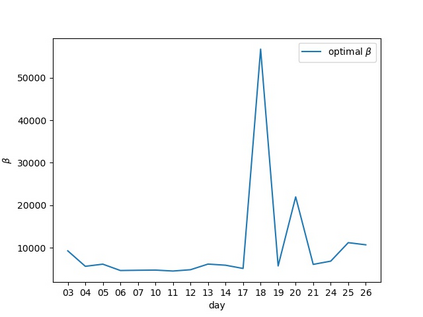
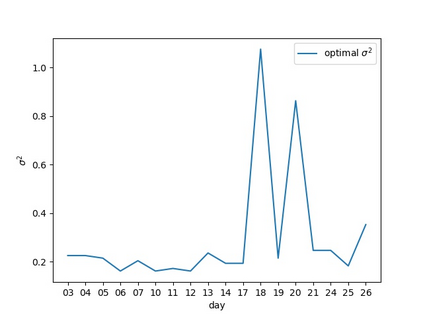
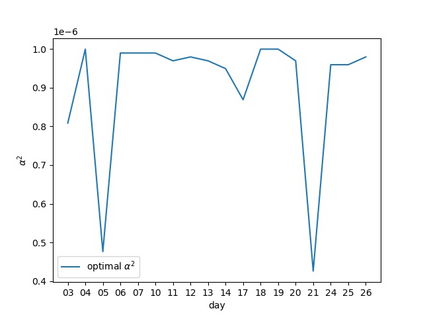
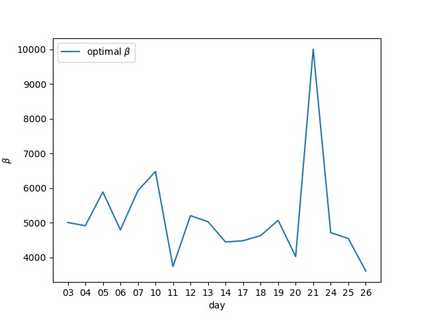
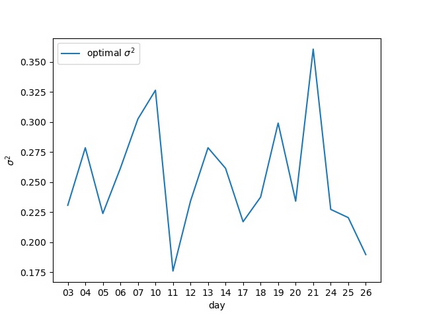
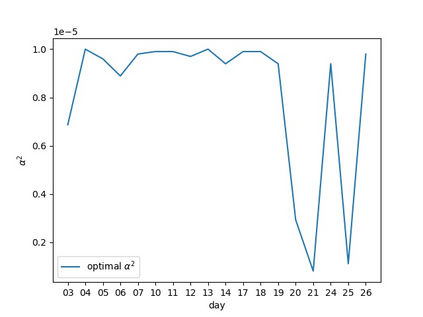
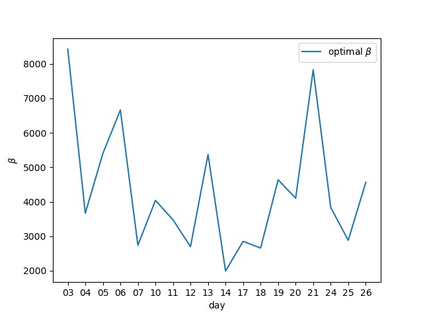
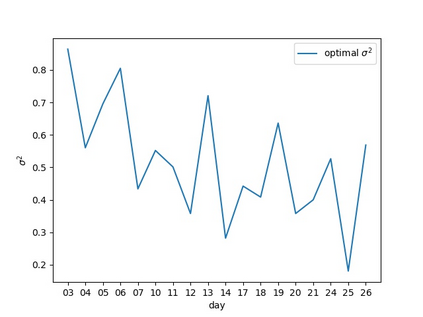
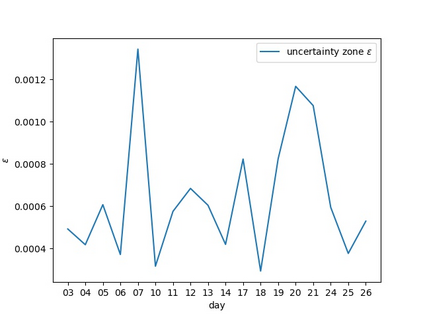
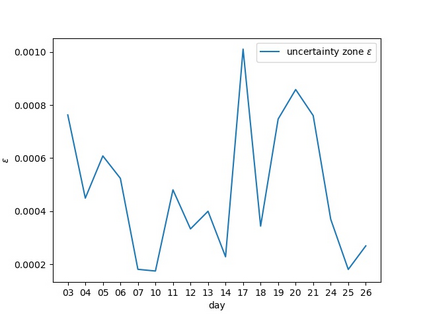
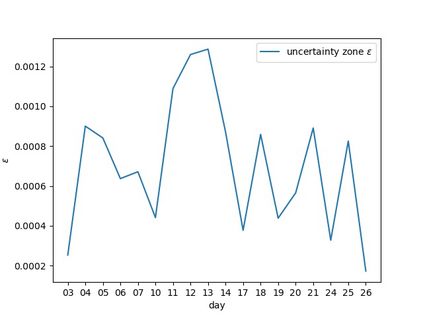
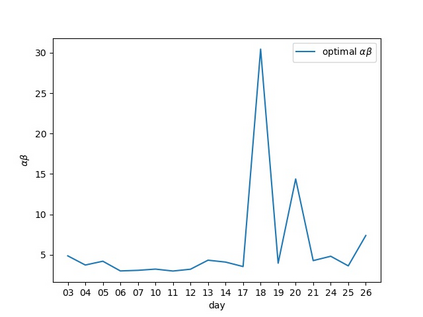
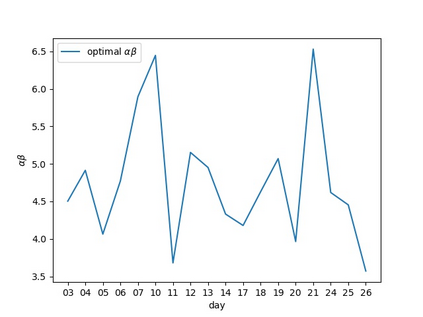
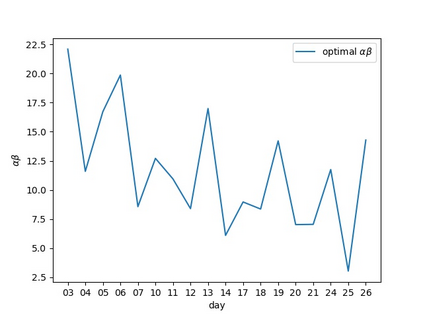
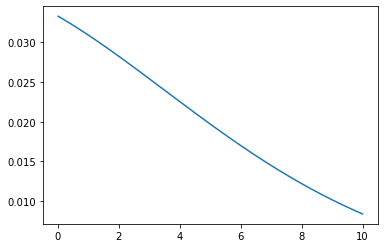
This paper presents a tractable sufficient condition for the consistency of maximum likelihood estimators (MLEs) in partially observed diffusion models, stated in terms of stationary distributions of the associated test processes, under the assumption that the set of unknown parameter values is finite. We illustrate the tractability of this sufficient condition by verifying it in the context of a latent price model of market microstructure. Finally, we describe an algorithm for computing MLEs in partially observed diffusion models and test it on historical data to estimate the parameters of the latent price model.