Time Series Estimation of the Dynamic Effects of Disaster-Type Shock

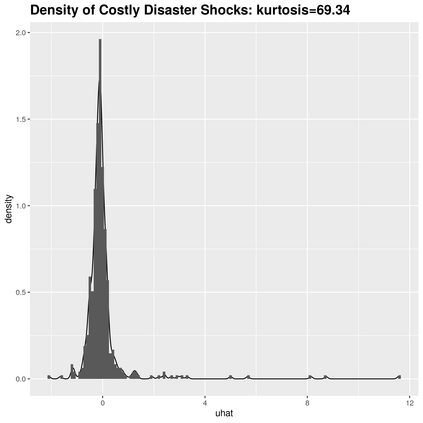
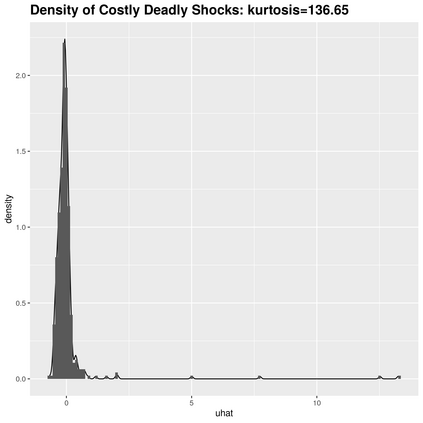
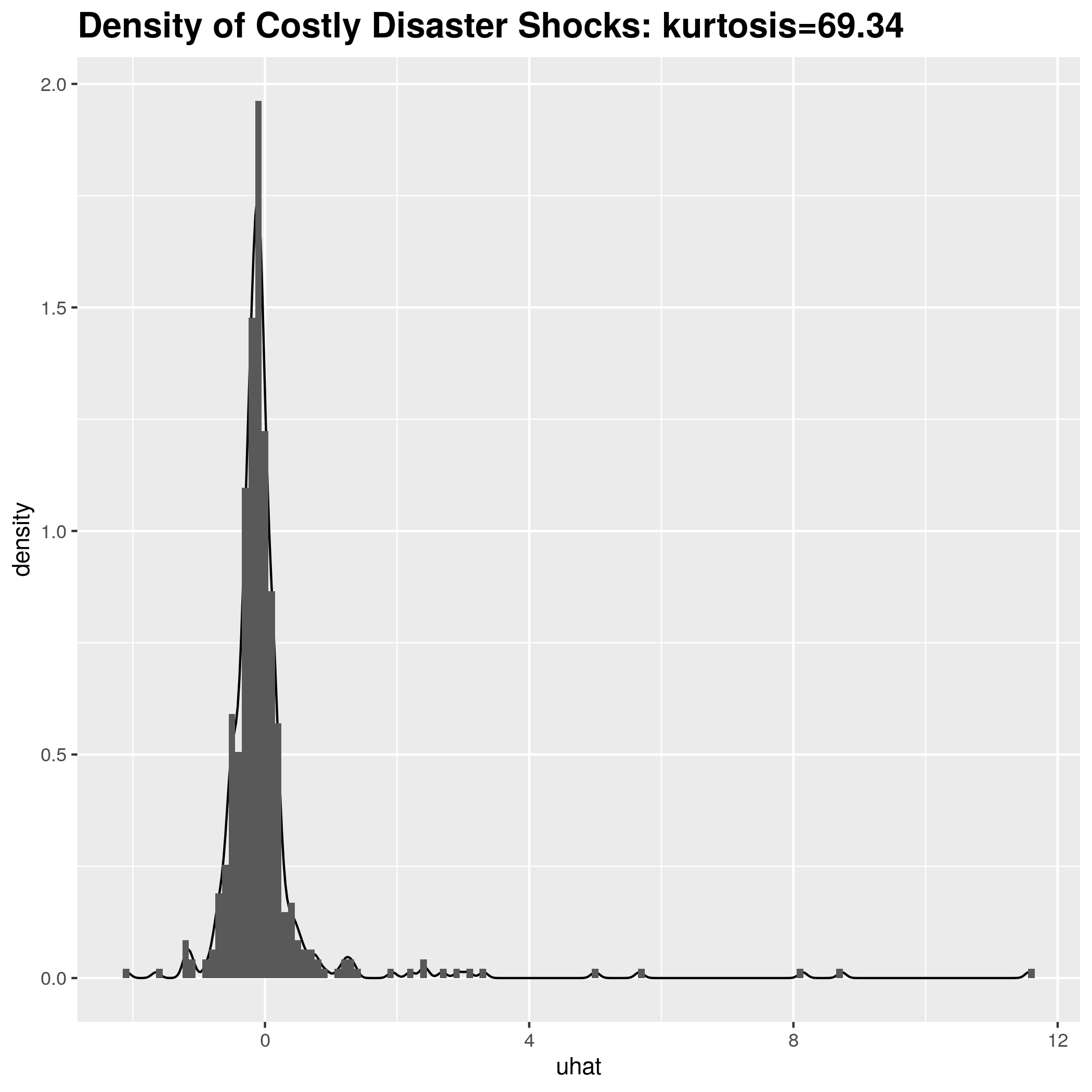
The paper provides three results for SVARs under the assumption that the primitive shocks are mutually independent. First, a framework is proposed to study the dynamic effects of disaster-type shocks with infinite variance. We show that the least squares estimates of the VAR are consistent but have non-standard properties. Second, it is shown that the restrictions imposed on a SVAR can be validated by testing independence of the identified shocks. The test can be applied whether the data have fat or thin tails, and to over as well as exactly identified models. Third, the disaster shock is identified as the component with the largest kurtosis, where the mutually independent components are estimated using an estimator that is valid even in the presence of an infinite variance shock. Two applications are considered. In the first, the independence test is used to shed light on the conflicting evidence regarding the role of uncertainty in economic fluctuations. In the second, disaster shocks are shown to have short term economic impact arising mostly from feedback dynamics.