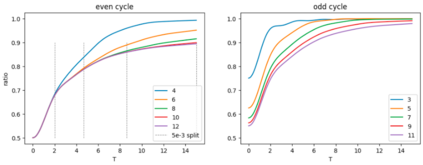
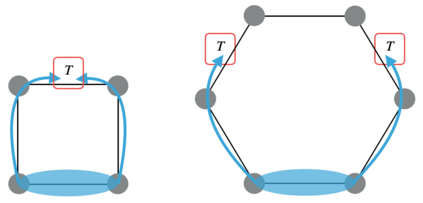
Quantum Annealing (QA) is a computational framework where a quantum system's continuous evolution is used to find the global minimum of an objective function over an unstructured search space. It can be seen as a general metaheuristic for optimization problems, including NP-hard ones if we allow an exponentially large running time. While QA is widely studied from a heuristic point of view, little is known about theoretical guarantees on the quality of the solutions obtained in polynomial time. In this paper we use a technique borrowed from theoretical physics, the Lieb-Robinson (LR) bound, and develop new tools proving that short, constant time quantum annealing guarantees constant factor approximations ratios for some optimization problems when restricted to bounded degree graphs. Informally, on bounded degree graphs the LR bound allows us to retrieve a (relaxed) locality argument, through which the approximation ratio can be deduced by studying subgraphs of bounded radius. We illustrate our tools on problems MaxCut and Maximum Independent Set for cubic graphs, providing explicit approximation ratios and the runtimes needed to obtain them. Our results are of similar flavor to the well-known ones obtained in the different but related QAOA (quantum optimization algorithms) framework. Eventually, we discuss theoretical and experimental arguments for further improvements.
翻译:QA 是一个计算框架, 量子系统的持续进化用于在无结构的搜索空间中找到全球最低目标功能。 它可以被视为优化问题的一般计量经济学, 包括当我们允许极大运行时间时, 包括NP- 硬问题。 虽然QA是从超速角度广泛研究的, 却很少知道对多边时间所获取解决方案质量的理论保障。 在本文中, 我们使用从理论物理学中借用的技术, Lieb- Robinson (LR) 捆绑, 并开发新的工具, 证明时间不变的量子保证某些优化问题在限制为约束度图时, 包括NP- 硬问题 。 非正式地, 在约束度图上, LA 将使我们能够检索一个( 松散的) 地点参数, 通过研究受约束的半径的子图可以推断出近似比率。 我们用“ MaxCut ” 和“ 最大独立 ” 工具来绘制立方图, 提供明确的精确的精确度比对准率比率, 和运行时间 QA 相关实验性框架 。 我们获得的结果与“ ” 相似, 与最终的逻辑框架相似。