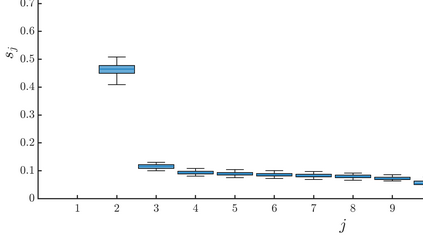
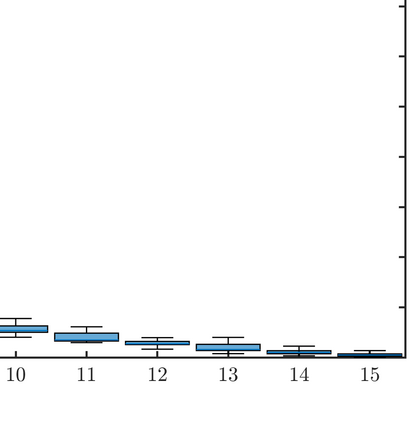
This paper deals with the dynamic factor analysis problem for an ARMA process. To robustly estimate the number of factors, we construct a confidence region centered in a finite sample estimate of the underlying model which contains the true model with a prescribed probability. In this confidence region, the problem, formulated as a rank minimization of a suitable spectral density, is efficiently approximated via a trace norm convex relaxation. The latter is addressed by resorting to the Lagrange duality theory, which allows to prove the existence of solutions. Finally, a numerical algorithm to solve the dual problem is presented. The effectiveness of the proposed estimator is assessed through simulation studies both with synthetic and real data.
翻译:本文论述ARMA进程动态要素分析问题。 为了精确估计因素的数量, 我们构建了一个信任区, 其核心是对包含真实模型且具有一定概率的概率的模型基础模型进行有限的抽样估计。 在这个信任区, 这个问题是作为适当光谱密度的排位最小化, 是通过微量规范二次曲线的放松来有效估计的。 后一种问题通过使用拉格朗双元理论来解决, 该理论可以证明存在解决办法。 最后, 提出了解决双重问题的数字算法。 拟议的估计器的有效性通过合成数据和真实数据的模拟研究来评估 。