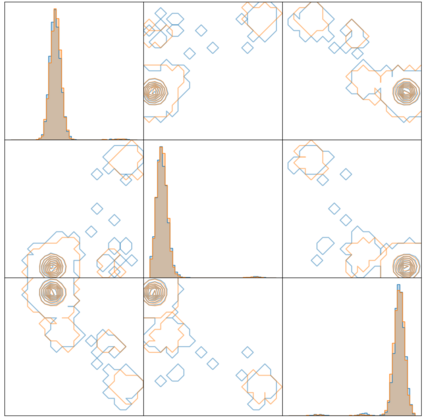
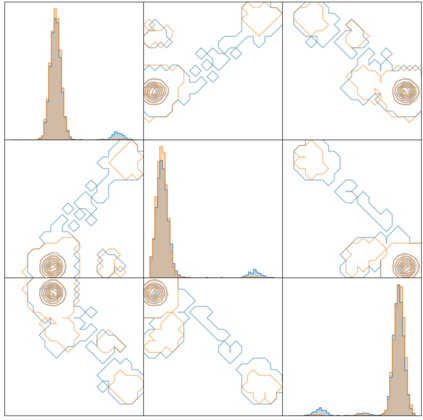
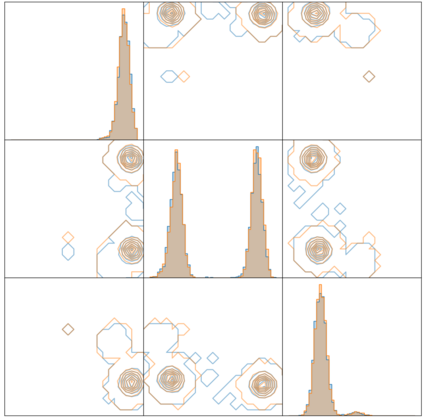
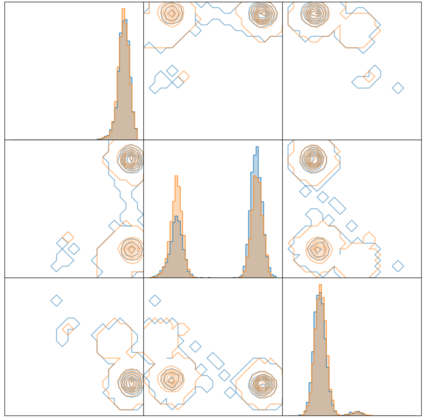
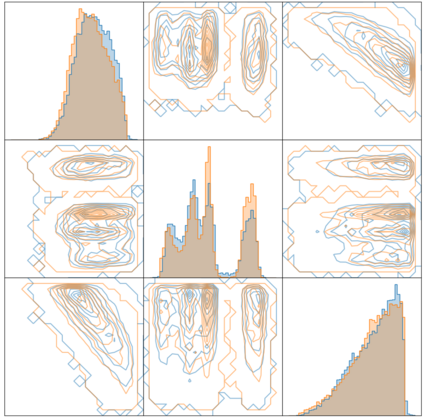
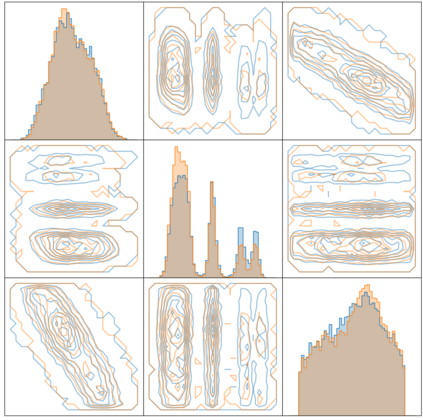
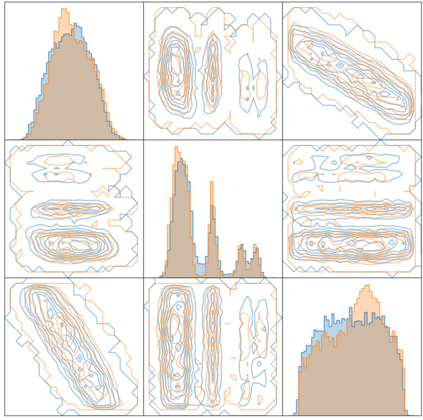
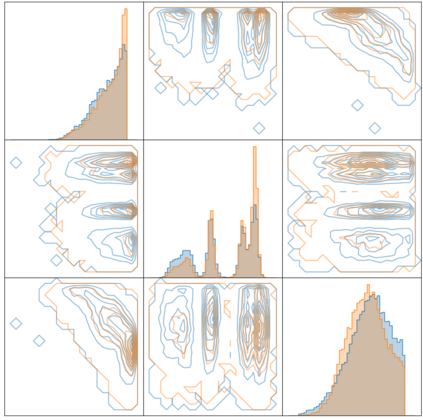
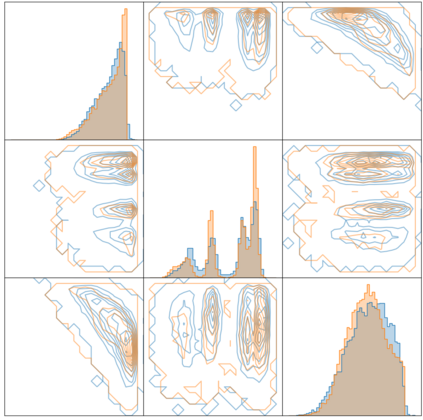
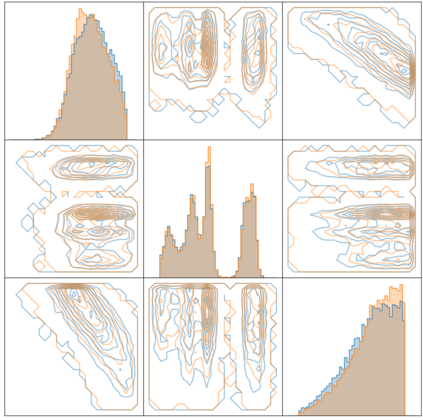
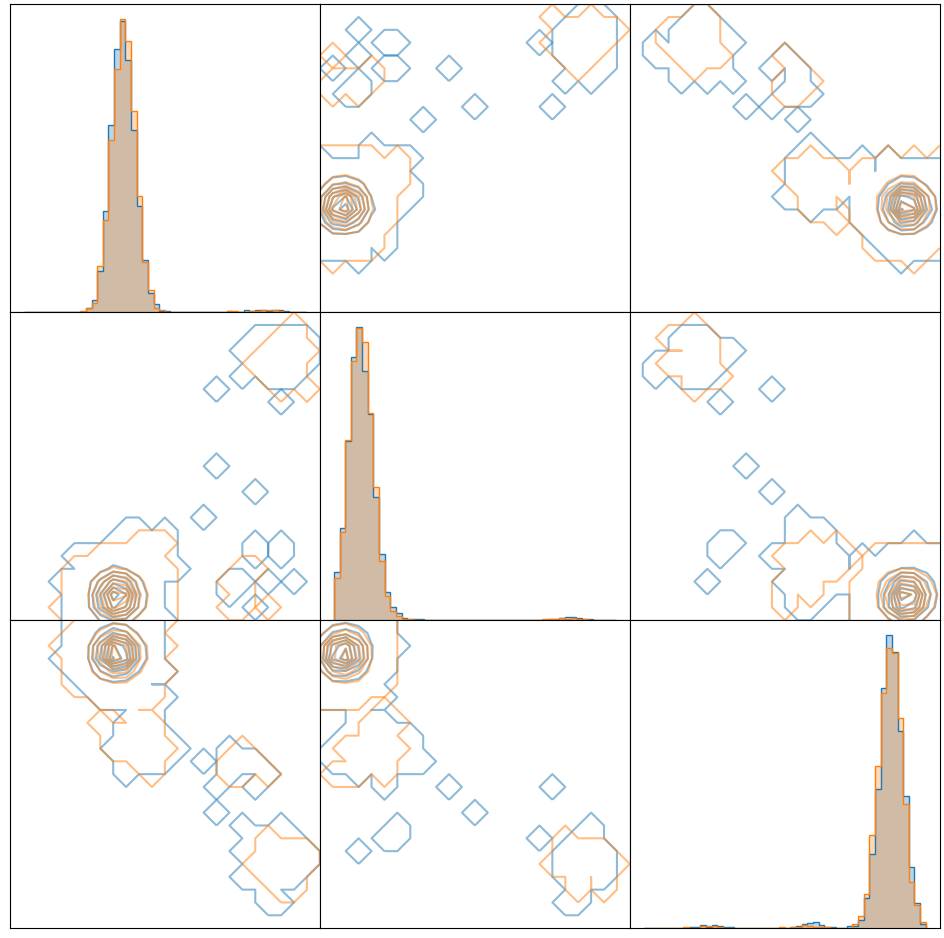
To overcome topological constraints and improve the expressiveness of normalizing flow architectures, Wu, K\"ohler and No\'e introduced stochastic normalizing flows which combine deterministic, learnable flow transformations with stochastic sampling methods. In this paper, we consider stochastic normalizing flows from a Markov chain point of view. In particular, we replace transition densities by general Markov kernels and establish proofs via Radon-Nikodym derivatives which allows to incorporate distributions without densities in a sound way. Further, we generalize the results for sampling from posterior distributions as required in inverse problems. The performance of the proposed conditional stochastic normalizing flow is demonstrated by numerical examples.
翻译:为了克服地形限制并改善正常流体结构的表达性,Wu, K\'ohler and No\e引入了将确定性、可学习性流变与随机采样方法相结合的随机性流流的正常化流动。在本文中,我们考虑从Markov 链点的角度对随机性流进行正常化。特别是,我们用一般的Markov内核取代过渡性密度,并通过Radon-Nikodym衍生物建立证据,从而能够以合理的方式将无密度的分布纳入其中。此外,我们按需要将后方分布的采样结果概括为反面问题。提议的有条件的随机性正常化流动的绩效通过数字实例来证明。