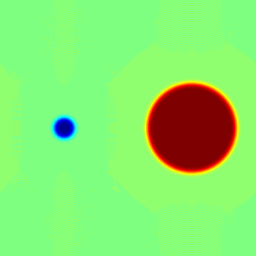
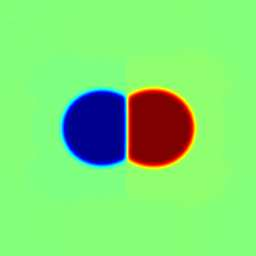
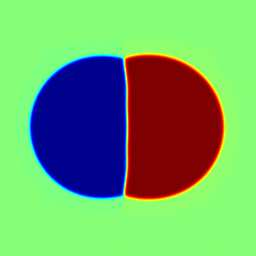
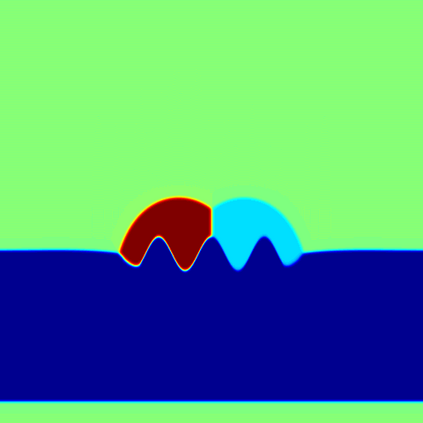
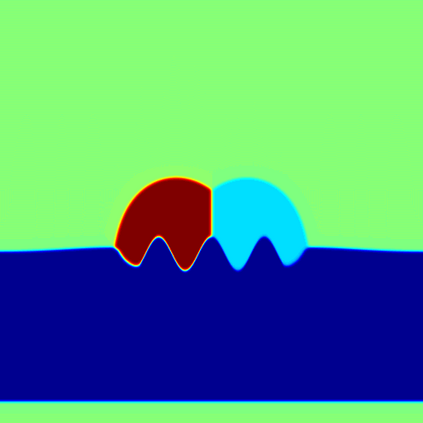
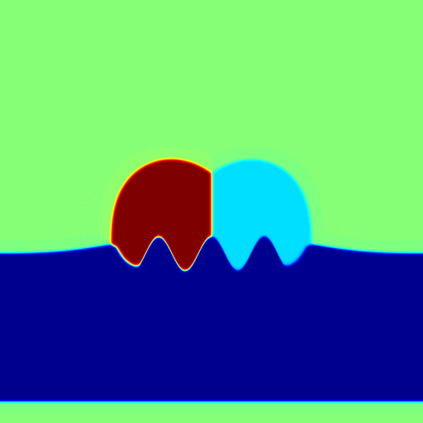
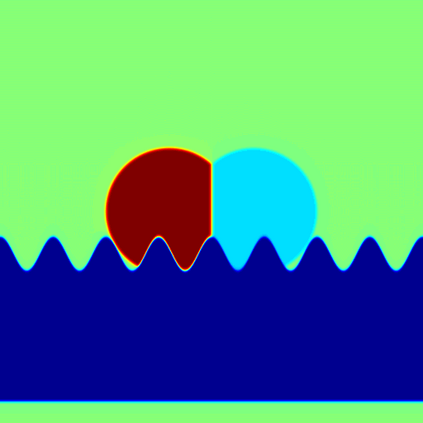
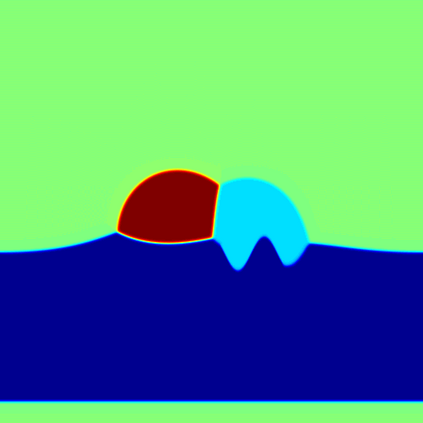
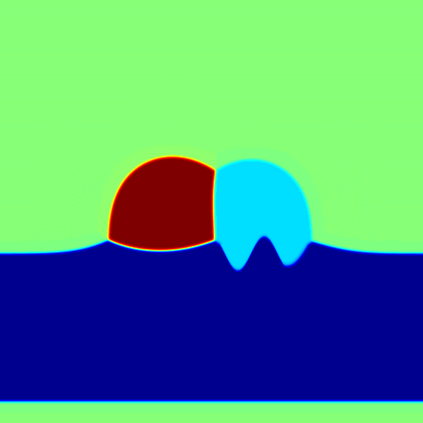
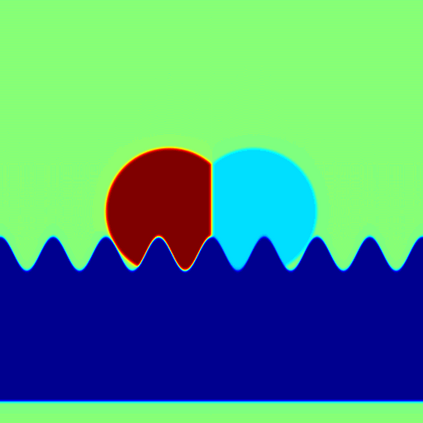
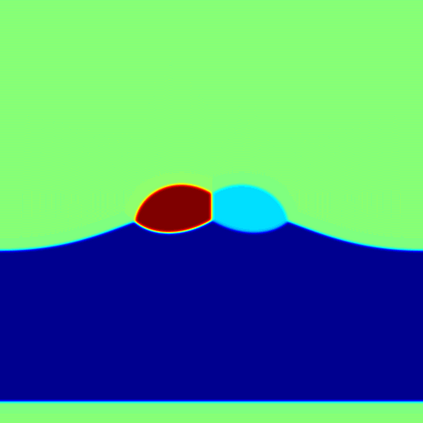
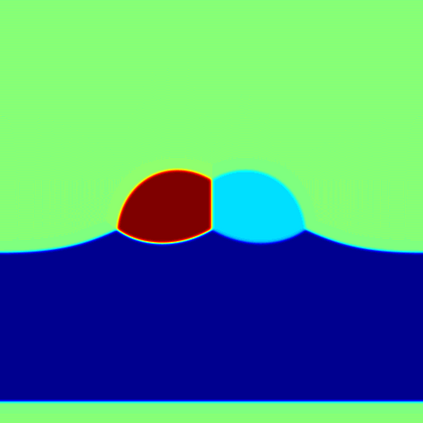
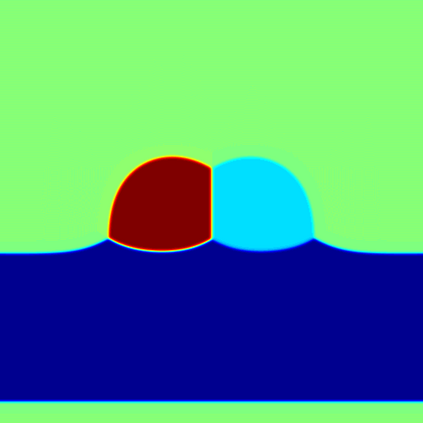
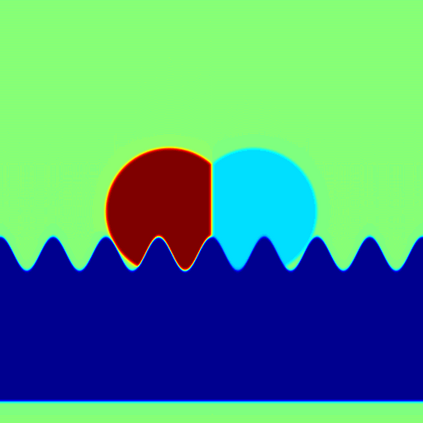
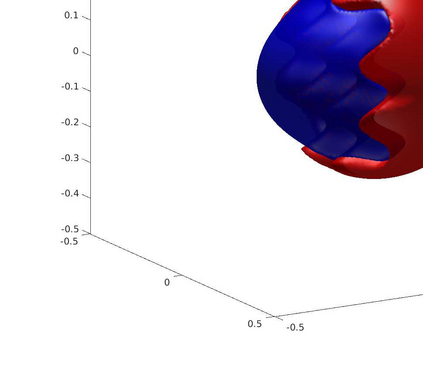
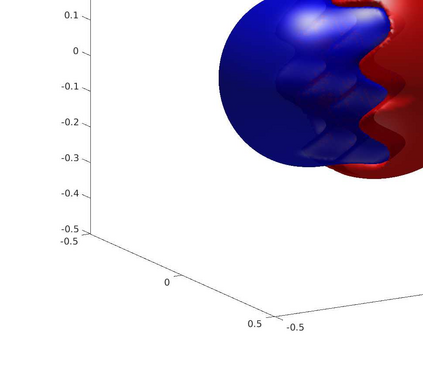
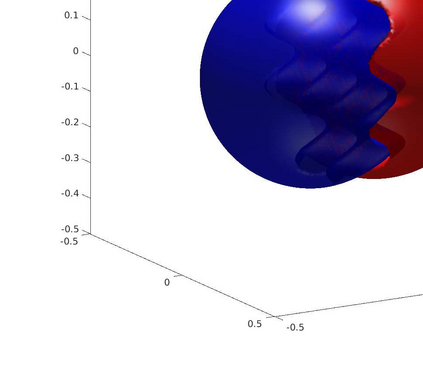
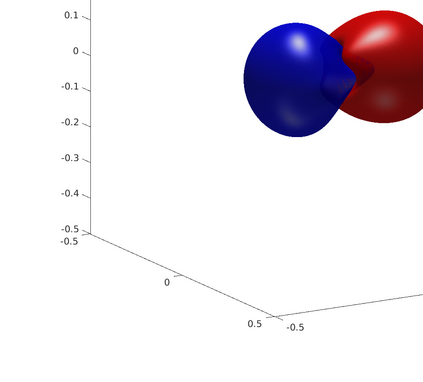
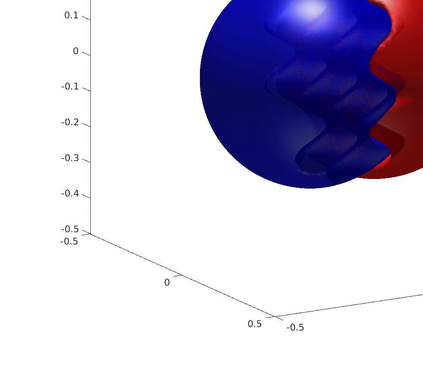
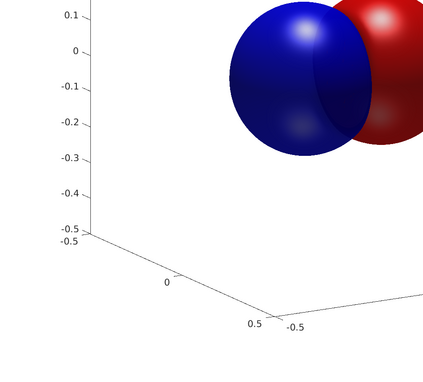
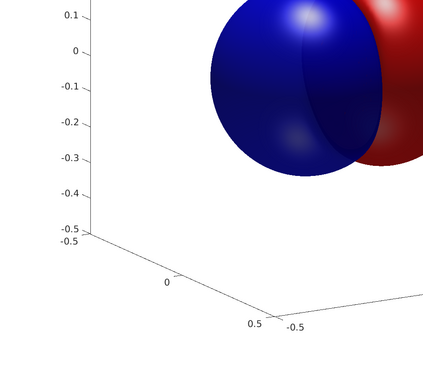
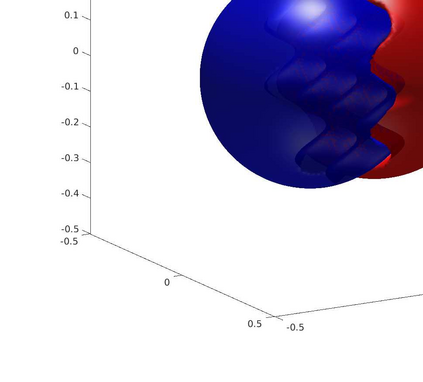
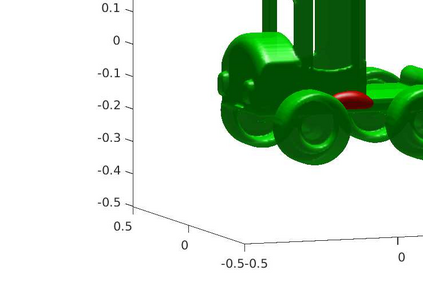
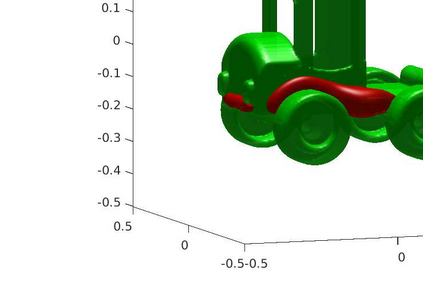
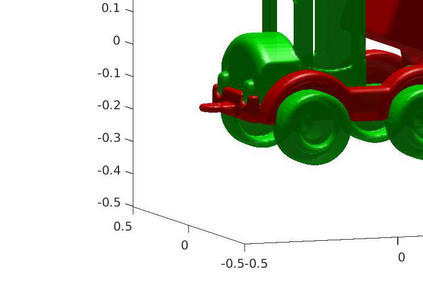
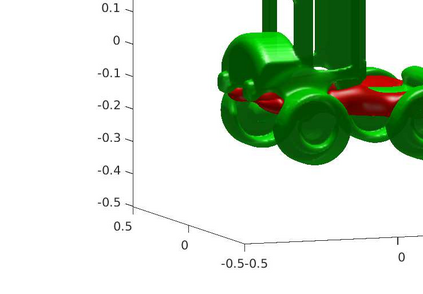
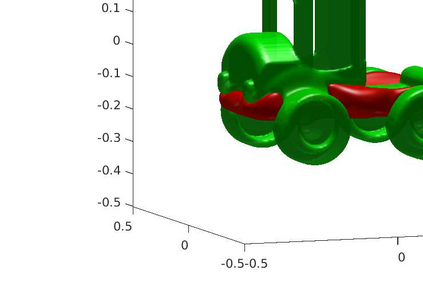
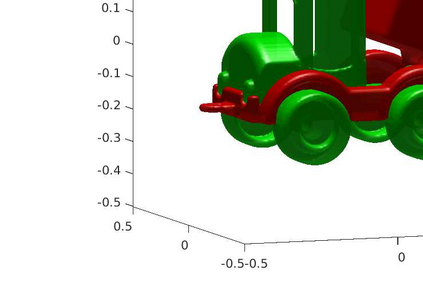
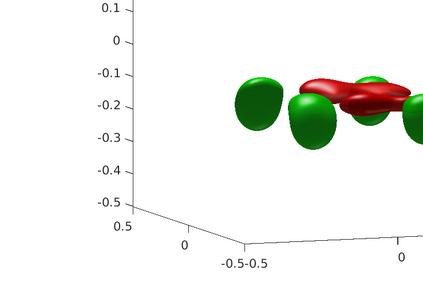
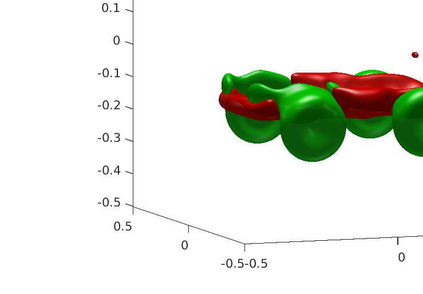
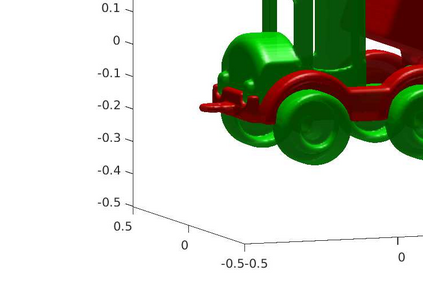
This paper is devoted to the robust approximation with a variational phase field approach of multiphase mean curvature flows with possibly highly contrasted mobilities. The case of harmonically additive mobilities has been addressed recently using a suitable metric to define the gradient flow of the phase field approximate energy. We generalize this approach to arbitrary nonnegative mobilities using a decomposition as sums of harmonically additive mobilities. We establish the consistency of the resulting method by analyzing the sharp interface limit of the flow: a formal expansion of the phase field shows that the method is of second order. We propose a simple numerical scheme to approximate the solutions to our new model. Finally, we present some numerical experiments in dimensions 2 and 3 that illustrate the interest and effectiveness of our approach, in particular for approximating flows in which the mobility of some phases is zero.
翻译:本文专门论述以多相平均曲线流的变相阶段实地方法对多相平均曲线流进行稳健近似近似,并可能存在高度对比的动态。关于协调性添加性动员的案例,最近用一个适当的指标来界定相干场能量的梯度流。我们用分解作为协调性添加性动员的总和,将这一方法推广到任意性非阴性动员。我们通过分析流动的尖锐界面界限,确定由此产生的方法的一致性:阶段字段的正式扩展表明该方法为第二顺序。我们提出了一个简单的数字方案,将解决方案与我们的新模型相近。最后,我们介绍了一些规模2和3的数字实验,说明我们方法的兴趣和有效性,特别是对于一些阶段的流动性为零的相近流动。