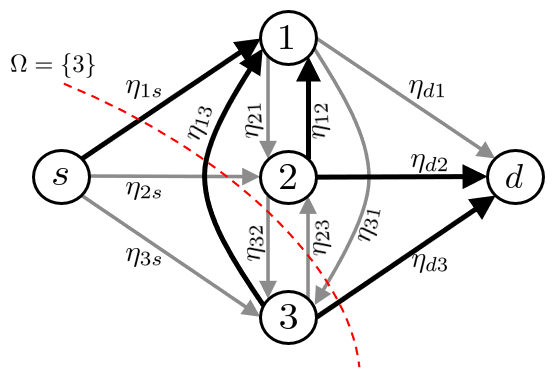
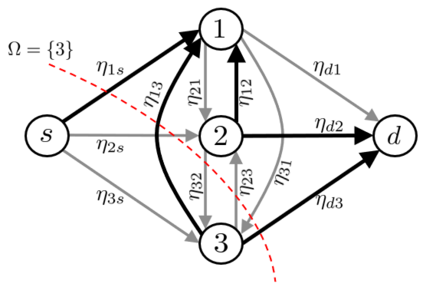
This paper considers a diamond network with $n$ interconnected relays, namely a network where a source communicates with a destination by hopping information through $n$ communicating/interconnected relays. Specifically, the main focus of the paper is on characterizing sufficient conditions under which the $n+1$ states (out of the $2^{n}$ possible ones) in which at most one relay is transmitting suffice to characterize the approximate capacity, that is the Shannon capacity up to an additive gap that only depends on $n$. Furthermore, under these sufficient conditions, closed form expressions for the approximate capacity and scheduling (that is, the fraction of time each relay should receive and transmit) are provided. A similar result is presented for the dual case, where in each state at most one relay is in receive mode.
翻译:本文考虑的是具有美元互联式继电器的钻石网络,即一个来源通过通信/互连式继电器与目的地通信的网络,具体而言,本文件的主要重点是说明n+1美元状态(可能为2 ⁇ n}$)足以说明大致容量,即香农容量达到仅取决于美元的额外差距的钻石网络。此外,根据这些足够条件,为大致容量和时间安排(即每个继电器应接收和传输的时间的一小部分)提供了封闭式表达方式。对于双重情况,也提出了类似的结果,即在大多数州,每个中继器都处于接收模式。