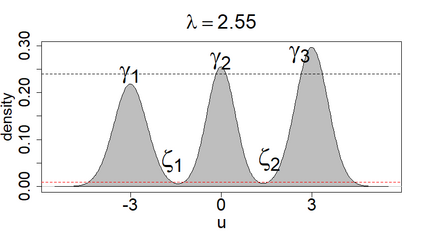
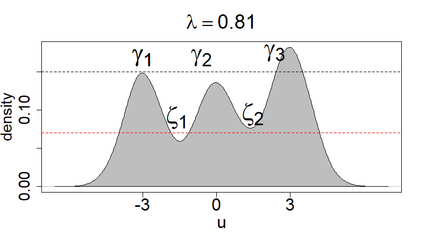
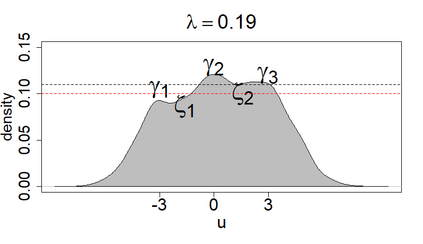
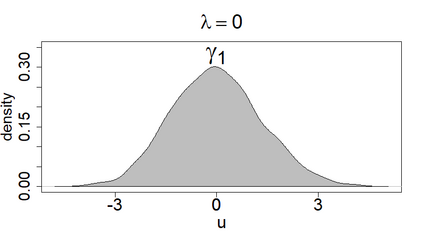
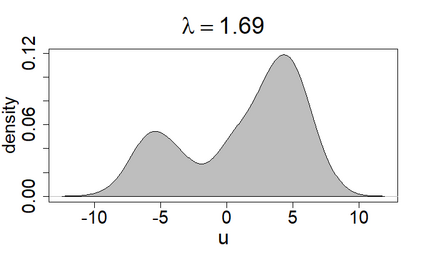
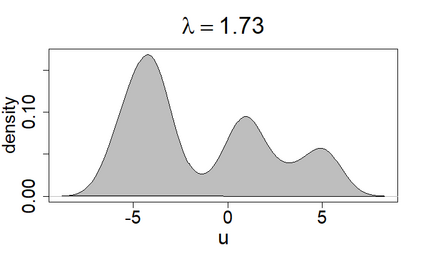
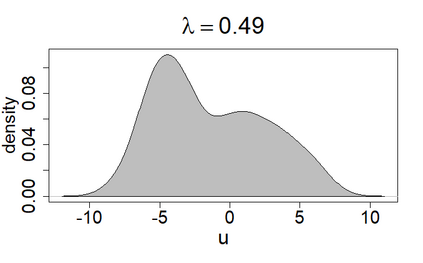
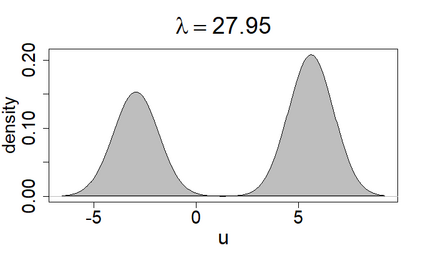
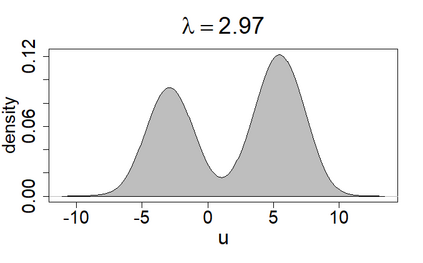
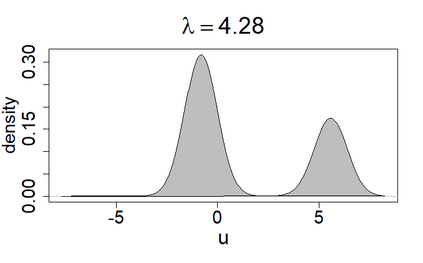
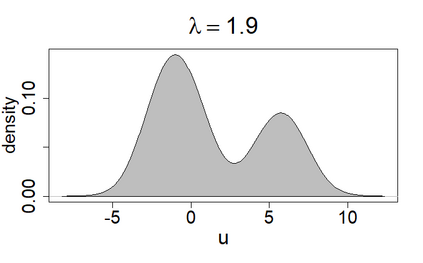
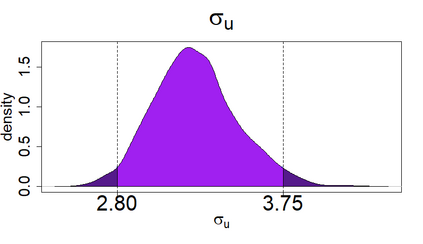
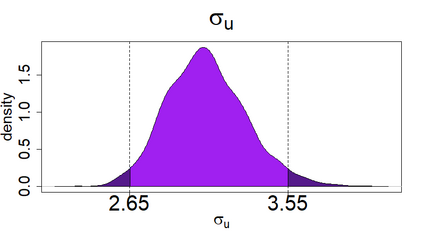
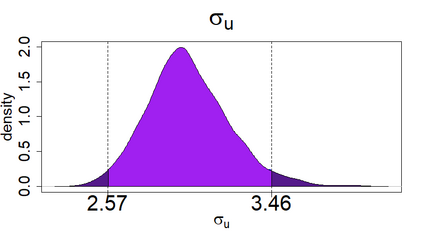
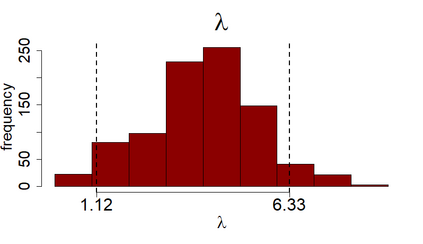
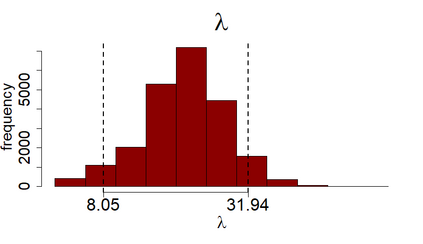
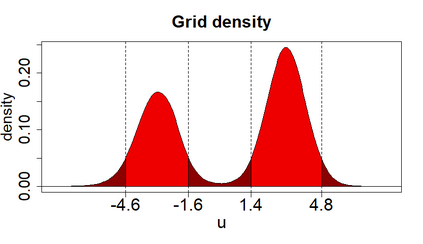
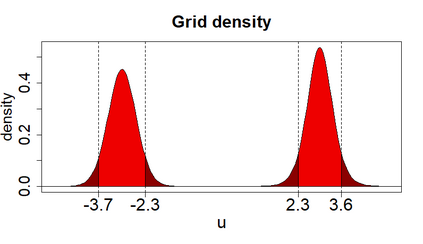
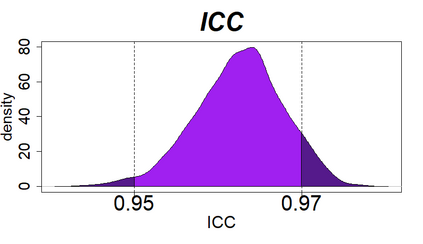
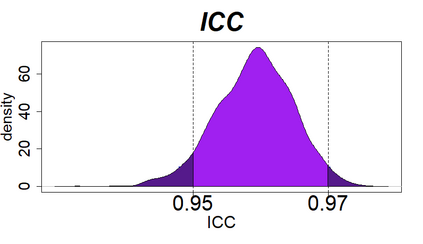
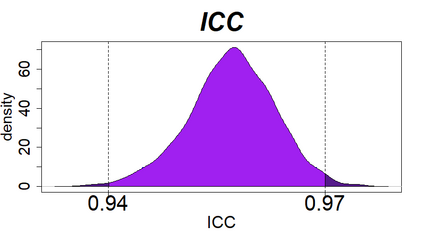
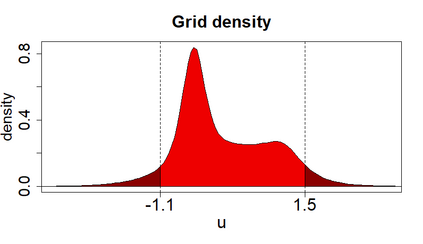
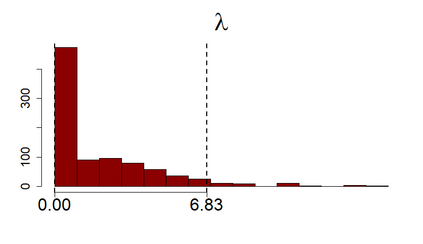
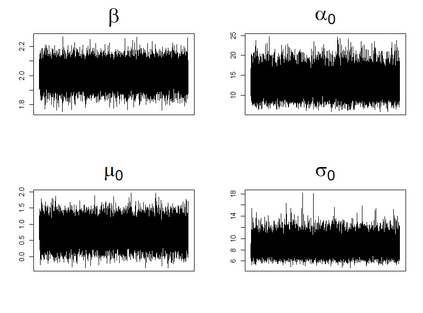
In several observational contexts where different raters evaluate a set of items, it is common to assume that all raters draw their scores from the same underlying distribution. However, a plenty of scientific works have evidenced the relevance of individual variability in different type of rating tasks. To address this issue the intra-class correlation coefficient (ICC) has been used as a measure of variability among raters within the Hierarchical Linear Models approach. A common distributional assumption in this setting is to specify hierarchical effects as independent and identically distributed from a normal with the mean parameter fixed to zero and unknown variance. The present work aims to overcome this strong assumption in the inter-rater agreement estimation by placing a Dirichlet Process Mixture over the hierarchical effects' prior distribution. A new nonparametric index $\lambda$ is proposed to quantify raters polarization in presence of group heterogeneity. The model is applied on a set of simulated experiments and real world data. Possible future directions are discussed.
翻译:暂无翻译