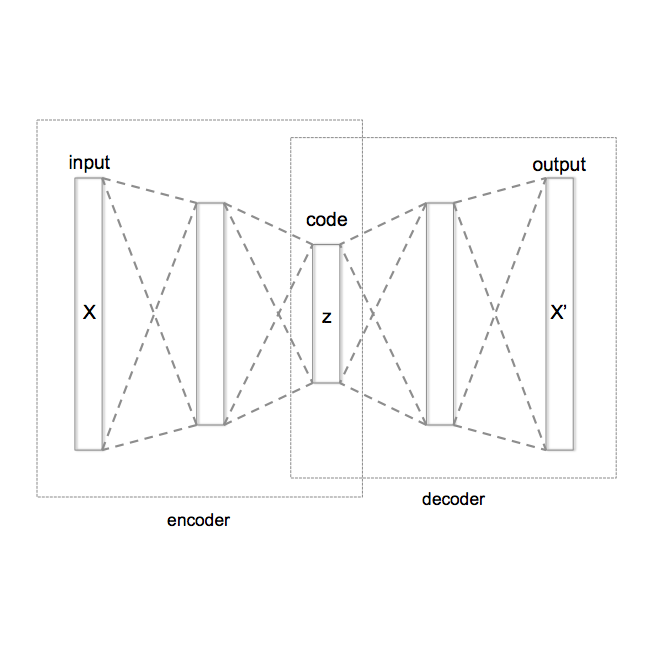
Despite its wide use and empirical successes, the theoretical understanding and study of the behaviour and performance of the variational autoencoder (VAE) have only emerged in the past few years. We contribute to this recent line of work by analysing the VAE's reconstruction ability for unseen test data, leveraging arguments from the PAC-Bayes theory. We provide generalisation bounds on the theoretical reconstruction error, and provide insights on the regularisation effect of VAE objectives. We illustrate our theoretical results with supporting experiments on classical benchmark datasets.
翻译:尽管对变式自动编码器(VAE)的使用广泛,也取得了经验性的成功,但是对变式自动编码器(VAE)行为和表现的理论理解和研究只是在过去几年才出现。我们通过分析VAE对无形测试数据的重建能力,利用PAC-Bayes理论的论据,为最近的工作作出了贡献。我们提供了理论重建错误的概括性界限,并提供了对VAE目标的规范化效果的洞察力。我们用经典基准数据集方面的支持性实验来说明我们的理论结果。