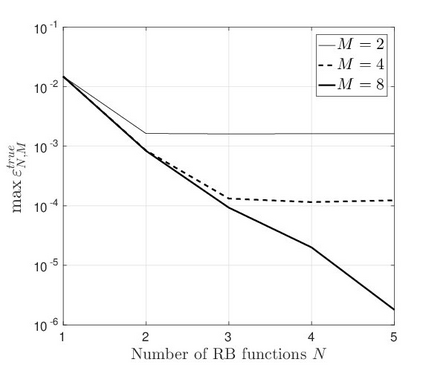
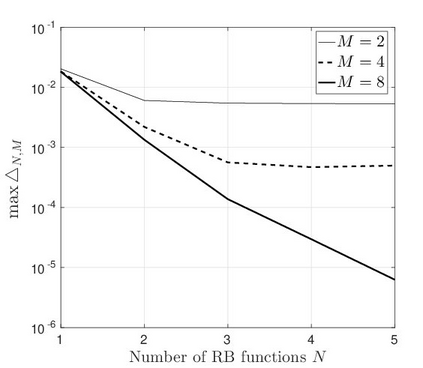
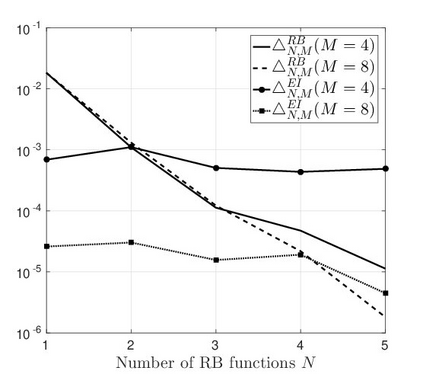
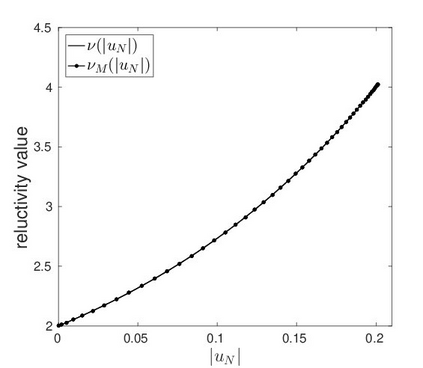
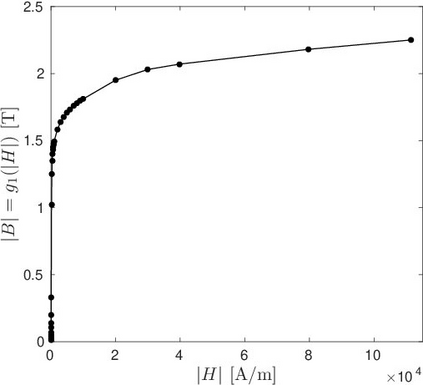
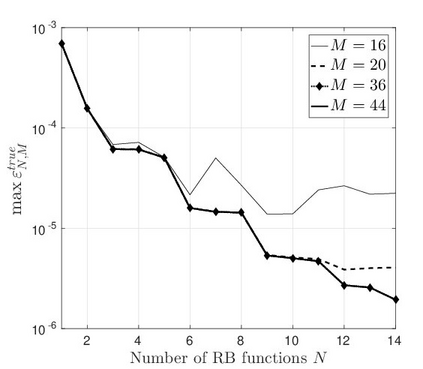
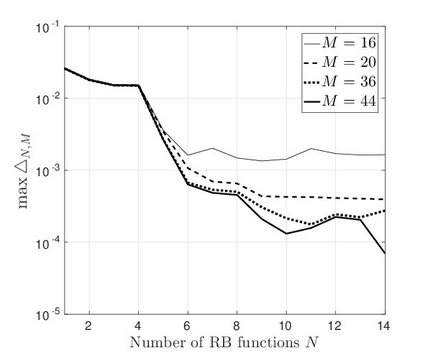
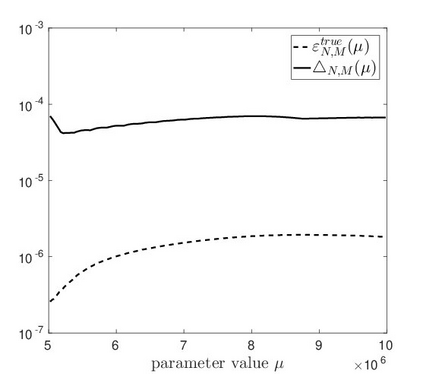
In this paper, we propose a certified reduced basis (RB) method for quasilinear parabolic problems. The method is based on a space-time variational formulation. We provide a residual-based a-posteriori error bound on a space-time level and the corresponding efficiently computable estimator for the certification of the method. We use the Empirical Interpolation method (EIM) to guarantee the efficient offline-online computational procedure. The error of the EIM method is then rigorously incorporated into the certification procedure. The Petrov-Galerkin finite element discretization allows to benefit from the Crank-Nicolson interpretation of the discrete problem and to use a POD-Greedy approach to construct the reduced-basis spaces of small dimensions. It computes the reduced basis solution in a time-marching framework while the RB approximation error in a space-time norm is controlled by the estimator. Therefore the proposed method incorporates a POD-Greedy approximation into a space-time certification.
翻译:在本文中,我们建议对准线性抛物线问题采用经认证的减少基准(RB)方法,该方法以空间-时间变异配方为基础,提供基于空间-时间水平的剩余偏差,并用相应的高效可比较的估测器来验证该方法。我们使用“经验性内插法”来保证高效的离线离线计算程序。然后,EIM方法的错误被严格地纳入认证程序。Petrov-Galerkin 有限元素分解使离散能够受益于对离散问题的Crank-Nicolson解释,并使用POD-Greedy 方法来构建小维度的缩小的空格空间。它用时间框架来计算减少的基础解决方案,而空间-时间规范的RB近差则由估测器控制。因此,拟议方法将POD-Greedy近似值纳入空间-时间验证。