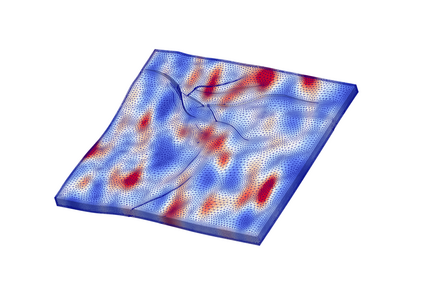
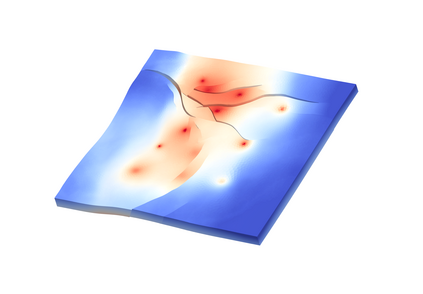
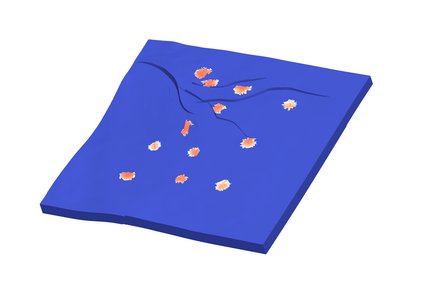
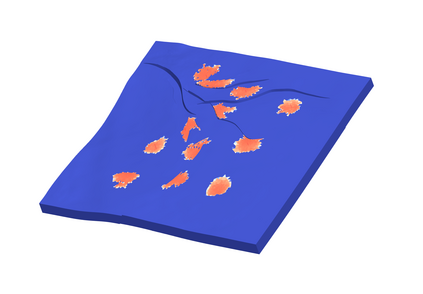
Many subsurface engineering applications involve tight-coupling between fluid flow, solid deformation, fracturing, and similar processes. To better understand the complex interplay of different governing equations, and therefore design efficient and safe operations, numerical simulations are widely used. Given the relatively long time-scales of interest, fully-implicit time-stepping schemes are often necessary to avoid time-step stability restrictions. A major computational bottleneck for these methods, however, is the linear solver. These systems are extremely large and ill-conditioned. Because of the wide range of processes and couplings that may be involved--e.g. formation and propagation of fractures, deformation of the solid porous medium, viscous flow of one or more fluids in the pores and fractures, complicated well sources and sinks, etc.--it is difficult to develop general-purpose but scalable linear solver frameworks. This challenge is further aggravated by the range of different discretization schemes that may be adopted, which have a direct impact on the linear system structure. To address this obstacle, we describe a flexible framework based on multigrid reduction that can produce purely algebraic preconditioners for a wide spectrum of relevant physics and discretizations. We demonstrate its broad applicability by constructing scalable preconditioners for several problems, notably: a hybrid discretization of single-phase flow, compositional multiphase flow with complex wells, and hydraulic fracturing simulations. Extension to other systems can be handled quite naturally. We demonstrate the efficiency and scalability of the resulting solvers through numerical examples of difficult, field-scale problems.
翻译:许多地表以下工程应用涉及流体流、固态变形、分解和类似过程之间的紧密结合。为了更好地了解不同治理方程的复杂相互作用,从而设计高效和安全的操作,广泛使用数字模拟。鉴于兴趣的时间尺度相对较长,往往需要完全隐含的时间跨度计划来避免时间步稳定限制。这些方法的主要计算瓶颈是线性求解器。这些系统非常庞大,条件极差。由于可能涉及的多种进程和联结,例如断裂的形成和传播、坚固的松散介介质的变形、一个或一个以上流体在孔和断裂中的粘性流动、复杂的源和汇等等。——很难制定通用但可缩放的线性求解框架。由于采用不同的离分解机制,这可能会对线性系统结构产生直接的影响。为了解决这一障碍,我们描述一个灵活的框架,其基础是多层次流的介质介质介质,其直径流的内流流和直径直径直径直流性,我们描述一个基于多层次流流流流流流流的灵活框架,从而展示了多频度的多层次的直径性前提。我们可以展示了多层次物理学的多层次流流流流流的多层次的多层次结构。