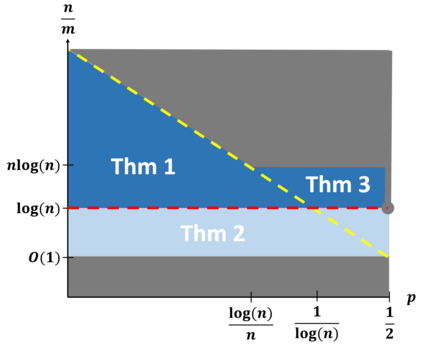
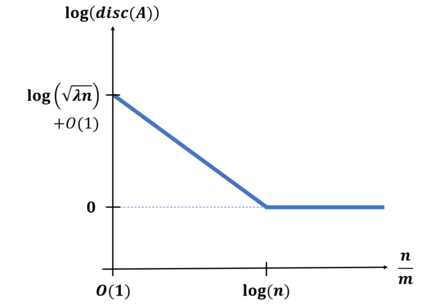
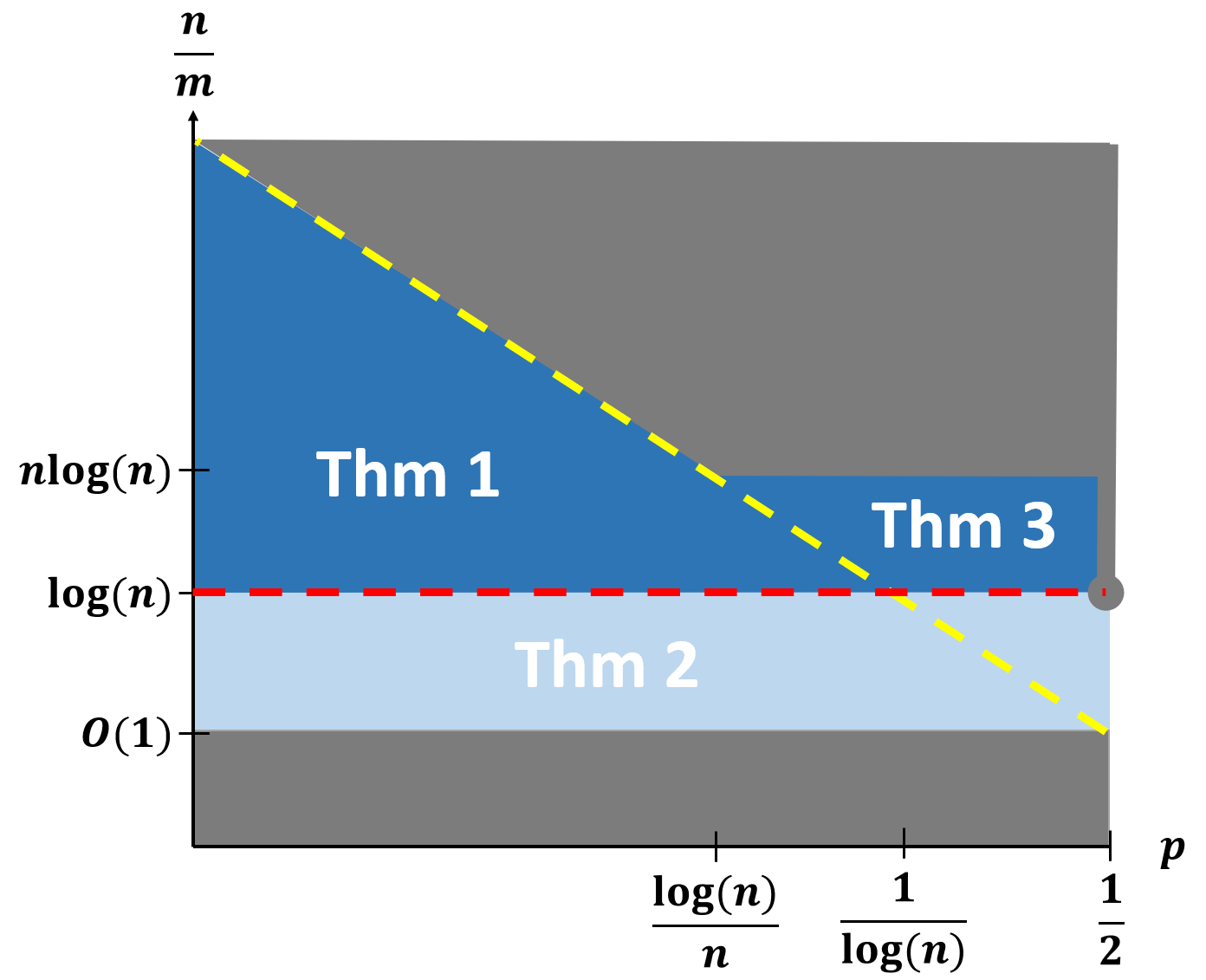
A recent approach to the Beck-Fiala conjecture, a fundamental problem in combinatorics, has been to understand when random integer matrices have constant discrepancy. We give a complete answer to this question for two natural models: matrices with Bernoulli or Poisson entries. For Poisson matrices, we further characterize the discrepancy for any rectangular aspect ratio. These results give sharp answers to questions of Hoberg and Rothvoss (SODA 2019) and Franks and Saks (Random Structures Algorithms 2020). Our main tool is a conditional second moment method combined with Stein's method of exchangeable pairs. While previous approaches are limited to dense matrices, our techniques allow us to work with matrices of all densities. This may be of independent interest for other sparse random constraint satisfaction problems.
翻译:最近对Beck-Fiala的预测方法,即组合学的一个根本问题,是了解随机整数矩阵是否始终存在差异。我们对两种自然模型的问题给出了完整的答案:Bernoulli 或 Poisson 条目的矩阵。Poisson 矩阵,我们进一步辨别了任何矩形方位比例的差异。这些结果对Hoberg和Rothvos(SODA 2019)以及Franks和Saks(Random结构Algorithms 2020)的问题做出了清晰的回答。我们的主要工具是有条件的第二时刻方法,与Stein的可交换配对方法相结合。虽然以前的方法仅限于密集的矩阵,但我们的技术允许我们使用所有密度的矩阵。对于其他稀有的随机制约性满意度问题,这可能具有独立的兴趣。