A scattering transform for graphs based on heat semigroups, with an application for the detection of anomalies in positive time series with underlying periodicities

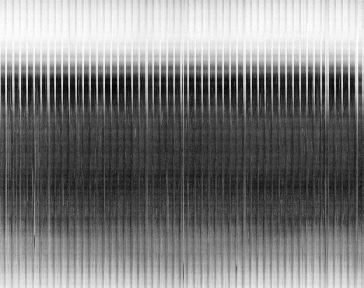
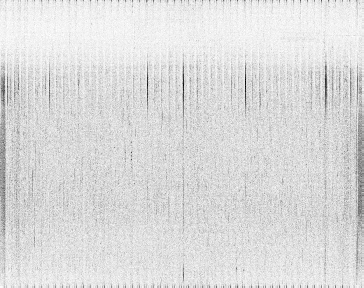
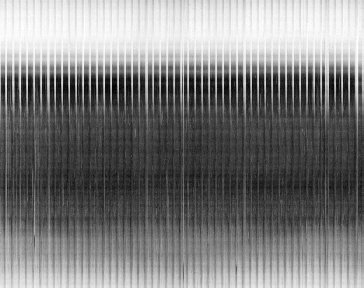
This paper develops an adaptive version of Mallat's scattering transform for signals on graphs. The main results are norm bounds for the layers of the transform, obtained from a version of a Beurling-Deny inequality that permits to remove the nonlinear steps in the scattering transform. Under statistical assumptions on the input signal, the norm bounds can be refined. The concepts presented here are illustrated with an application to traffic counts which exhibit characteristic daily and weekly periodicities. Anomalous traffic patterns which deviate from these expected periodicities produce a response in the scattering transform.