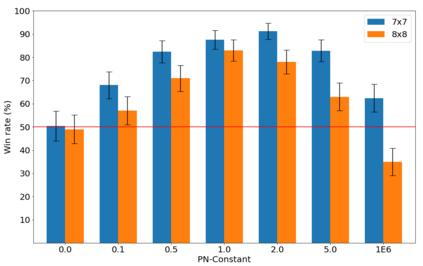
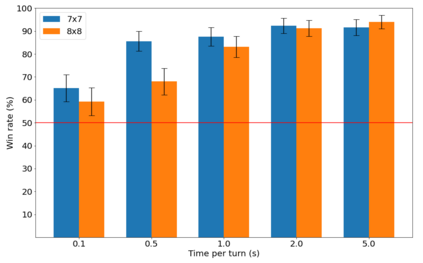
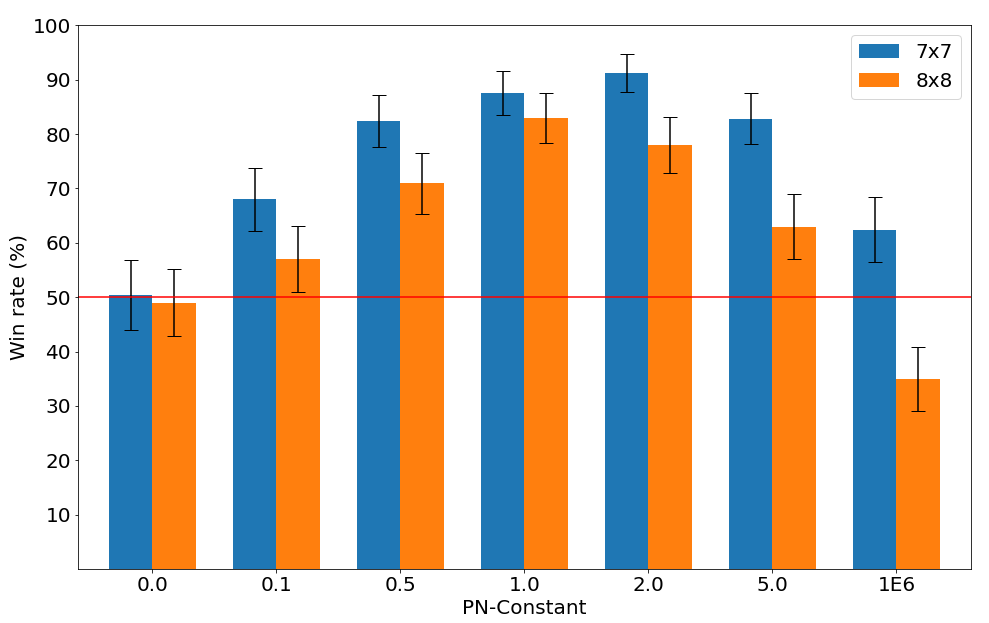
Proof-Number Search (PNS) and Monte-Carlo Tree Search (MCTS) have been successfully applied for decision making in a range of games. This paper proposes a new approach called PN-MCTS that combines these two tree-search methods by incorporating the concept of proof and disproof numbers into the UCT formula of MCTS. Experimental results demonstrate that PN-MCTS outperforms basic MCTS in several games including Lines of Action, MiniShogi, Knightthrough, and Awari, achieving win rates up to 94.0%.
翻译：在一系列游戏中,在决策中成功地应用了校对数搜索(PNS)和蒙特卡洛树搜索(MCTS),本文提出了一种名为PN-MCTS的新办法,将这两种树搜索方法结合起来,将校对和不贴切数字的概念纳入MCTS的UCT公式。实验结果显示,PN-MCTS在包括行动线、MiniShogi、Nightnough和Awari在内的若干游戏中,优于基本的MCTS, 达到了高达94.0%的赢率。
相关内容
Arxiv
0+阅读 · 2022年7月24日
Arxiv
0+阅读 · 2022年7月19日
相关主题
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2022年7月24日
Arxiv
0+阅读 · 2022年7月19日
微信扫码咨询专知VIP会员