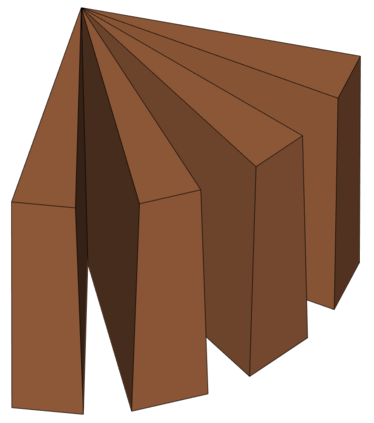
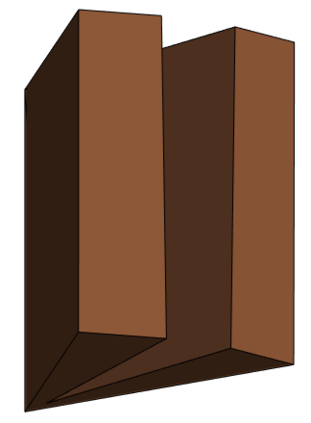
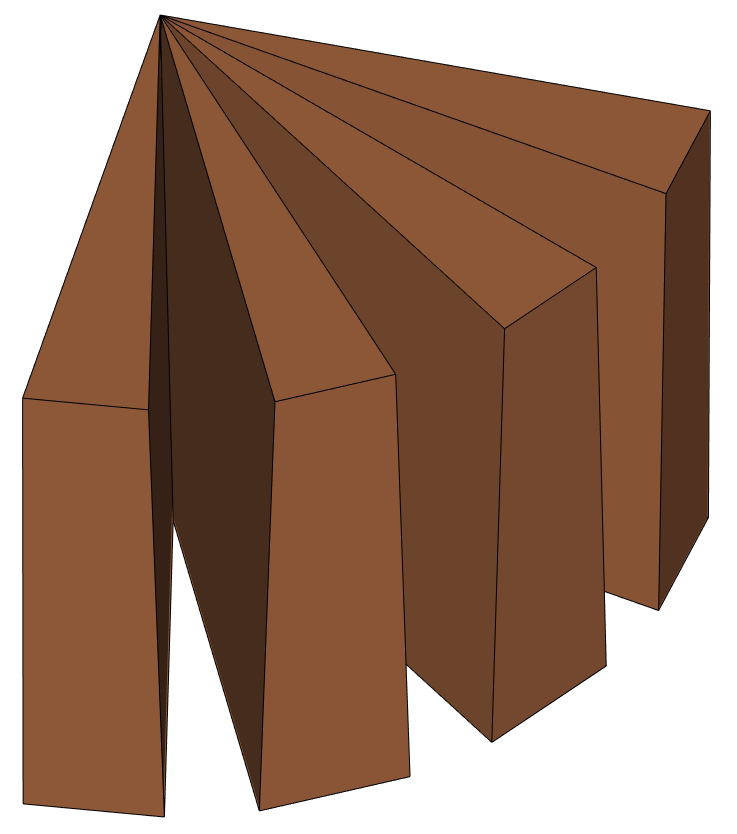
It is well known that, with a particular choice of norm, the classical double-layer potential operator $D$ has essential norm $<1/2$ as an operator on the natural trace space $H^{1/2}(\Gamma)$ whenever $\Gamma$ is the boundary of a bounded Lipschitz domain. This implies, for the standard second-kind boundary integral equations for the interior and exterior Dirichlet and Neumann problems in potential theory, convergence of the Galerkin method in $H^{1/2}(\Gamma)$ for any sequence of finite-dimensional subspaces $(\mathcal{H}_N)_{N=1}^\infty$ that is asymptotically dense in $H^{1/2}(\Gamma)$. Long-standing open questions are whether the essential norm is also $<1/2$ for $D$ as an operator on $L^2(\Gamma)$ for all Lipschitz $\Gamma$ in 2-d; or whether, for all Lipschitz $\Gamma$ in 2-d and 3-d, or at least for the smaller class of Lipschitz polyhedra in 3-d, the weaker condition holds that the operators $\pm \frac{1}{2}I+D$ are compact perturbations of coercive operators -- this a necessary and sufficient condition for the convergence of the Galerkin method for every sequence of subspaces $(\mathcal{H}_N)_{N=1}^\infty$ that is asymptotically dense in $L^2(\Gamma)$. We settle these open questions negatively. We give examples of 2-d and 3-d Lipschitz domains with Lipschitz constant equal to one for which the essential norm of $D$ is $\geq 1/2$, and examples with Lipschitz constant two for which the operators $\pm \frac{1}{2}I +D$ are not coercive plus compact. We also give, for every $C>0$, examples of Lipschitz polyhedra for which the essential norm is $\geq C$ and for which $\lambda I+D$ is not a compact perturbation of a coercive operator for any real or complex $\lambda$ with $|\lambda|\leq C$. Finally, we resolve negatively a related open question in the convergence theory for collocation methods.
翻译:众所周知, 典型的双层潜在操作员$D, 任何一系列的有限维基次空间 $( mathcal) $(D$), 而不是美元(H%1/2}) (Gamma) 基本规范 $(Gamma) 美元, 只要$(Gamma) 是受约束的 Lipschitz 域域的边界。 这意味着, 在潜在理论中, 普通的内外部Drichlet 和 Nemann 标准二种边界组合方程式, 普通的 Galkin 方法在 $H%1/2} (Gamma) 美元(Gamma) 美元(Gamma) 美元(Gamma) 美元($(D$) 美元(D$) 美元(D$(D) 美元), 普通的2美元(Hlickr2) 美元(Orickrickrickricker), 固定的Gal- 美元(D) 美元(美元) 美元(美元) 美元(美元) 美元(美元) 美元(美元) 美元(美元) 美元) 基的操作员的根基的根基的根(O) 或基的基的根(L) 的根(美元) 基的根) 的根(美元) 基的基的基) 的基) 的基的根基的根基的根基的根基的根基的根基的基的基的基的根基的根基的基的基的根基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的根) 的根基的基的基的基的基的基的基的基的基的基的基的基的基的基的根基的基的基的基的基的根基的根基的基的基的根基的基的基的根基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的基的根基的基的基的基的基的基的基的基的基的