Splitting schemes for FitzHugh--Nagumo stochastic partial differential equations

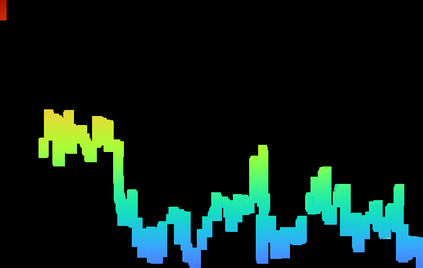
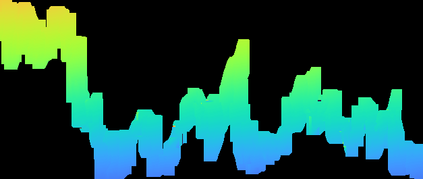
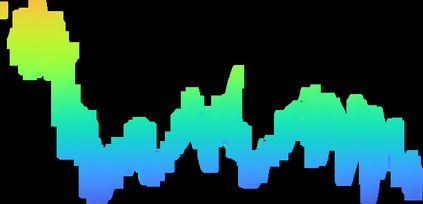
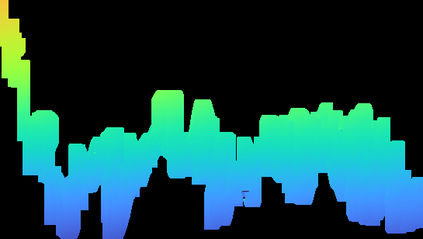
We design and study splitting integrators for the temporal discretization of the stochastic FitzHugh--Nagumo system. This system is a model for signal propagation in nerve cells where the voltage variable is solution of a one-dimensional parabolic PDE with a cubic nonlinearity driven by additive space-time white noise. We first show that the numerical solutions have finite moments. We then prove that the splitting schemes have, at least, the strong rate of convergence $1/4$. Finally, numerical experiments illustrating the performance of the splitting schemes are provided.