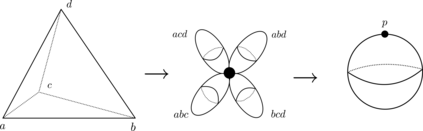
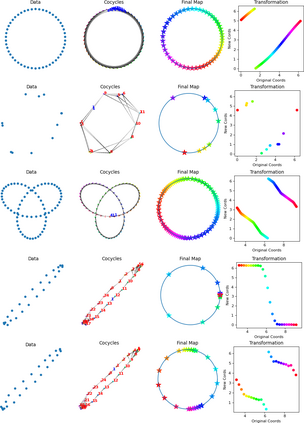
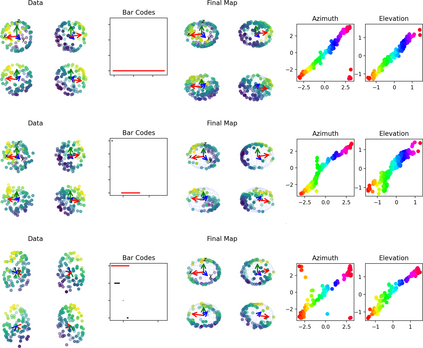
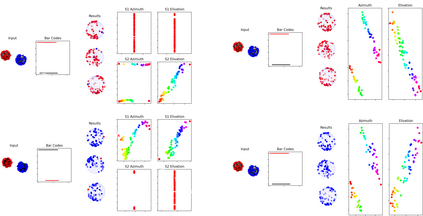
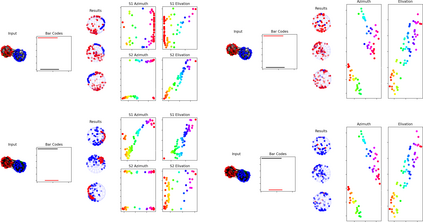
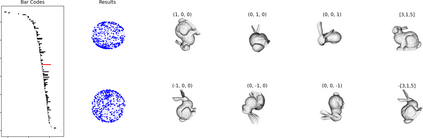
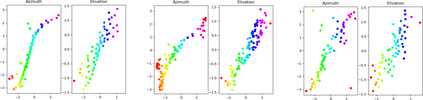
We describe a method to obtain spherical parameterizations of arbitrary data through the use of persistent cohomology and variational optimization. We begin by computing the second-degree persistent cohomology of the filtered Vietoris-Rips (VR) complex of a data set $X$. We extract a cocycle $\alpha$ from any significant feature and define an associated map $\alpha: VR(X) \to S^2$. We use this map as an infeasible initialization for a variational optimization problem with a unique minimizer, up to rigid motion. We employ an alternating gradient descent/ M\"{o}bius transformation update method to solve the problem and generate a more suitable, i.e., smoother, representative of the homotopy class of $\alpha$. We show that this process preserves the relevant topological feature of the data and converges to a feasible optimum. Finally, we conduct numerical experiments on both synthetic and real-world data sets to show the efficacy of our proposed approach.
翻译:我们描述一种通过使用持久性共生学和变异优化获得任意数据的球度参数的方法。 我们首先计算一组数据中经过过滤的Veaoris-Rips(VR)综合体的二度持久性共生学。 我们从任何重要特征中提取一个双循环 $\ alpha$, 并定义一个相关的地图 $\ ALpha: VR(X)\ to S% 2$。 我们用这张地图作为无法应用的初始化方法, 解决使用独特的最小化器的变形优化问题, 直至硬化运动。 我们使用一种交替梯度下沉/ M\\\\\\ { o}bius 更新方法解决问题, 产生更合适的, 即平滑的, 代表同质级 $\ alpha$ 。 我们证明这一过程保留了数据的相关表层特征, 并汇集到一个可行的最佳方法。 最后, 我们在合成和现实世界数据集上进行数字实验, 以显示我们拟议方法的功效。