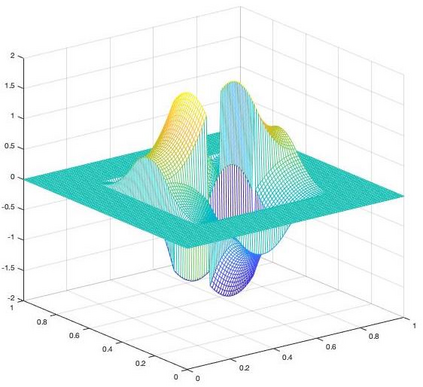
We introduce a very weak space-time variational formulation for the wave equation, prove its well-posedness (even in the case of minimal regularity) and optimal inf-sup stability. Then, we introduce a tensor product-style space-time Petrov-Galerkin discretization with optimal discrete inf-sup stability, obtained by a non-standard definition of the trial space. As a consequence, the numerical approximation error is equal to the residual, which is particularly useful for a posteriori error estimation. For the arising {discrete linear systems} in space and time, we introduce efficient numerical solvers that appropriately exploit the equation structure, either at the preconditioning level or in the approximation phase by using a tailored Galerkin projection. This Galerkin method shows competitive behavior concerning {wall-clock} time, accuracy and memory as compared with a standard time-stepping method in particular in low regularity cases. Numerical experiments with a 3D (in space) wave equation illustrate our findings.
翻译:我们为波形引入了非常弱的空间-时间变异配方,证明它具有很好的储存性(即使是在最低的规律性情况下)和最佳的内流稳定性。然后,我们引入了通过试验空间的非标准定义获得的具有最佳离散性内部稳定性的高压产品式空间-时-时-Petrov-Galerkin 分解。结果,数字近似误差等于剩余误差,对于事后误差估计特别有用。对于在时空中出现的{分辨线性系统},我们引入了高效的数字解算器,在设定前一级或近似阶段适当利用方形结构。这一Galerkin方法显示了与标准时间跨度方法相比的竞争性行为,特别是在低常态情况下。3D(空间)波方程式的数值实验说明了我们的调查结果。