Bernstein-Type Bounds for Beta Distribution

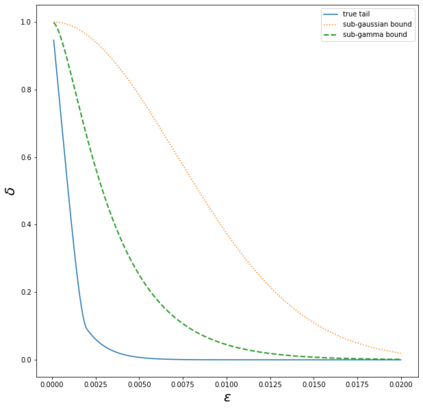
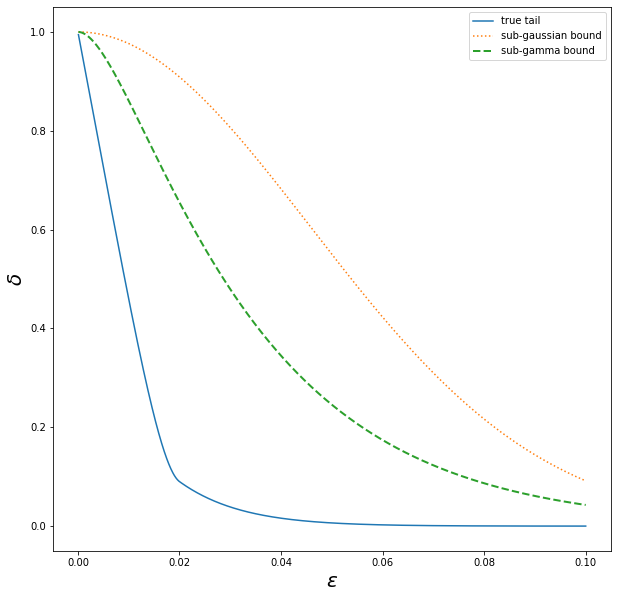
This work establishes Bernstein-type closed-form concentration inequalities for the beta distribution, with optimal variance proxy. For skewed distributions, these bounds are demonstrated to be more accurate then sub-gaussian inequalities from prior works. The approach builds on the recursion obtained from a hyper-geometric representation of the central moments.