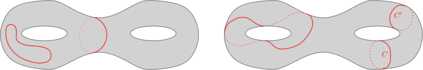
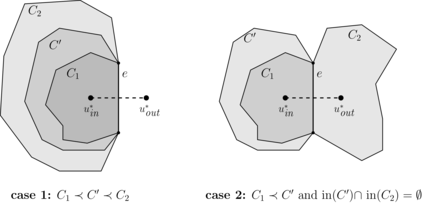
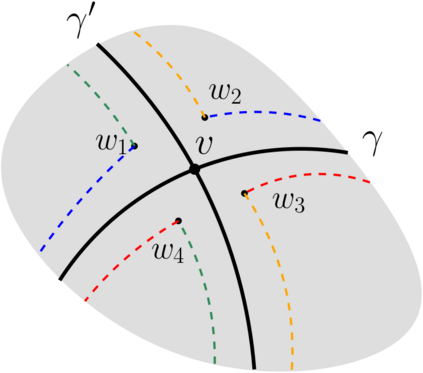
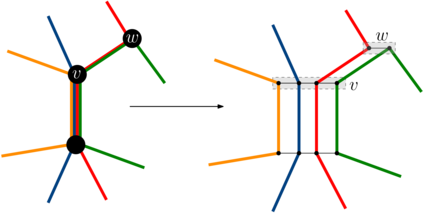
We devise the first constant-factor approximation algorithm for finding an integral multi-commodity flow of maximum total value for instances where the supply graph together with the demand edges can be embedded on an orientable surface of bounded genus. This extends recent results for planar instances. Our techniques include an uncrossing algorithm, which is significantly more difficult than in the planar case, a partition of the cycles in the support of an LP solution into free homotopy classes, and a new rounding procedure for freely homotopic non-separating cycles.
翻译:我们设计了第一个常数要素近似算法,为能够将供应图和需求边缘嵌入受约束的基因的可调整表面时,寻找一个具有最大总价值的综合多通货流。这延及了规划性实例的最新结果。我们的技术包括非交叉算法,这比计划性案例要困难得多,在支持液相溶液溶液后将周期分割成自由的同质类,以及自由同质性非分离周期的新四舍五入程序。
相关内容
Integration:Integration, the VLSI Journal。
Explanation:集成,VLSI杂志。
Publisher:Elsevier。
SIT:http://dblp.uni-trier.de/db/journals/integration/
Arxiv
0+阅读 · 2021年7月22日
Arxiv
0+阅读 · 2021年7月20日