Modeling Performance and Energy trade-offs in Online Data-Intensive Applications

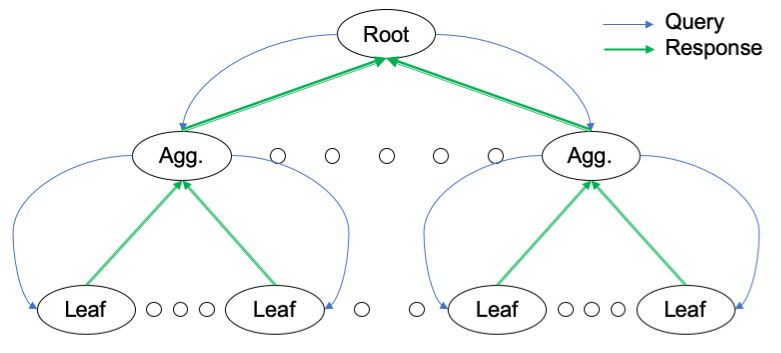
We consider energy minimization for data-intensive applications run on large number of servers, for given performance guarantees. We consider a system, where each incoming application is sent to a set of servers, and is considered to be completed if a subset of them finish serving it. We consider a simple case when each server core has two speed levels, where the higher speed can be achieved by higher power for each core independently. The core selects one of the two speeds probabilistically for each incoming application request. We model arrival of application requests by a Poisson process, and random service time at the server with independent exponential random variables. Our model and analysis generalizes to today's state-of-the-art in CPU energy management where each core can independently select a speed level from a set of supported speeds and corresponding voltages. The performance metrics under consideration are the mean number of applications in the system and the average energy expenditure. We first provide a tight approximation to study this previously intractable problem and derive closed form approximate expressions for the performance metrics when service times are exponentially distributed. Next, we study the trade-off between the approximate mean number of applications and energy expenditure in terms of the switching probability.