Tests for circular symmetry of complex-valued random vectors

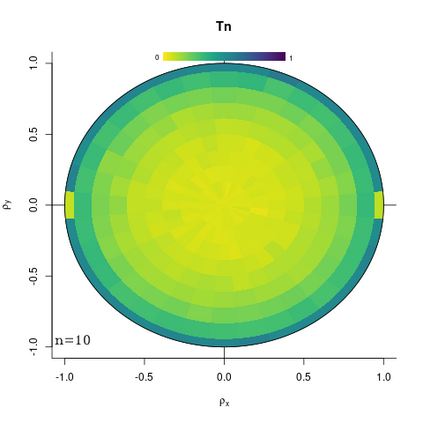
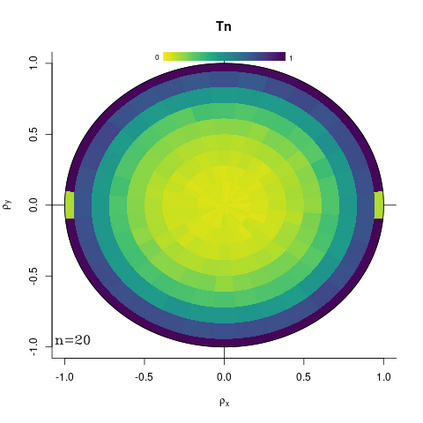
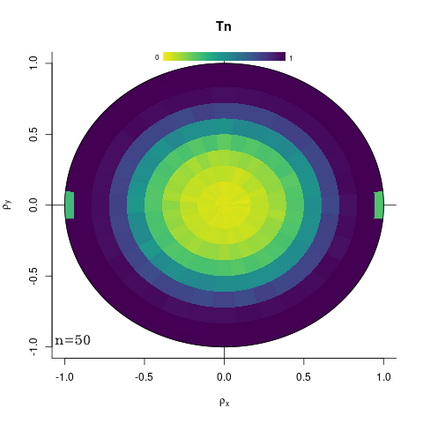
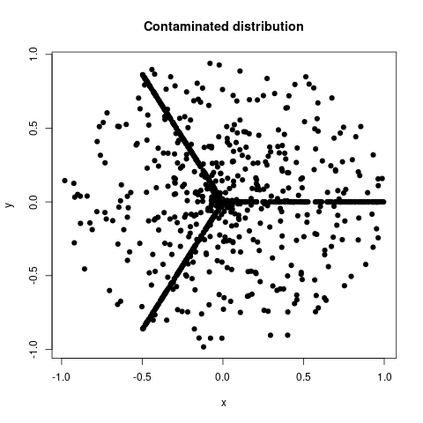
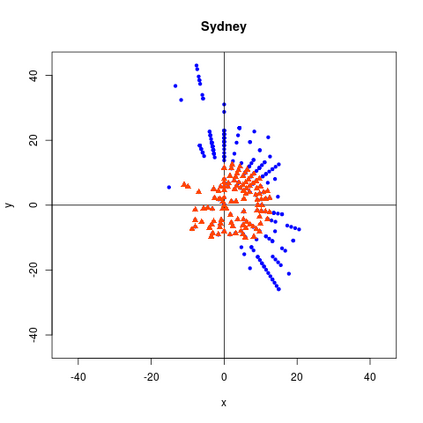
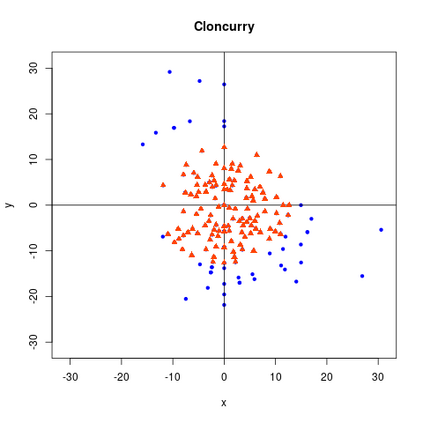
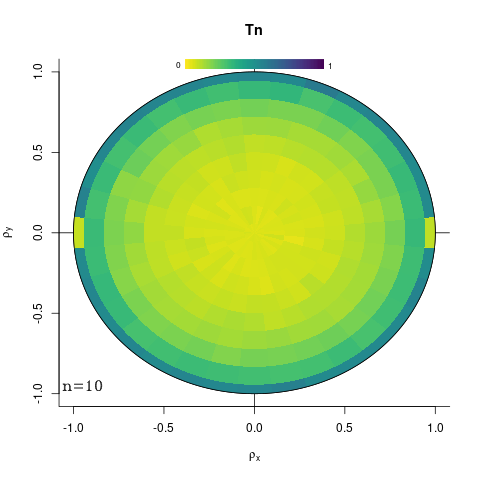
We propose tests for the null hypothesis that the law of a complex-valued random vector is circularly symmetric. The test criteria are formulated as $L^2$-type criteria based on empirical characteristic functions, and they are convenient from the computational point of view. Asymptotic as well as Monte-Carlo results are presented. Applications on real data are also reported. An R package called CircSymTest is available from the authors.