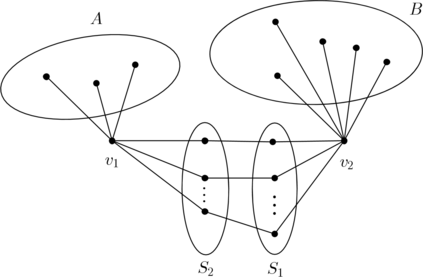
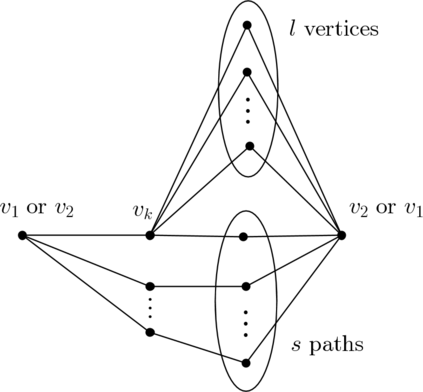
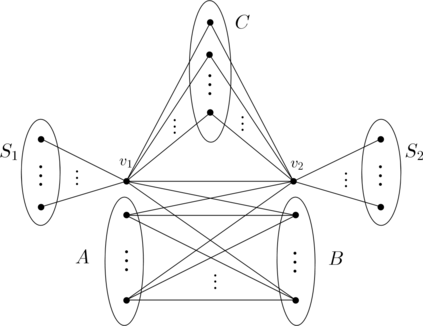
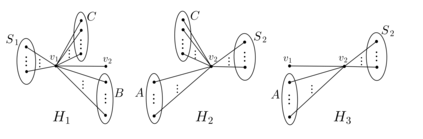
For a graph $G$, let $\lambda_2(G)$ denote its second smallest Laplacian eigenvalue. It was conjectured that $\lambda_2(G) + \lambda_2(\overline{G}) \geq 1$, where $\bar{G}$ is the complement of $G$. Here, we prove this conjecture in the general case. Also, we will show that $\max\{\lambda_2(G), \lambda_2(\overline{G})\} \geq 1 - O(n^{-\frac 13})$, where $n$ is the number of vertices of $G$.
翻译：以G$为单位, 请用$lambda_ 2( G) 表示其第二最小的 Laplacian egenvalue。 推测是$\lambda_ 2( G) +\ lambda_ 2 ( G) +\ lambda_ 2( overline{ G})\ geq 1$, 其中$\ bar{ G} 是 G美元的补充。 在此, 我们证明一般情况下的这一推测。 另外, 我们将显示$\ max\ lambda_ 2 ( G),\ lambda_ 2( overline{ G} \\\ geq 1 - O (n\\\\\\\\\\\ frac 13} $, 其中美元是 G美元。
相关内容
Arxiv
0+阅读 · 2021年8月25日
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2021年8月25日
微信扫码咨询专知VIP会员