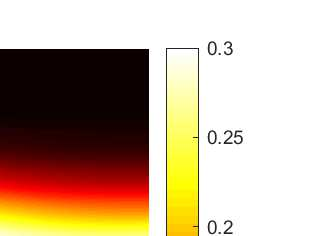
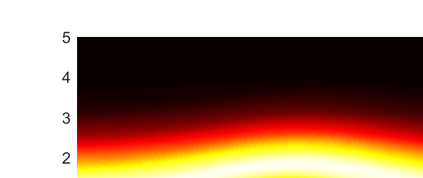
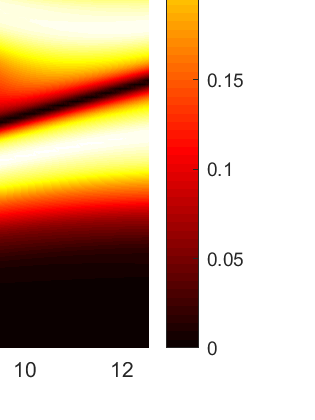
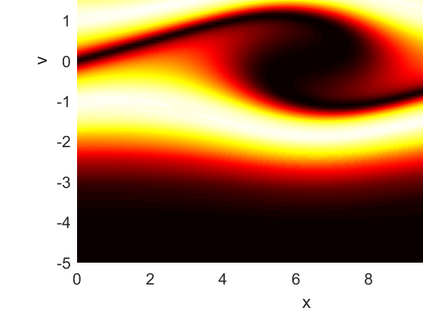
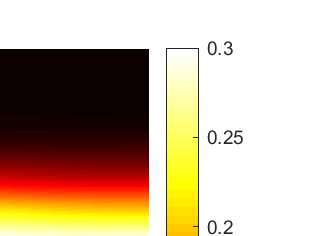
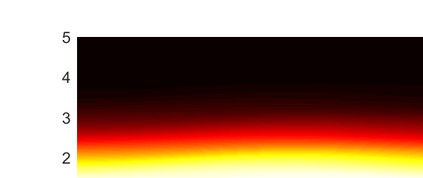
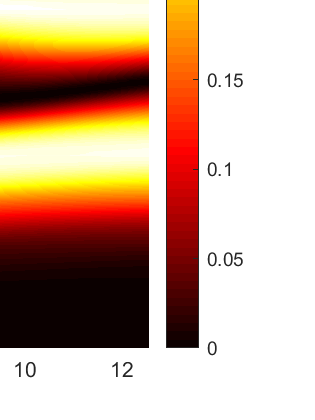
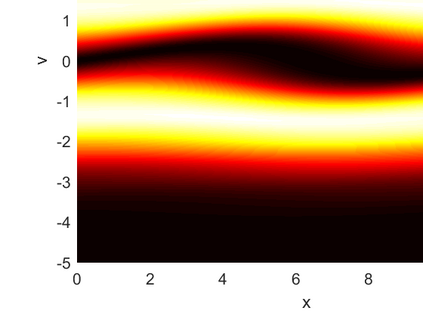
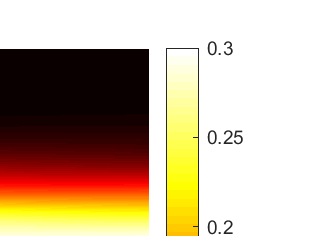
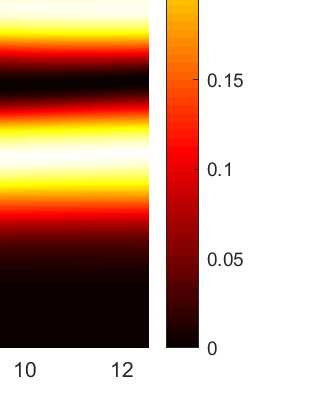
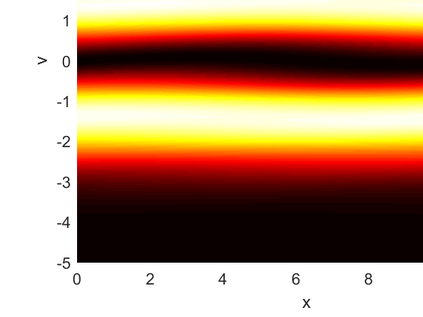
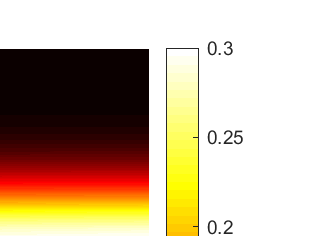
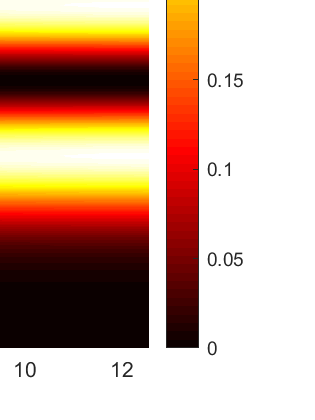
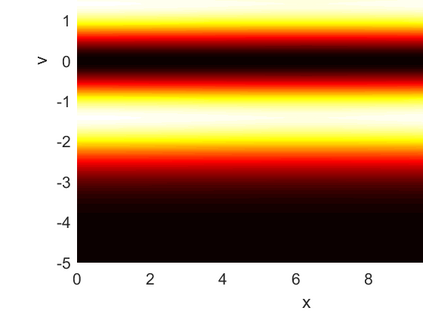
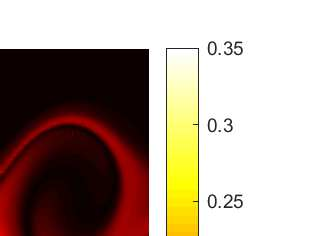
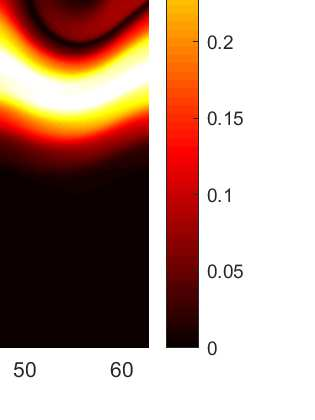
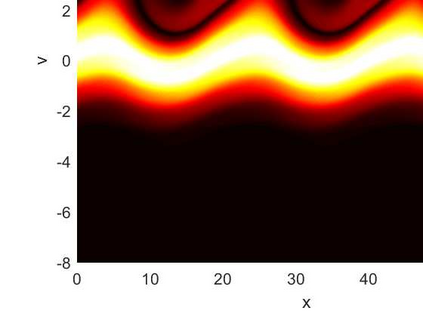
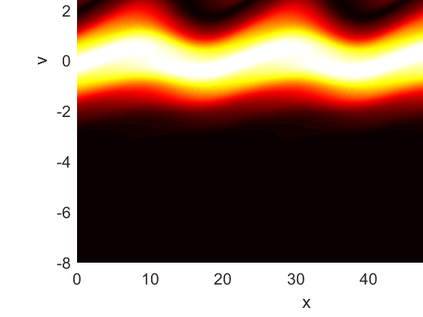
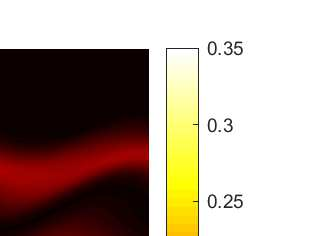
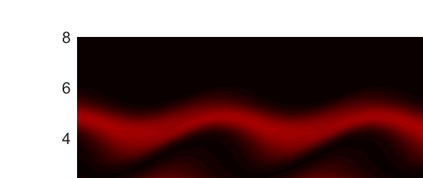
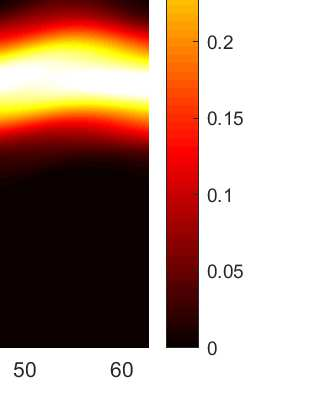
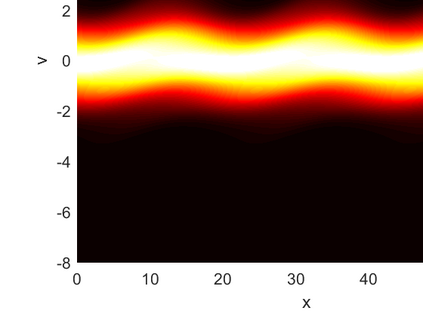
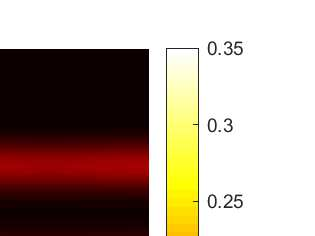
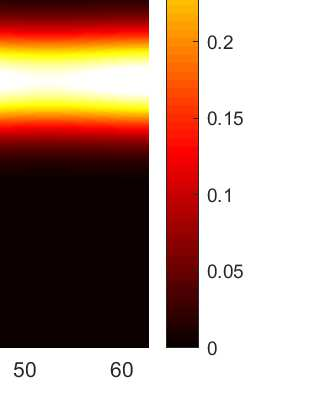
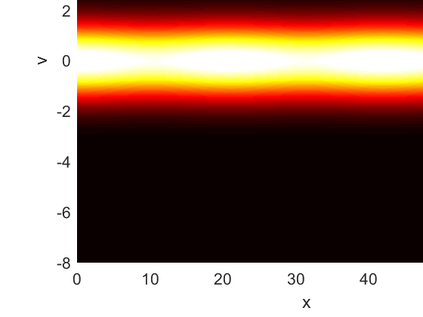
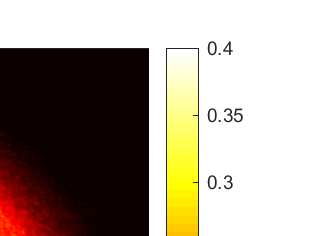
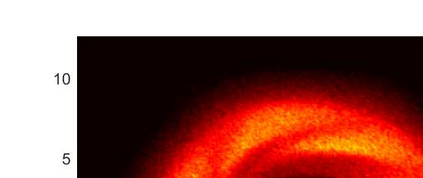
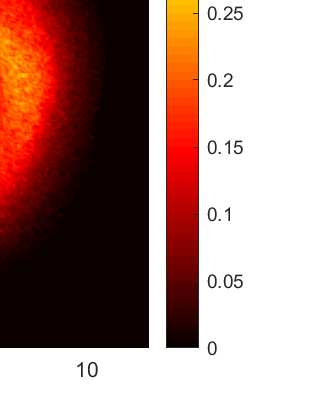
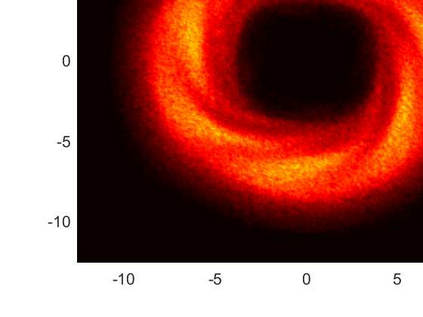
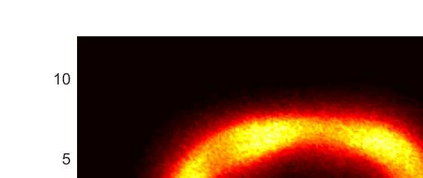
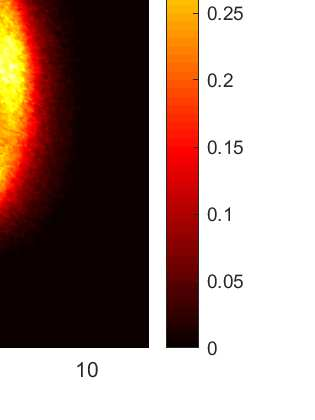
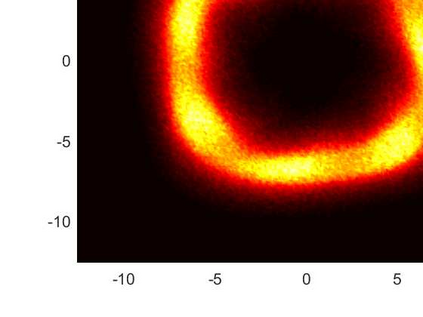
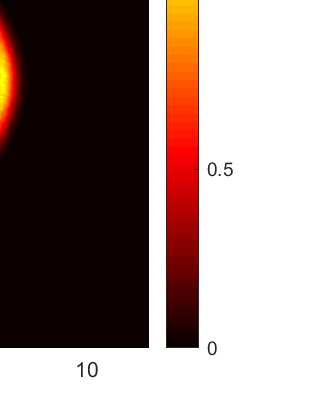
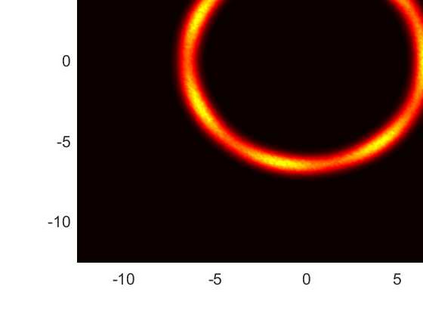
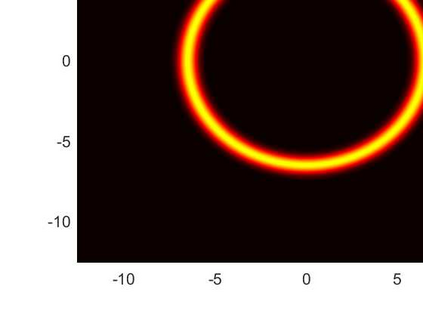
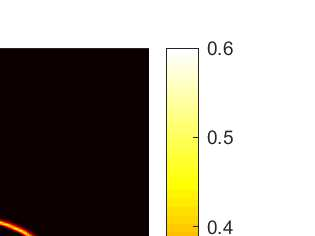
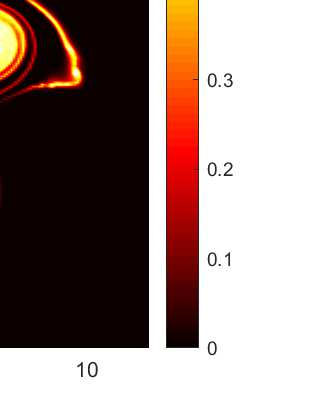
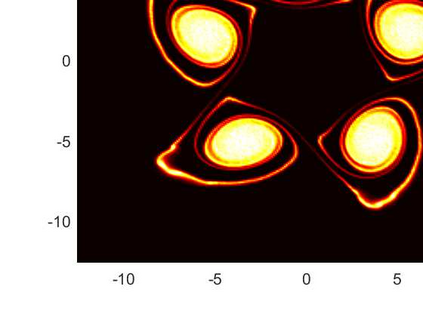
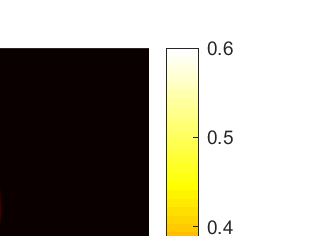
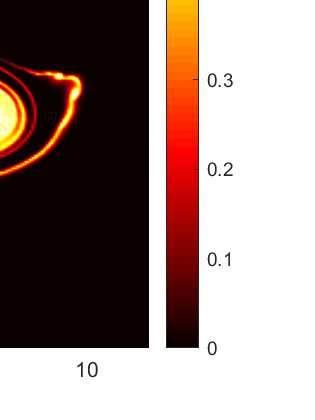
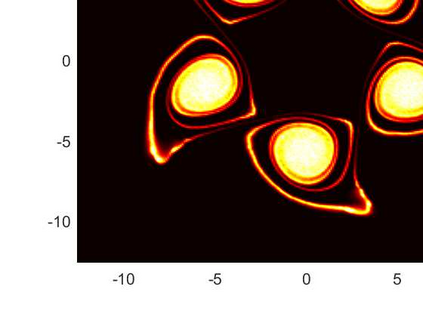
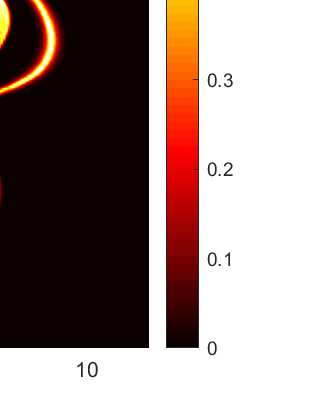
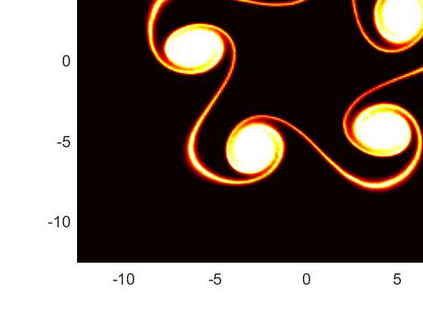
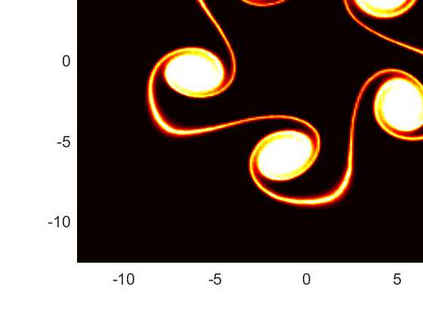
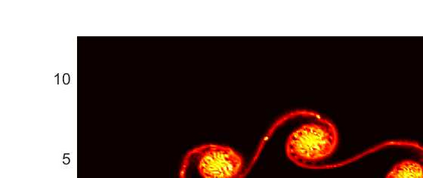
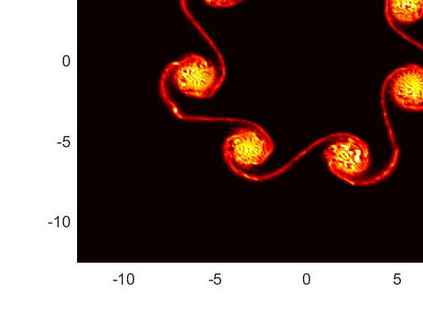
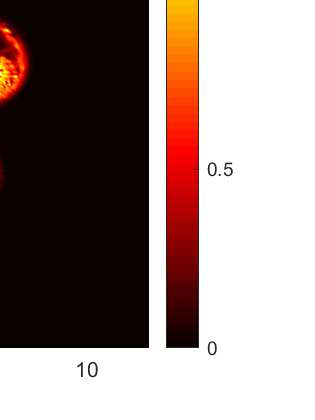
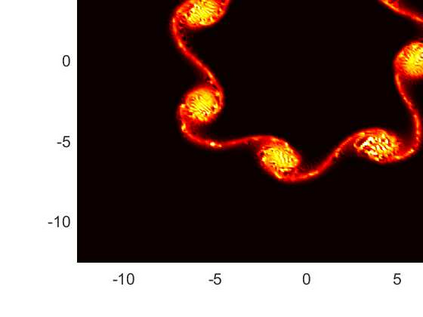
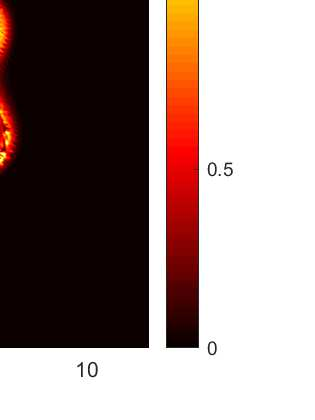
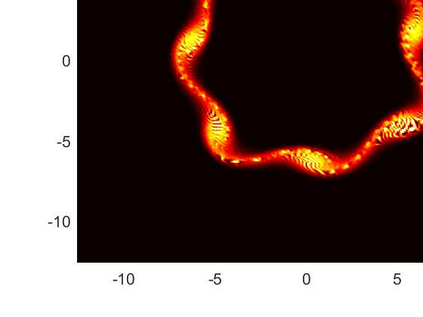
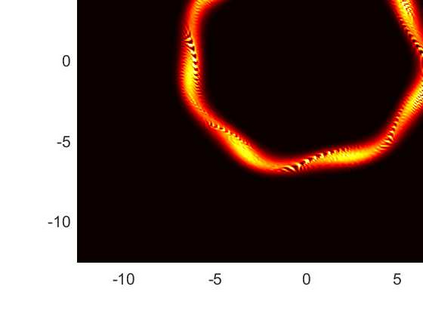
In this paper, Particle-in-Cell algorithms for the Vlasov-Poisson system are presented based on its Poisson bracket structure. The Poisson equation is solved by finite element methods, in which the appropriate finite element spaces are taken to guarantee that the semi-discretized system possesses a well defined discrete Poisson bracket structure. Then, splitting methods are applied to the semi-discretized system by decomposing the Hamiltonian function. The resulting discretizations are proved to be Poisson bracket preserving. Moreover, the conservative quantities of the system are also well preserved. In numerical experiments, we use the presented numerical methods to simulate various physical phenomena. Due to the huge computational effort of the practical computations, we employ the strategy of parallel computing. The numerical results verify the efficiency of the new derived numerical discretizations.
翻译:在本文中, Vlasov-Poisson 系统的分解算法以其 Poisson 括号结构为基础。 Poisson 等式通过有限元素方法解析,其中采用适当的有限元素空间以保证半分解系统拥有一个定义清晰的离散Poisson 括号结构。然后,对半分解系统应用分解方法,将汉密尔顿函数分解。由此产生的分解方法被证明是保有的。此外,这个系统的保守数量也得到了很好的保存。在数字实验中,我们使用所提出的数字方法模拟各种物理现象。由于实际计算的巨大计算努力,我们采用了平行计算的战略。数字结果验证了新衍生的数字分解法的效率。