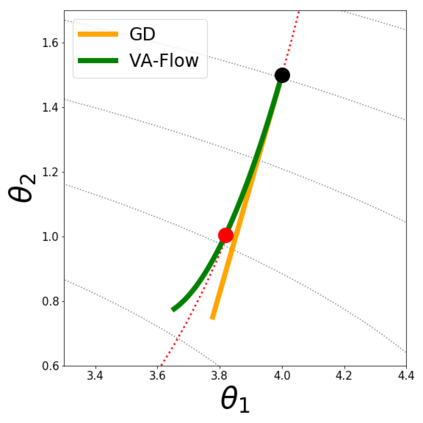
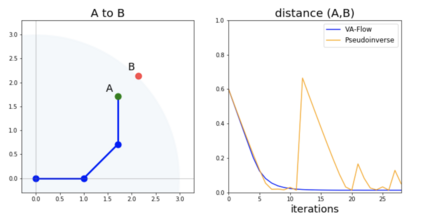
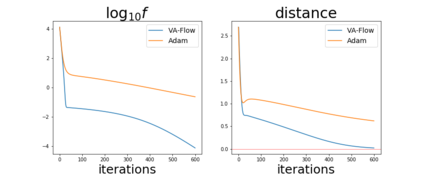
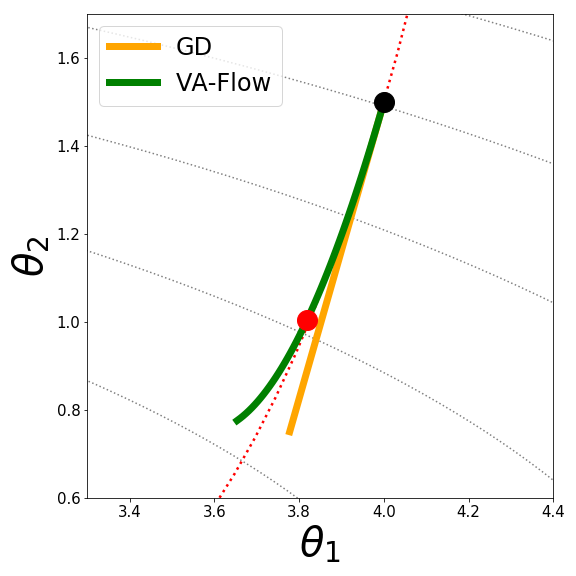
It has long been a goal to efficiently compute and use second order information on a function ($f$) to assist in numerical approximations. Here it is shown how, using only basic physics and a numerical approximation, such information can be accurately obtained at a cost of ${\cal O}(N)$ complexity, where $N$ is the dimensionality of the parameter space of $f$. In this paper, an algorithm ({\em VA-Flow}) is developed to exploit this second order information, and pseudocode is presented. It is applied to two classes of problems, that of inverse kinematics (IK) and gradient descent (GD). In the IK application, the algorithm is fast and robust, and is shown to lead to smooth behavior even near singularities. For GD the algorithm also works very well, provided the cost function is locally well-described by a polynomial.
翻译:长期以来,它一直是高效计算并使用函数的第二顺序信息(f美元)来帮助数字近似。 这里显示的是,仅使用基本物理和数字近似,如何以$=cal O}(N) 的复杂度来准确获得这种信息,而美元是参数空间的维度(f美元)。 在本文中,开发了一种算法(@em VA-Flow})来利用第二顺序信息,并提供了伪代码。它适用于两类问题,即反动运动和梯度下行(GD)。在 IK 应用程序中,算法既快速又稳健,并显示可以导致平稳的行为,甚至接近奇数。对于GD 算法也非常有效,只要成本函数是本地的,由多元函数很好地描述。