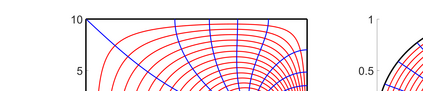
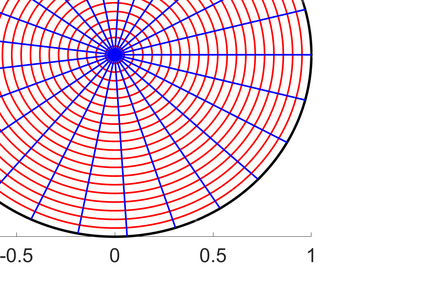
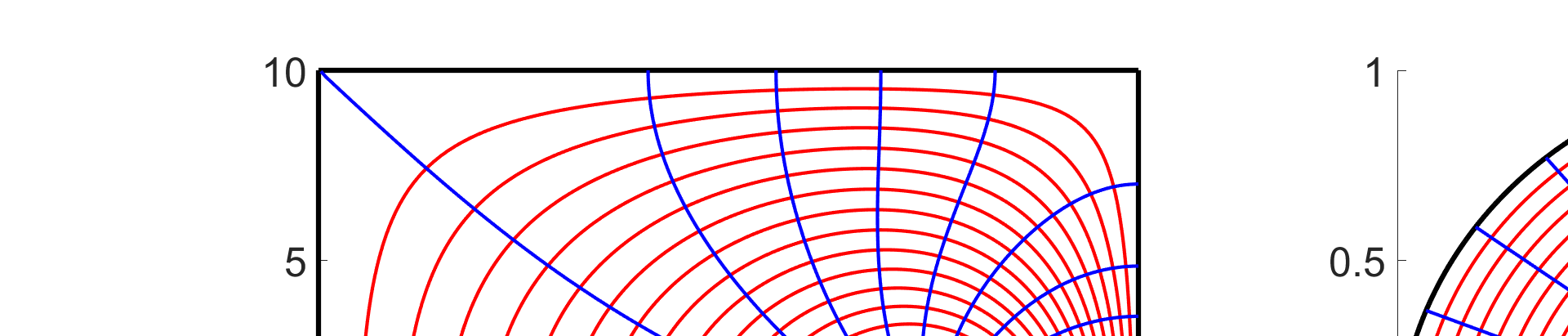
In this paper we present a theoretical background of a coupled analytical-numerical approach to model a crack propagation process in two-dimensional bounded domains. The goal of the coupled analytical-numerical approach is to obtain the correct solution behaviour near the crack tip by help of the analytical solution constructed by using tools of the complex function theory and couple it continuously with the finite element solution in the region far from singularity. In this way, crack propagation could be modelled without using remeshing. Possible directions of crack growth can be calculated through the minimization of the total energy composed of the potential energy and the dissipated energy based on the energy release rate. Within this setting, an analytical solution of a mixed boundary value problem based on complex analysis and conformal mapping techniques is presented in a circular region containing an arbitrary crack path. More precisely, the linear elastic problem is transformed into a Riemann-Hilbert problem in the unit disk for holomorphic functions. Utilising advantages of the analytical solution in the region near the crack tip, the total energy could be evaluated within short computation times for various crack kink angles and lengths leading to a potentially efficient way of computing the minimization procedure. To this end, the paper presents a general strategy of the new coupled approach for crack propagation modelling. Additionally, we also discuss obstacles on the way of practical realisation of this strategy.
翻译:在本文中,我们展示了在两维界限域模拟裂缝传播过程的结合分析数字方法的理论背景。结合分析数字方法的目标是,通过使用复杂功能理论工具构建的分析解决方案,帮助在裂缝附近找到正确的解决方案行为。通过使用复杂功能理论工具构建的分析解决方案,并连续将其与该地区有限的元素解决方案相结合,远非奇特性。这样,可以模拟裂缝传播,而不必使用再映射。裂缝增长的可能方向可以通过最大限度地减少由潜在能量组成的总能量和基于能源释放率的散射能量来计算。在这一背景下,基于复杂分析和符合性绘图技术的混合边界价值问题的分析解决方案在包含任意裂缝路径的圆形区域中呈现出来。更准确地说,线性弹性问题被转化成全局功能单元磁盘中的里曼-希尔伯特问题。在接近裂缝的区域利用分析解决方案的优势,总能量可以在短的计算时间内对各种裂缝和长度组成的能量进行评估,最终导致一个潜在的断裂缝和长度的混合边界价值问题的分析解决方案,在包含任意裂缝路径的循环区域提出。更精确的弹性弹性弹性的弹性弹性弹性弹性弹性弹性弹性分析策略,我们用新的模型来讨论这一模型化战略。