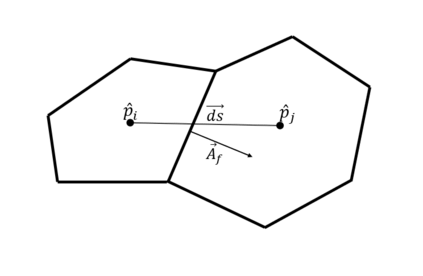
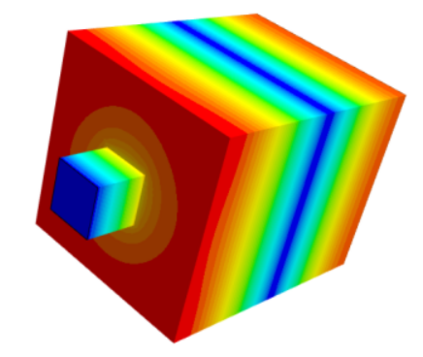
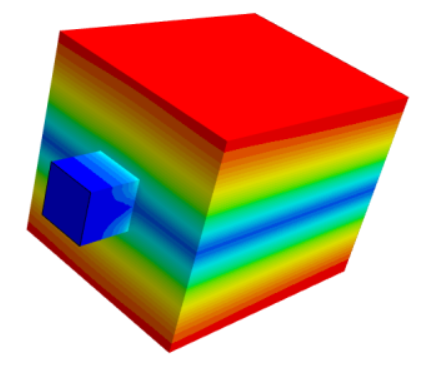
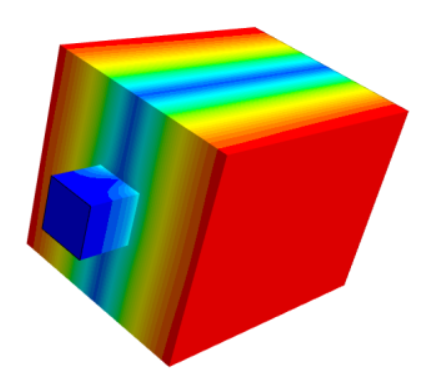
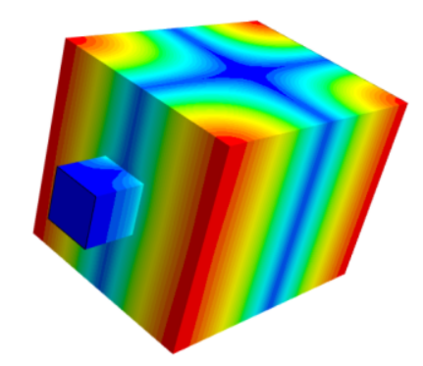
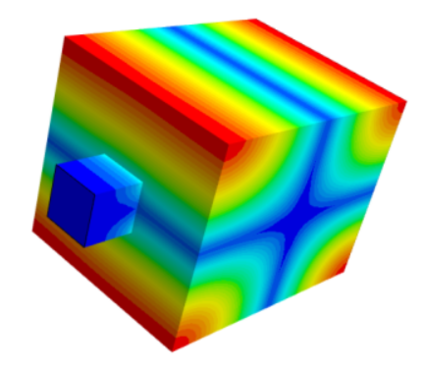
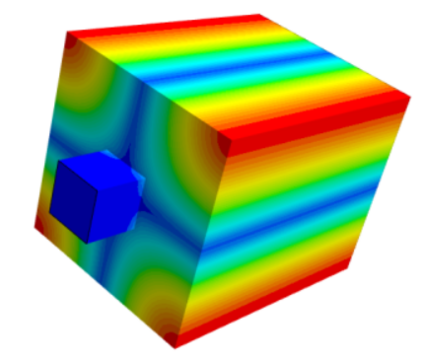
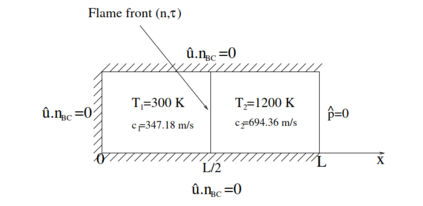
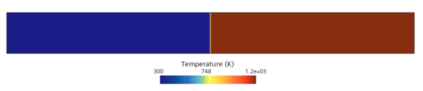
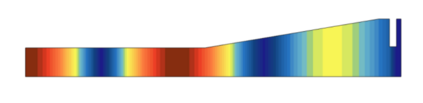
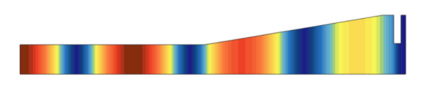
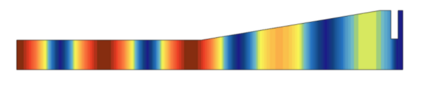
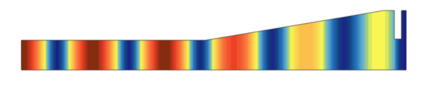
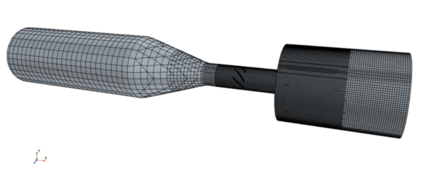
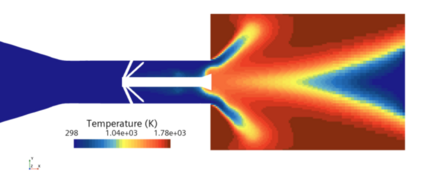
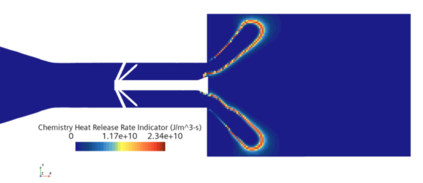
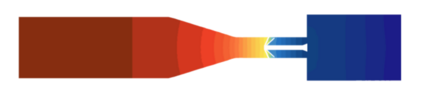
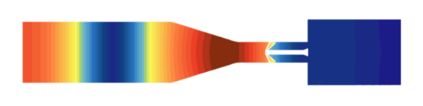
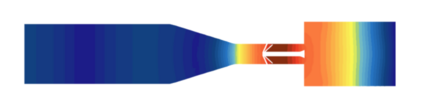
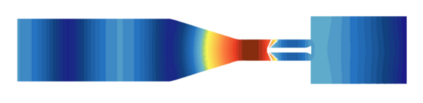
Closed combustion devices like gas turbines and rockets are prone to thermoacoustic instabilities. Design engineers in the industry need tools to accurately identify and remove instabilities early in the design cycle. Many different approaches have been developed by the researchers over the years. In this work we focus on the Helmholtz wave equation based solver which is found to be relatively fast and accurate for most applications. This solver has been a subject of study in many previous works. The Helmholtz wave equation in frequency space reduces to a nonlinear eigenvalue problem which needs to be solved to compute the acoustic modes. Most previous implementations of this solver have relied on linearized solvers and iterative methods which as shown in this work are not very efficient and sometimes inaccurate. In this work we make use of specialized algorithms implemented in SLEPc that are accurate and efficient for computing eigenvalues of nonlinear eigenvalue problems. We make use of the n-tau model to compute the reacting source terms in the Helmholtz equation and describe the steps involved in deriving the Helmholtz eigenvalue equation and obtaining its solution using the SLEPc library.
翻译:燃气涡轮机和火箭等封闭燃烧装置容易产生热声不稳。 工业的设计工程师需要工具来准确识别和清除设计周期早期的不稳定性。 研究人员多年来已经开发了许多不同的方法。 在这项工作中,我们把重点放在基于Helmholtz波方程式的求解器上,发现该求解器对大多数应用来说比较快和准确。 这个求解器是许多以前工作的一项研究课题。 频率空间中的Helmholtz波方程式将降低为非线性二元值问题,需要解决以计算声学模式。 这个求解器以前的大多数实施都依赖于线性解解解器和迭接方法,而这项工作显示这些求解器和迭接方法效率不高,有时不准确。 在这项工作中,我们使用了SLEPc 中执行的专门算法,该算出非线性乙基值问题的电子元值。 我们利用n- tau 模型来计算Helmhotz方程式中的反应源术语,需要加以解决来计算声调调调调调调调调调调调解调调调调调调调调调调调调调解调解解解解解解解的解解解解解解解解的解解的解的解的解的解解解解解解调解解解解解解解解解解解解解解解解的解的解的解的解的解的解器。