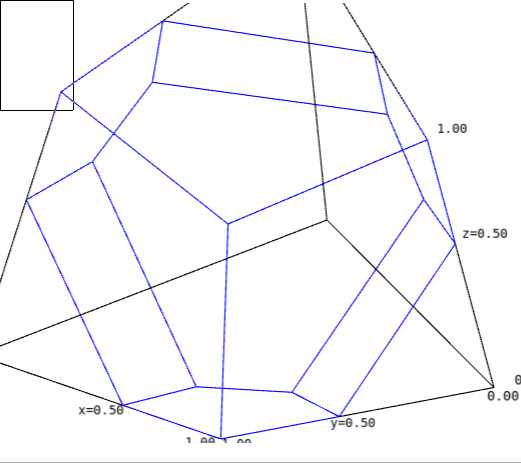
An elimination tree for a connected graph $G$ is a rooted tree on the vertices of $G$ obtained by choosing a root $x$ and recursing on the connected components of $G-x$ to produce the subtrees of $x$. Elimination trees appear in many guises in computer science and discrete mathematics, and they encode many interesting combinatorial objects, such as bitstrings, permutations and binary trees. We apply the recent Hartung-Hoang-M\"utze-Williams combinatorial generation framework to elimination trees, and prove that all elimination trees for a chordal graph $G$ can be generated by tree rotations using a simple greedy algorithm. This yields a short proof for the existence of Hamilton paths on graph associahedra of chordal graphs. Graph associahedra are a general class of high-dimensional polytopes introduced by Carr, Devadoss, and Postnikov, whose vertices correspond to elimination trees and whose edges correspond to tree rotations. As special cases of our results, we recover several classical Gray codes for bitstrings, permutations and binary trees, and we obtain a new Gray code for partial permutations. Our algorithm for generating all elimination trees for a chordal graph $G$ can be implemented in time $\mathcal{O}(m+n)$ per generated elimination tree, where $m$ and $n$ are the number of edges and vertices of $G$, respectively. If $G$ is a tree, we improve this to a loopless algorithm running in time $\mathcal{O}(1)$ per generated elimination tree. We also prove that our algorithm produces a Hamilton cycle on the graph associahedron of $G$, rather than just Hamilton path, if the graph $G$ is chordal and 2-connected. Moreover, our algorithm characterizes chordality, i.e., it computes a Hamilton path on the graph associahedron of $G$ if and only if $G$ is chordal.
翻译:连接的图形 $G$ 的消除树是一个根树 。 我们将最近的 Hartung- Hoang- M\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\G\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\\