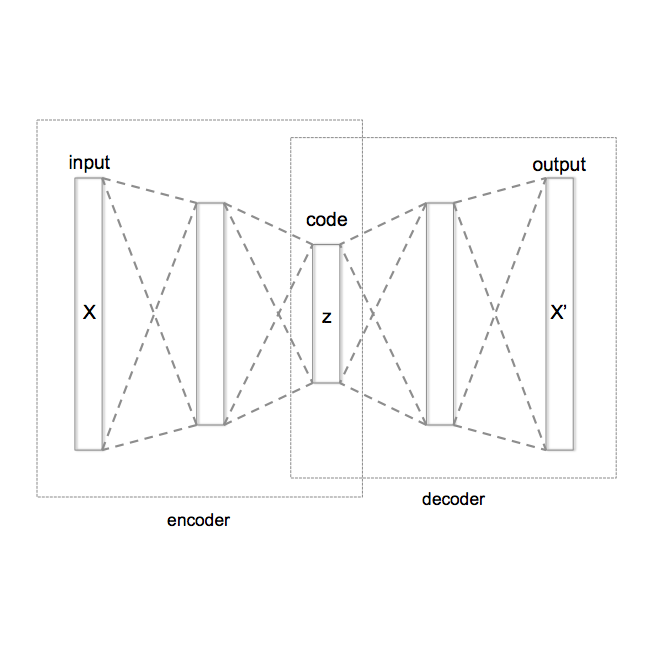
Monte Carlo Markov Chain (MCMC) methods commonly confront two fundamental challenges: the accurate characterization of the prior distribution and the efficient evaluation of the likelihood. In the context of Bayesian studies on tomography, principal component analysis (PCA) can in some cases facilitate the straightforward definition of the prior distribution, while simultaneously enabling the implementation of accurate surrogate models based on polynomial chaos expansion (PCE) to replace computationally intensive full-physics forward solvers. When faced with scenarios where PCA does not offer a direct means of easily defining the prior distribution alternative methods like deep generative models (e.g., variational autoencoders (VAEs)), can be employed as viable options. However, accurately producing a surrogate capable of capturing the intricate non-linear relationship between the latent parameters of a VAE and the outputs of forward modeling presents a notable challenge. Indeed, while PCE models provide high accuracy when the input-output relationship can be effectively approximated by relatively low-degree multivariate polynomials, this condition is typically unmet when utilizing latent variables derived from deep generative models. In this contribution, we present a strategy that combines the excellent reconstruction performances of VAE in terms of prio representation with the accuracy of PCA-PCE surrogate modeling in the context of Bayesian ground penetrating radar (GPR) travel-time tomography. Within the MCMC process, the parametrization of the VAE is leveraged for prior exploration and sample proposal. Concurrently, modeling is conducted using PCE, which operates on either globally or locally defined principal components of the VAE samples under examination.
翻译:暂无翻译