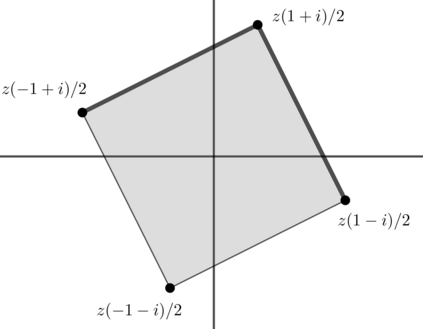
Secret Sharing Schemes (SSS) are methods for distributing a secret among a set of participants. One of the first Secret Sharing Schemes was proposed by M. Mignotte, based on the Chinese remainder theorem over the ring of integers. In this article we extend the Mignotte's scheme to the ring of Gaussian Integers and study some of its properties. While doing this we aim to solve a gap in a previous construction of such extension. In addition we show that any access structure can be made through a SSS over $ \mathbb{Z}[i]$.
翻译:秘密分享计划(SSS)是一组参与者之间分配秘密的方法。 第一批秘密分享计划之一由M. Mignotte提出, 其依据是整数圈的中国剩余理论。 本条将Mignotte的计划扩展至高山整数圈, 并研究其中的一些属性。 在这样做的时候, 我们的目标是解决先前构建的这种扩展中的空白。 此外, 我们还表明, 任何访问结构都可以通过SSS超过$\mathbb ⁇ [i] 来建立。
相关内容
医学人工智能AIM(Artificial Intelligence in Medicine)杂志发表了多学科领域的原创文章,涉及医学中的人工智能理论和实践,以医学为导向的人类生物学和卫生保健。医学中的人工智能可以被描述为与研究、项目和应用相关的科学学科,旨在通过基于知识或数据密集型的计算机解决方案支持基于决策的医疗任务,最终支持和改善人类护理提供者的性能。
官网地址:http://dblp.uni-trier.de/db/journals/artmed/
专知会员服务
17+阅读 · 2020年6月4日
Arxiv
0+阅读 · 2021年8月24日
Arxiv
0+阅读 · 2021年8月20日
Arxiv
3+阅读 · 2020年4月13日
Arxiv
8+阅读 · 2018年11月21日