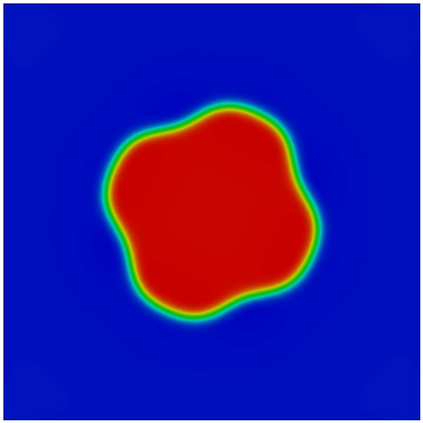
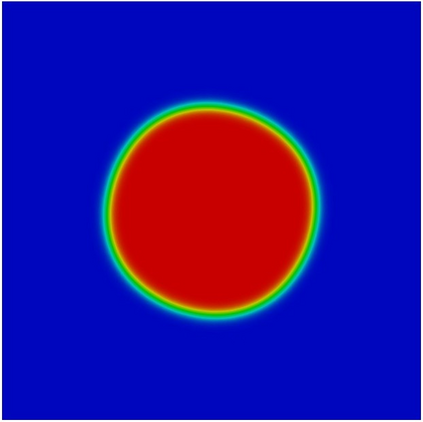
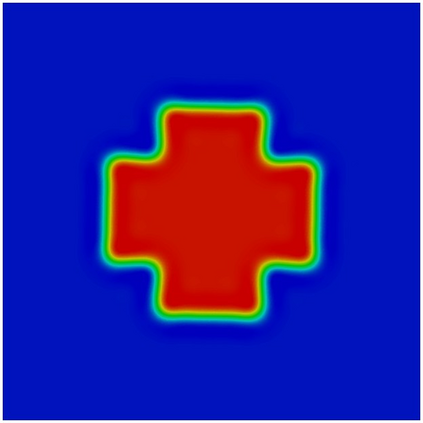
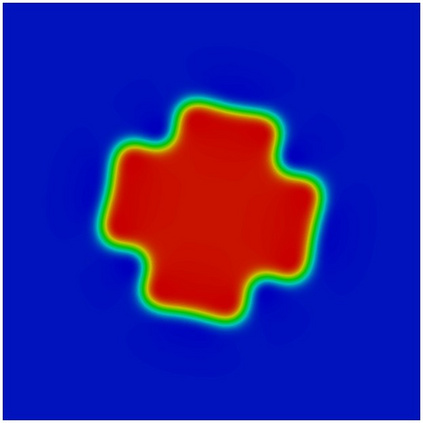
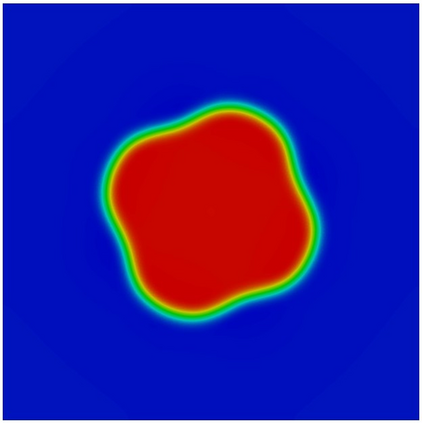
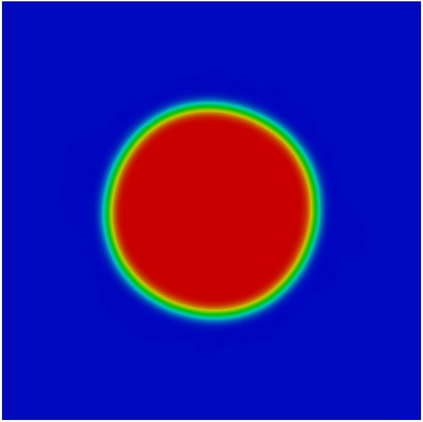
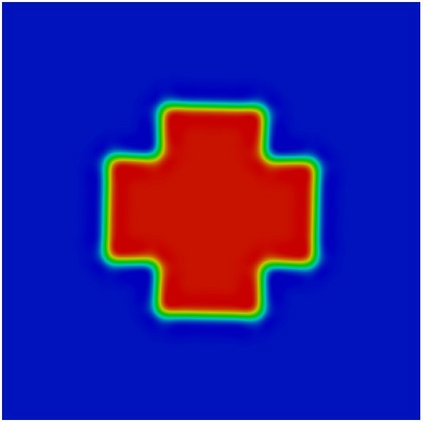
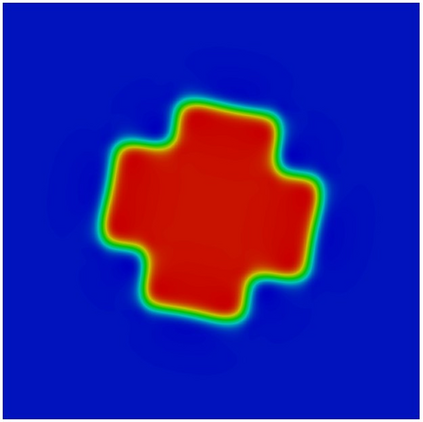
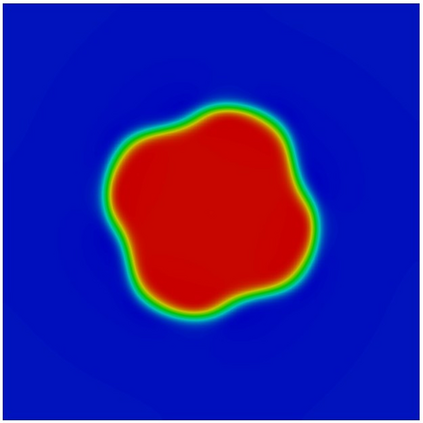
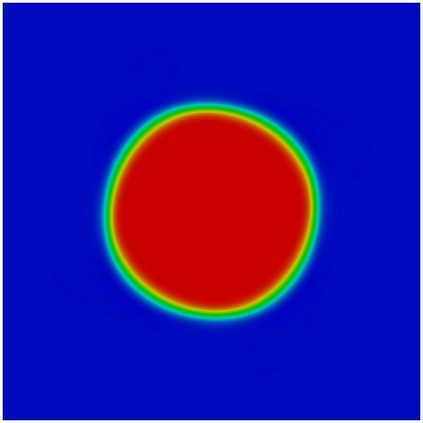
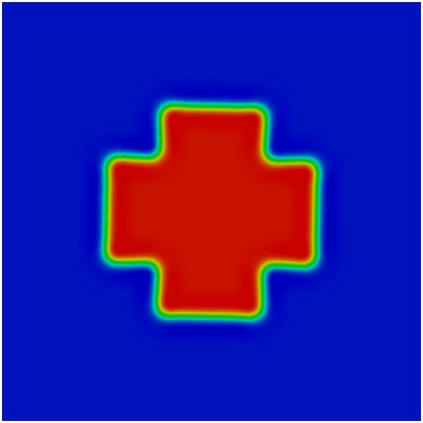
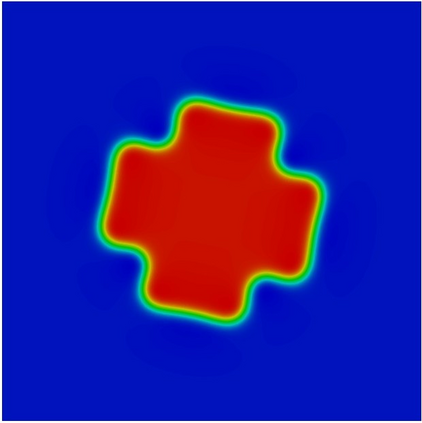
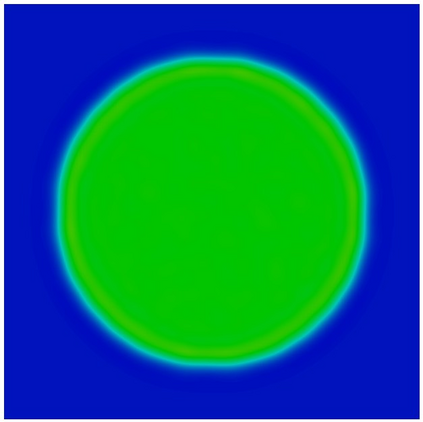
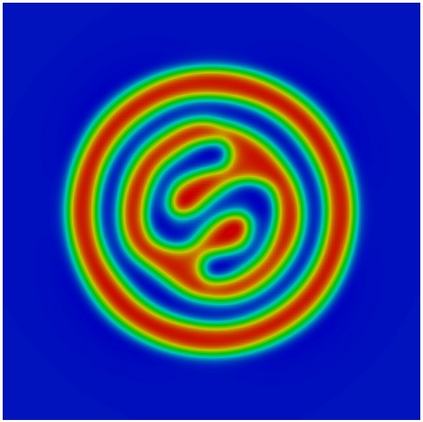
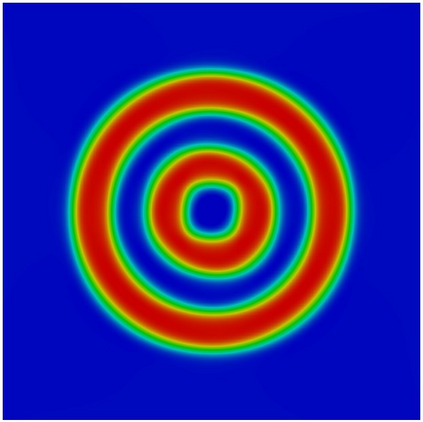
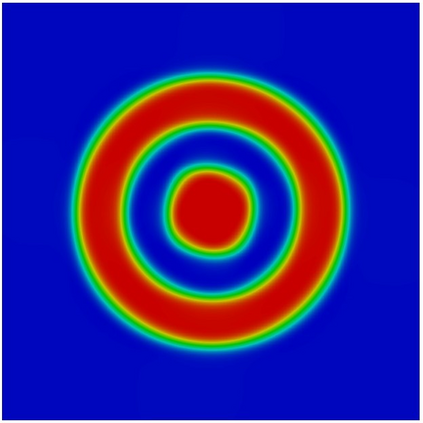
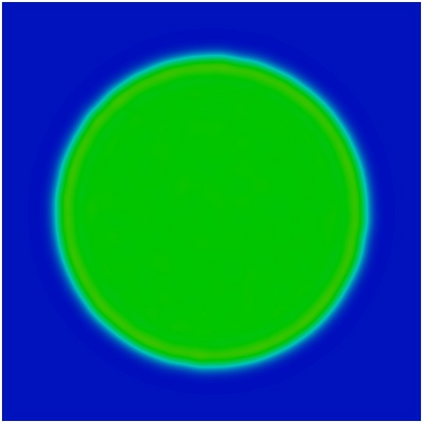
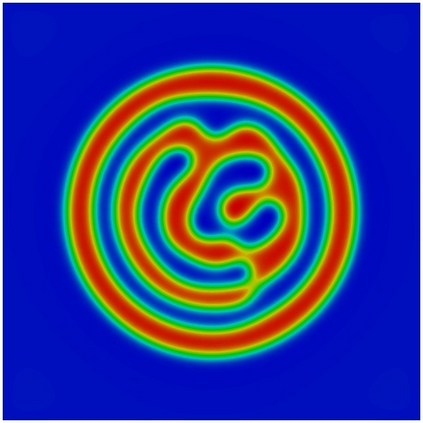
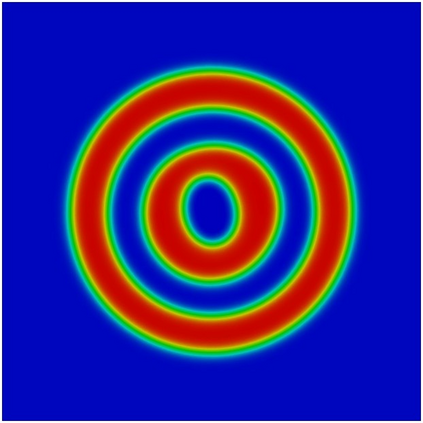
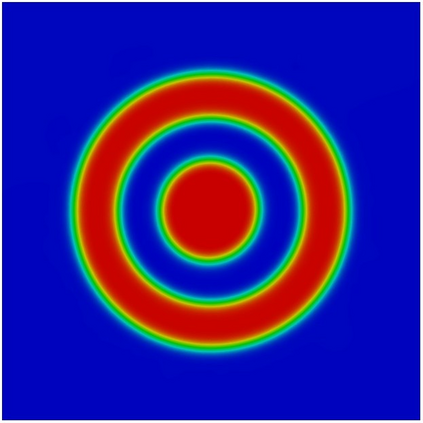
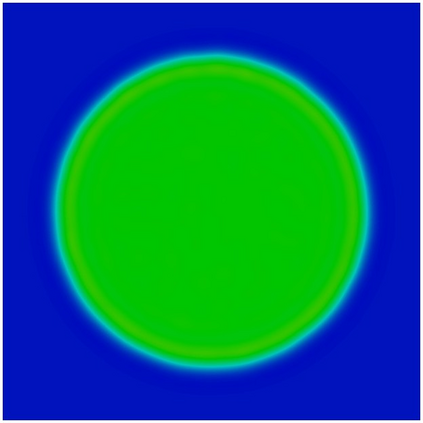
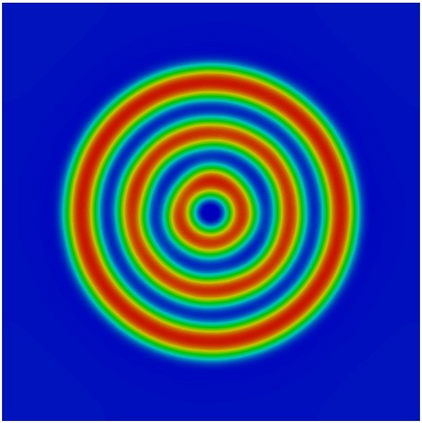
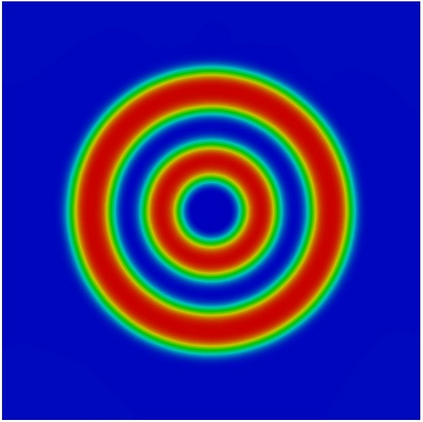
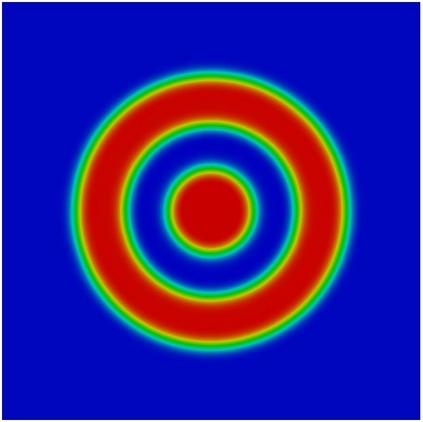
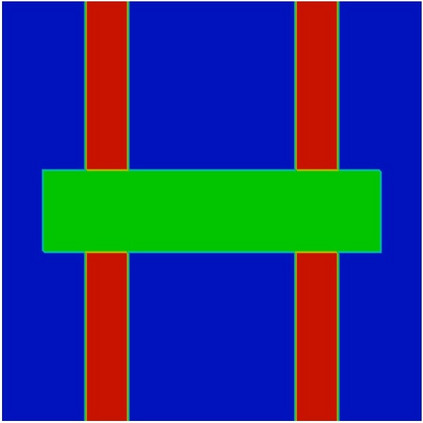
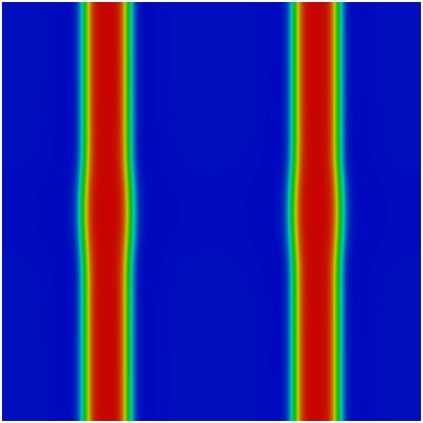
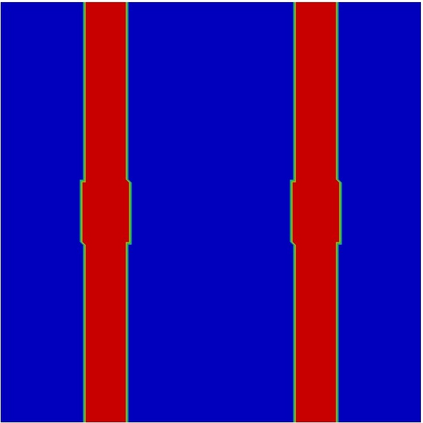
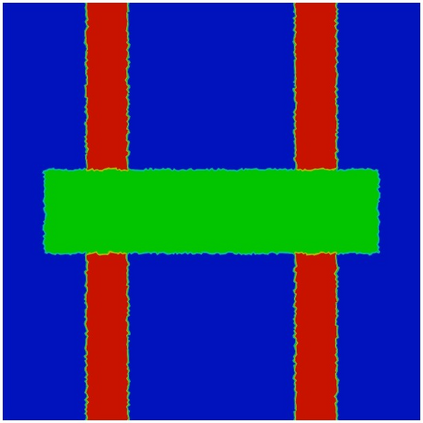
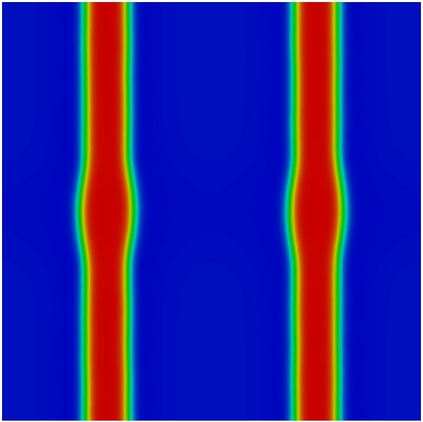
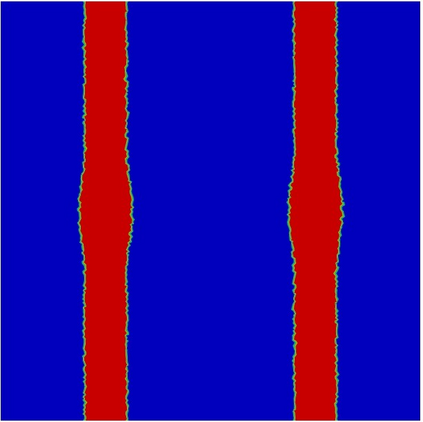
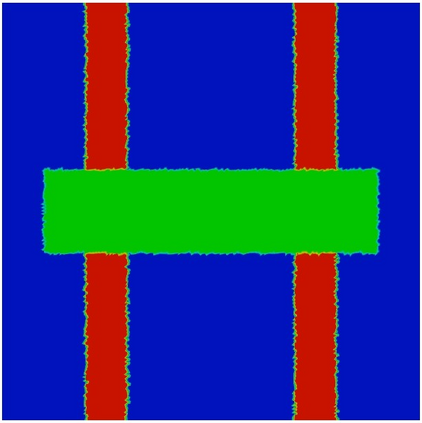
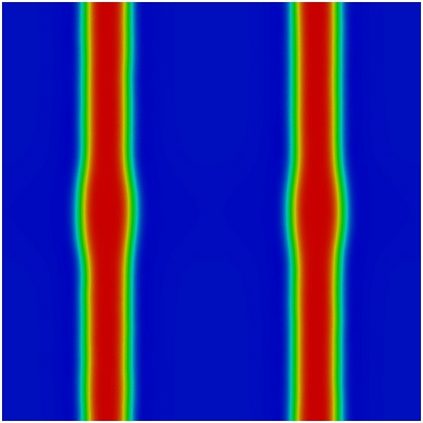
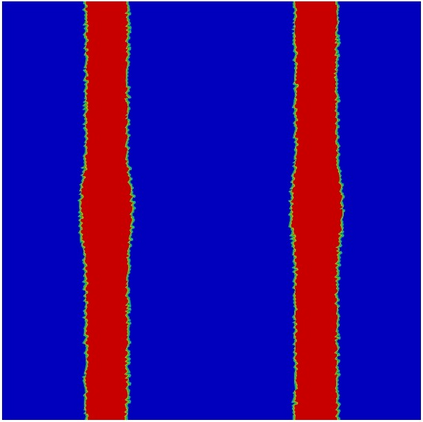
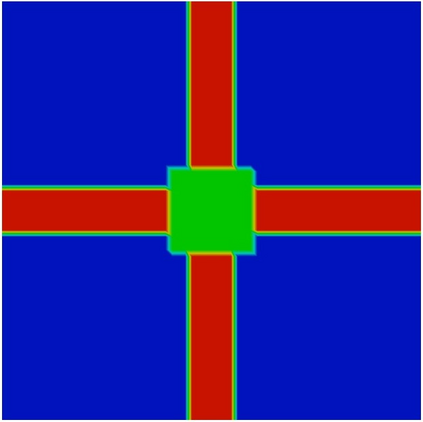
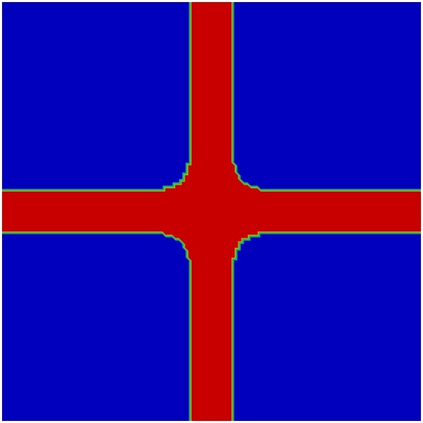
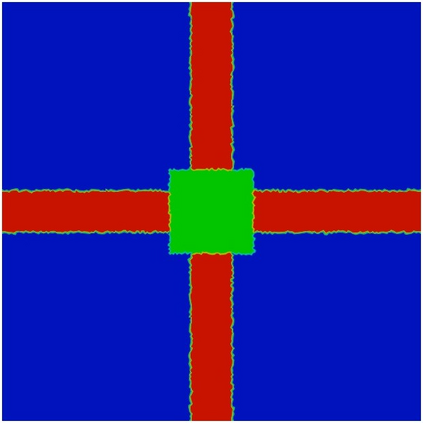
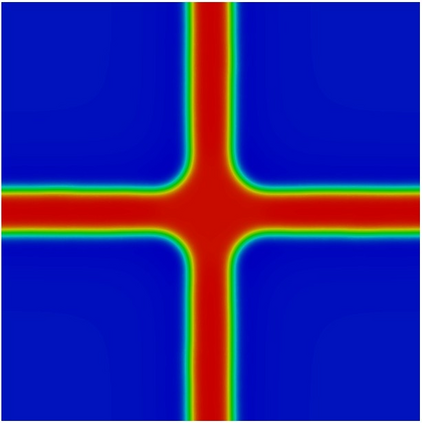
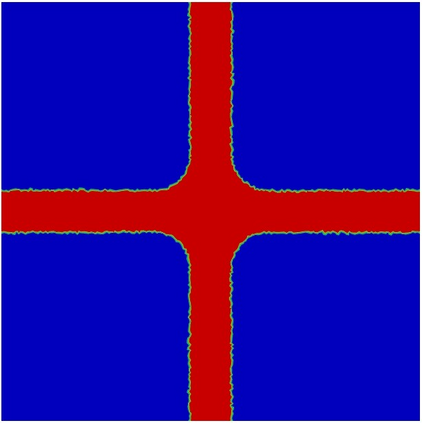
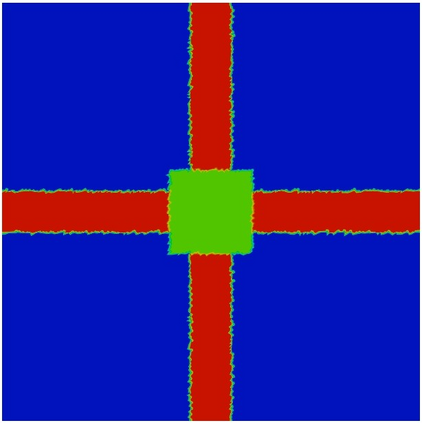
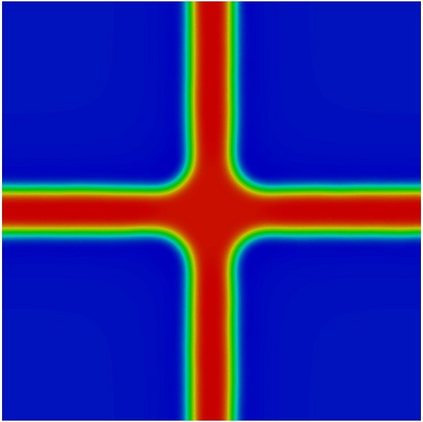
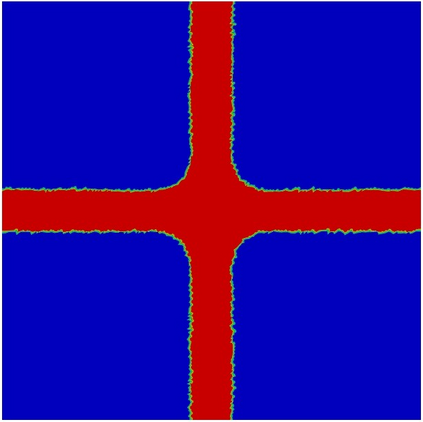
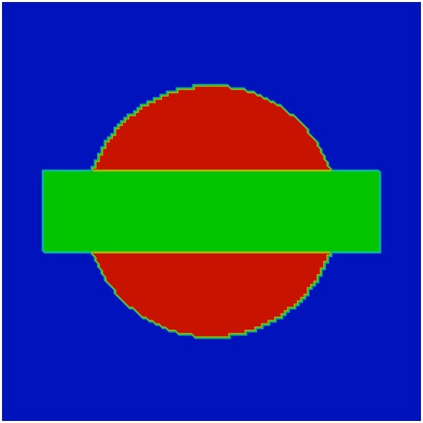
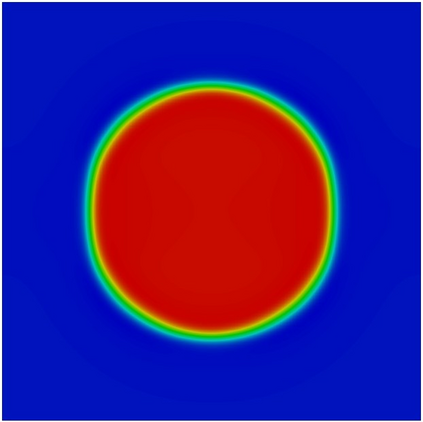
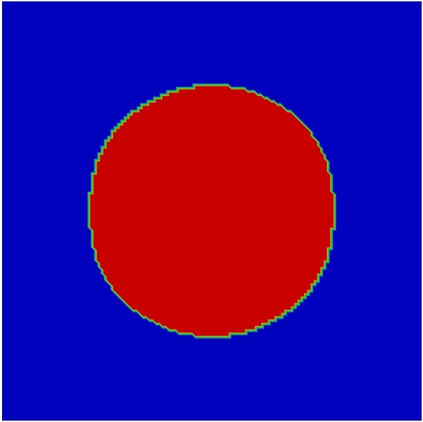
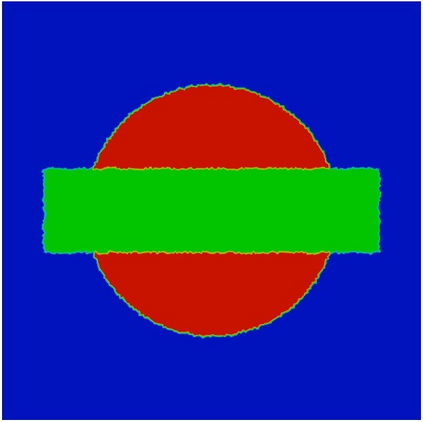
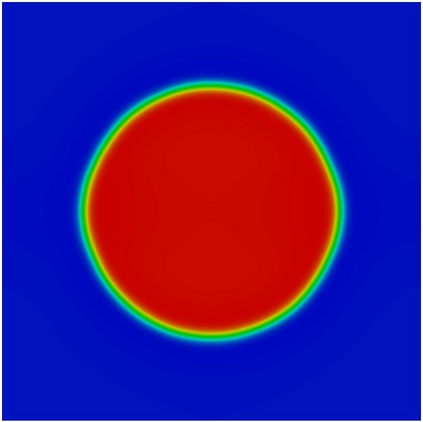
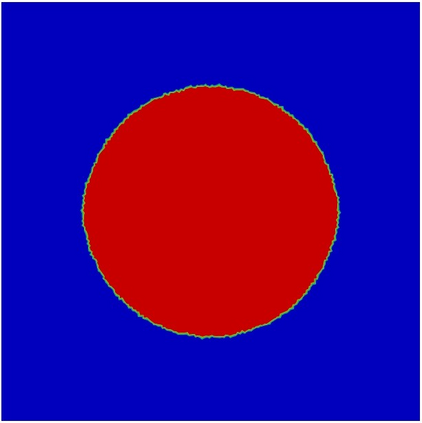
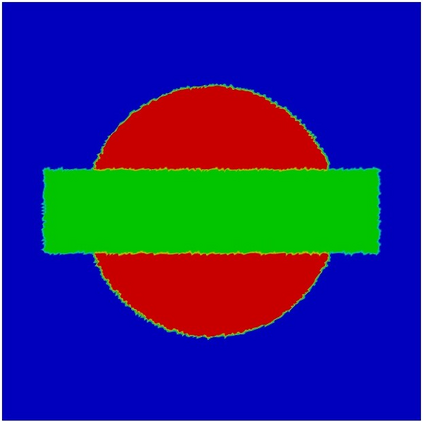
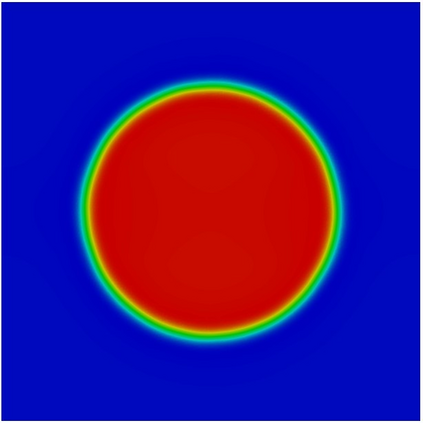
We consider the $C^1$-Virtual Element Method (VEM) for the conforming numerical approximation of some variants of the Cahn-Hilliard equation on polygonal meshes. In particular, we focus on the discretization of the advective Cahn-Hilliard problem and the Cahn-Hilliard inpainting problem. We present the numerical approximation and several numerical results to assess the efficacy of the proposed methodology.
翻译：我们考虑用1美元基本元素法(VEM)来计算多角藻的卡恩-希利亚德方程中某些变体的一致数字近似值,特别是侧重于对卡恩-希利亚德问题和卡恩-希利亚德涂漆问题的分解,我们提出数字近似值和若干数字结果,以评估拟议方法的功效。
相关内容
Arxiv
0+阅读 · 2022年4月18日
Arxiv
0+阅读 · 2022年4月15日
Arxiv
0+阅读 · 2022年4月15日
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2022年4月18日
Arxiv
0+阅读 · 2022年4月15日
Arxiv
0+阅读 · 2022年4月15日
微信扫码咨询专知VIP会员