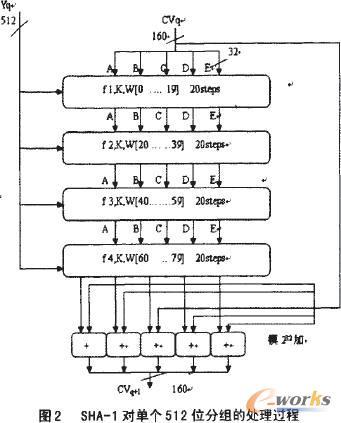
In this paper, we specify a class of mathematical problems, which we refer to as "Function Density Problems" (FDPs, in short), and point out novel connections of FDPs to the following two cryptographic topics; theoretical security evaluations of keyless hash functions (such as SHA-1), and constructions of provably secure pseudorandom generators (PRGs) with some enhanced security property introduced by Dubrov and Ishai [STOC 2006]. Our argument aims at proposing new theoretical frameworks for these topics (especially for the former) based on FDPs, rather than providing some concrete and practical results on the topics. We also give some examples of mathematical discussions on FDPs, which would be of independent interest from mathematical viewpoints. Finally, we discuss possible directions of future research on other cryptographic applications of FDPs and on mathematical studies on FDPs themselves.
翻译:在本文中,我们具体说明了一组数学问题,我们称之为“功能密度问题”,简要地说,我们指出FDP与以下两个加密专题的新联系;无关键散列功能(如SHA-1)的理论安全评估,以及Dubrov和Ishai介绍的、具有某种强化安全属性的、可探测安全的假冒原体(PRGs)的建造。我们的论点旨在根据FDP为这些专题(特别是前者)提出新的理论框架,而不是就这两个专题提供一些具体和实际的结果。我们还举了一些关于FDP的数学讨论的例子,从数学角度看,这些讨论将具有独立的兴趣。最后,我们讨论了未来研究FDP其他加密应用和FDP本身数学研究的可能方向。