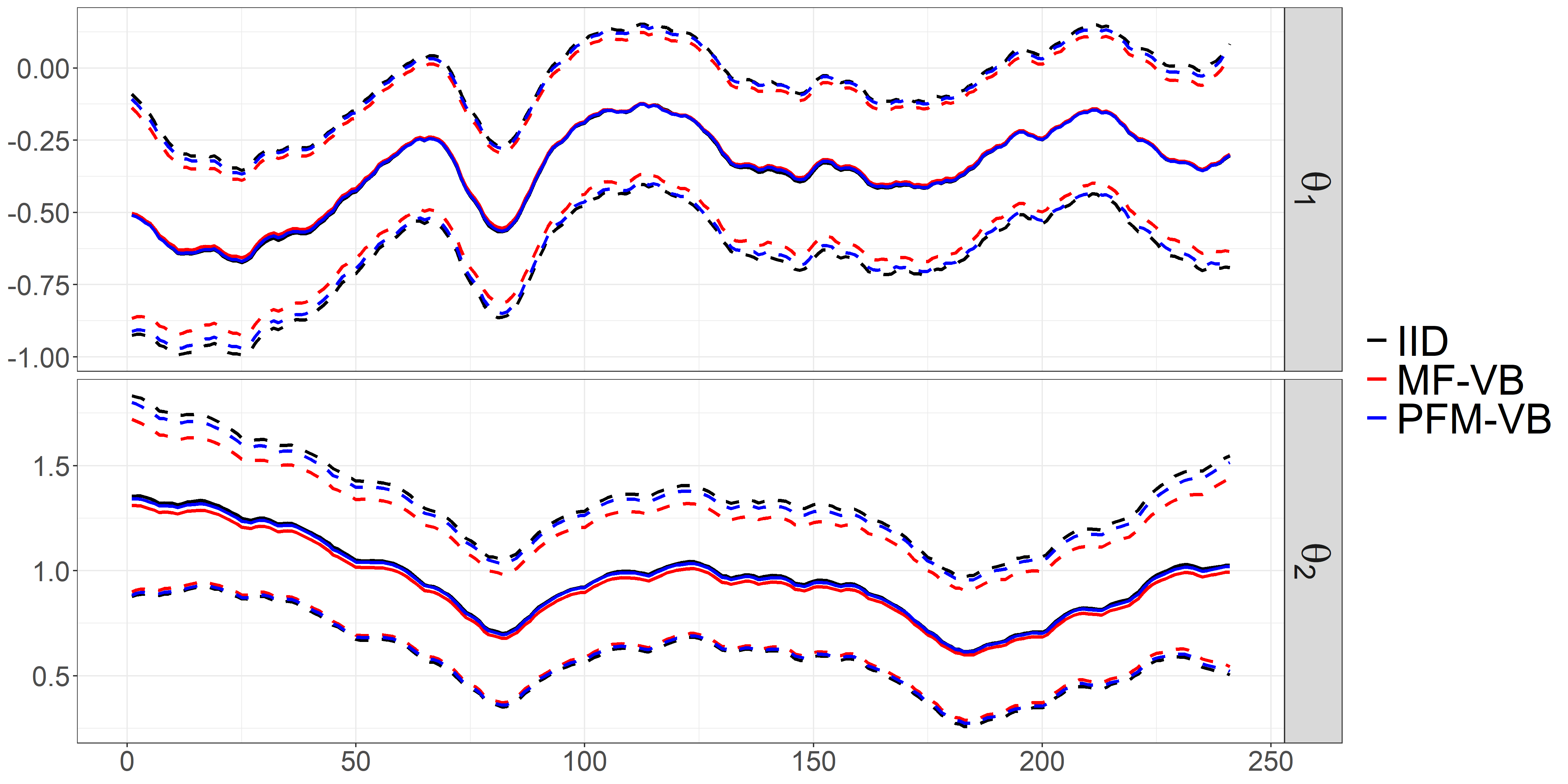
Recently, Fasano, Rebaudo, Durante and Petrone (2019) provided closed-form expressions for the filtering, predictive and smoothing distributions of multivariate dynamic probit models, leveraging on unified skew-normal distribution properties. This allows to develop algorithms to draw independent and identically distributed samples from such distributions, as well as sequential Monte Carlo procedures for the filtering and predictive distributions, allowing to overcome computational bottlenecks that may arise for large sample sizes. In this paper, we briefly review the above-mentioned closed-form expressions, mainly focusing on the smoothing distribution of the univariate dynamic probit. We develop a variational Bayes approach, extending the partially factorized mean-field variational approximation introduced by Fasano, Durante and Zanella (2019) for the static binary probit model to the dynamic setting. Results are shown for a financial application.
翻译:最近,Fasano、Rebaudo、Durante和Petrone (2019年) 提供了多种变式动态活性活性原体模型过滤、预测和平稳分布的闭式表达式(2019年), 借助于统一的Skew-正常分布特性, 从而开发了从这些分布中独立和相同分布的样本的算法, 以及随后的Monte Carlo过滤和预测分布程序, 从而可以克服大样本规模的计算瓶颈。 本文简要回顾了上述封闭式表达式, 重点是单体动态活性原体的平稳分布。 我们开发了一种变式贝斯方法, 将Fasano、 Durante和Zanella( 2019年) 引入的静态二元原原原原原原形模型的半成因位变化近似值扩展到动态环境。 我们为财务应用展示了结果 。