Private Information Retrieval from Colluding and Byzantine Servers with Binary Reed-Muller Codes

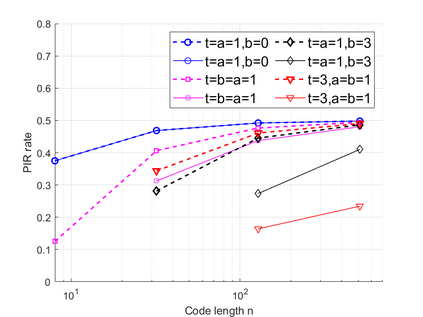
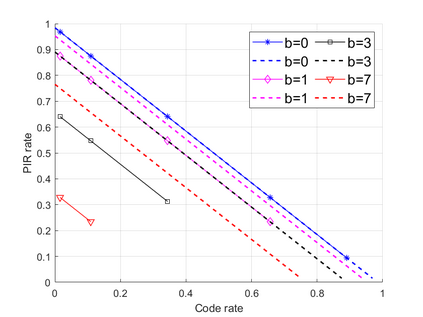
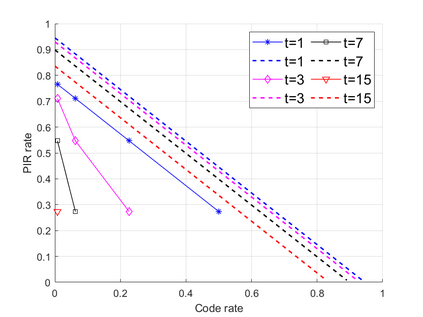
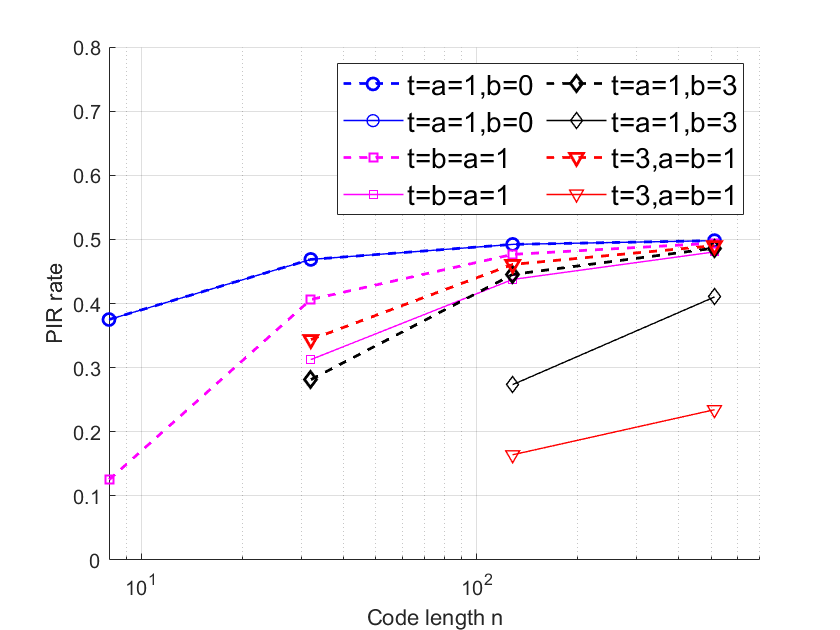
In this work, a flexible and robust private information retrieval (PIR) scheme based on binary non-maximum distance separable (non-MDS) codes is considered. This combines previous works on PIR schemes based on transitive non-MDS codes on one hand, and PIR from MDS-coded Byzantine and non-responsive servers on the other hand. More specifically, a PIR scheme employing binary Reed-Muller (RM) codes tolerant to colluding, Byzantine, and non-responsive servers is constructed, and bounds for the achievable rates are derived under certain conditions. The construction of such schemes turns out to be much more involved than for MDS codes. Namely, the binary query vectors have to be selected with great care to hit the desired information sets, which is technically challenging as will be shown.