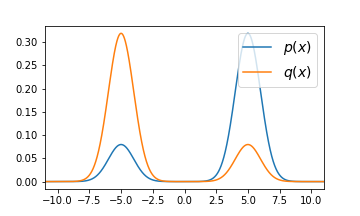
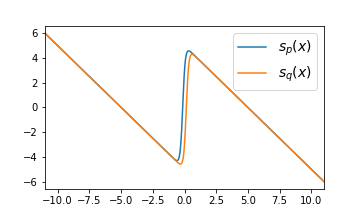
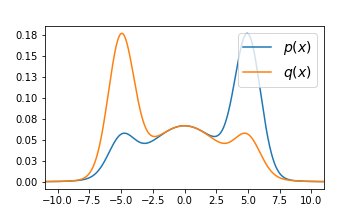
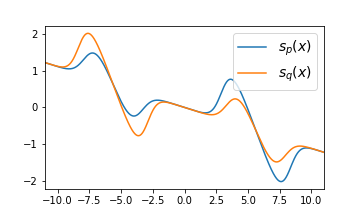
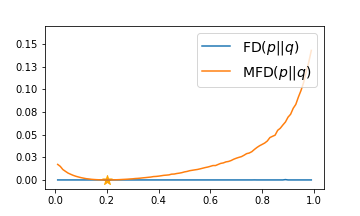
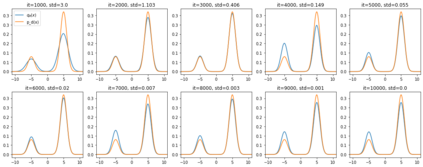
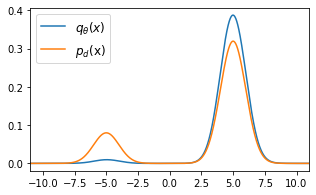
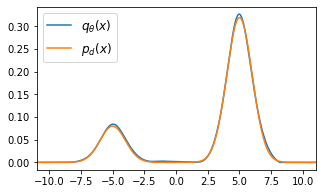
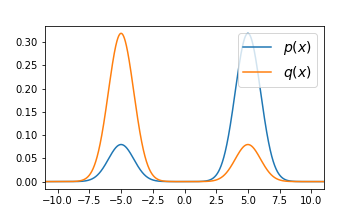
Score-based divergences have been widely used in machine learning and statistics applications. Despite their empirical success, a blindness problem has been observed when using these for multi-modal distributions. In this work, we discuss the blindness problem and propose a new family of divergences that can mitigate the blindness problem. We illustrate our proposed divergence in the context of density estimation and report improved performance compared to traditional approaches.
翻译:在机器学习和统计应用中广泛使用了基于分数的差异,尽管它们取得了经验性的成功,但在使用这些方法进行多模式分布时发现失明问题。在这项工作中,我们讨论了失明问题,提出了一个新的差异体系,以缓解失明问题。我们说明了在密度估计方面提出的差异,并报告了与传统方法相比业绩有所改善的情况。
相关内容
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2022年10月26日
Arxiv
1+阅读 · 2022年10月21日