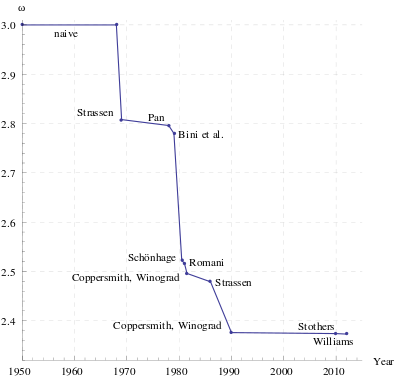
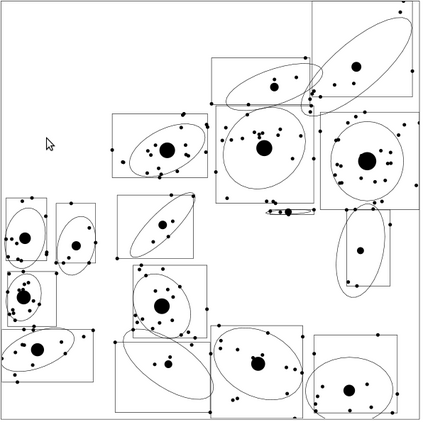
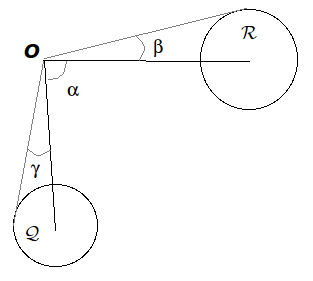
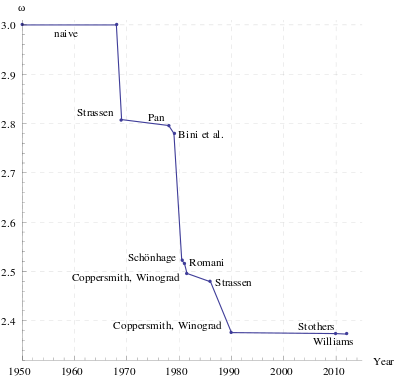
Herein we explore a dual tree algorithm for matrix multiplication of $A\in \mathbb{R}^{M\times D}$ and $B\in\mathbb{R}^{D\times N}$, very narrowly effective if the normalized rows of $A$ and columns of $B$, treated as vectors in $\mathbb{R}^{D}$, fall into clusters of order proportionate to $\Omega(D^{\tau})$ with radii less than $\arcsin(\epsilon/\sqrt{2})$ on the surface of the unit $D$-ball. The algorithm leverages a pruning rule necessary to guarantee $\epsilon$ precision proportionate to vector magnitude products in the resultant matrix. \textit{ Unfortunately, if the rows and columns are uniformly distributed on the surface of the unit $D$-ball, then the expected points per required cluster approaches zero exponentially fast in $D$; thus, the approach requires a great deal of work to pass muster.}
翻译:在这里,我们探索一个双树算法,用于在单位表面的 $A\ in\ mathb{R\\\M\timeD}D$和$B\in\mathb{R\D\timenN}$B\\\mathb{R\D\time$的矩阵乘法,如果以$mathb{R\D}$作为矢量处理的归一行和列为$B$B$,作为矢量的矢量的矢量,被划成与单位表面的 $Omega(D$D>Tau} 成正比的一组,而光度小于$\arcsin(\epsilon/\\sqrt{2}$($D$D$) 和$B$B$的基质值,则该双树算法将非常狭隘有效。因此,该算法将运用一条必要的修剪规则来保证在结果矩阵中保证 $\ epersillon ex un 精确度与矢量产品成比例的矢量值产品。\ textititititititle{如果行和柱在单位表面上统一分布在单位表面分配 $D$$D$- balls,那么,那么,那么,那么,那么,那么, 需要每组的预期的点点点点每组接近零xxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxx