Energy stability of Strang splitting for vector-valued and matrix-valued Allen-Cahn equations

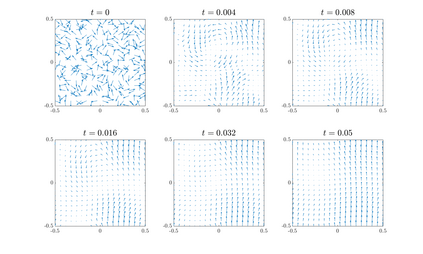
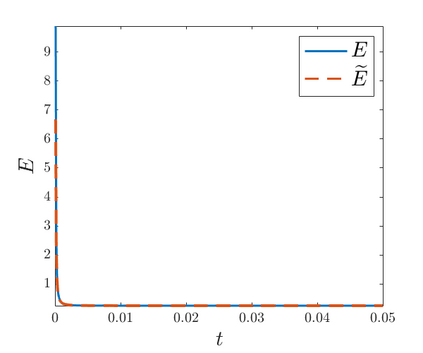
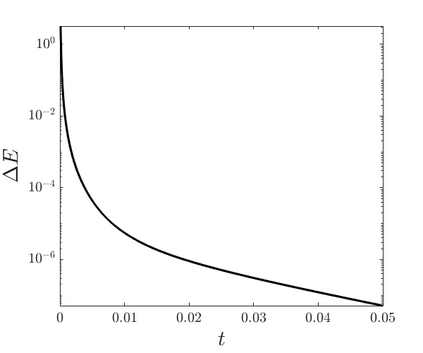
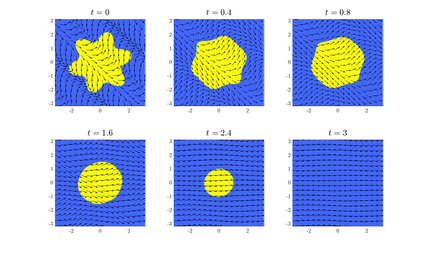
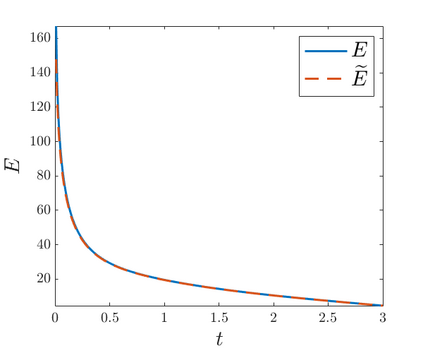
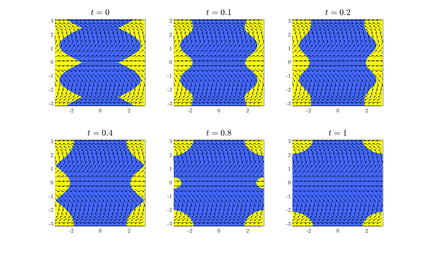
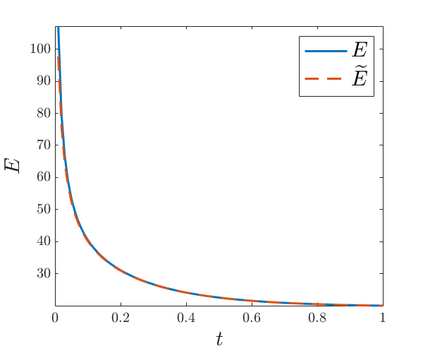
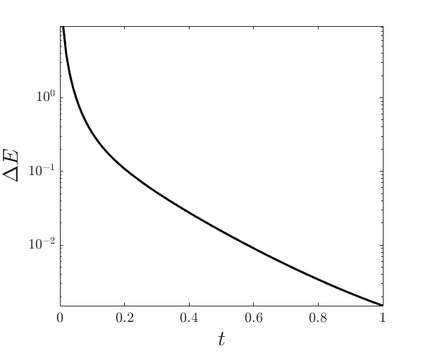
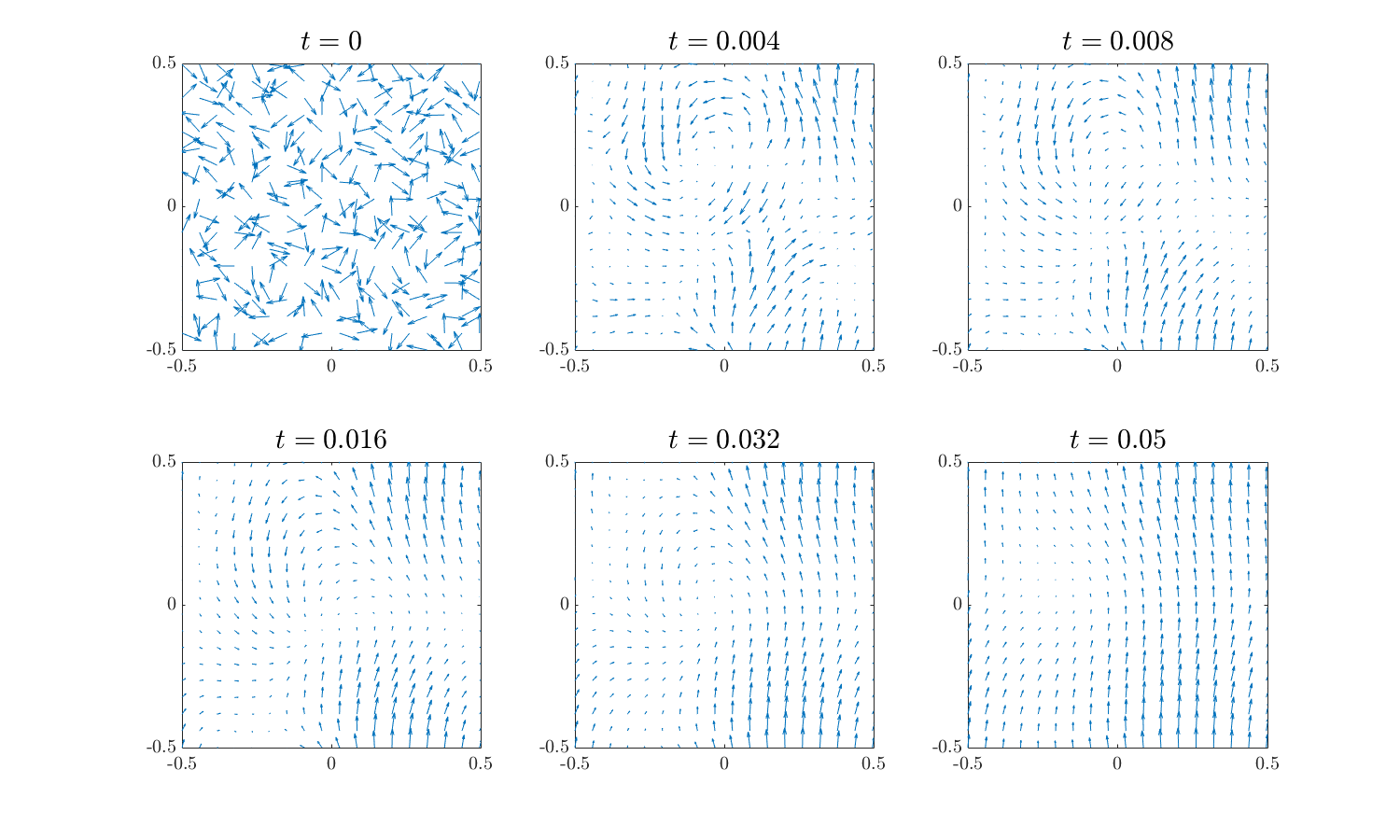
We consider the second-order in time Strang-splitting approximation for vector-valued and matrix-valued Allen-Cahn equations. Both the linear propagator and the nonlinear propagator are computed explicitly. For the vector-valued case, we prove the maximum principle and unconditional energy dissipation for a judiciously modified energy functional. The modified energy functional is close to the classical energy up to $\mathcal O(\tau)$ where $\tau$ is the splitting step. For the matrix-valued case, we prove a sharp maximum principle in the matrix Frobenius norm. We show modified energy dissipation under very mild splitting step constraints. We exhibit several numerical examples to show the efficiency of the method as well as the sharpness of the results.