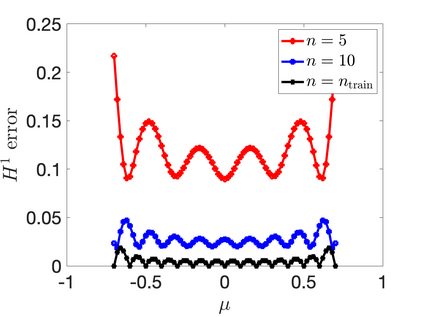
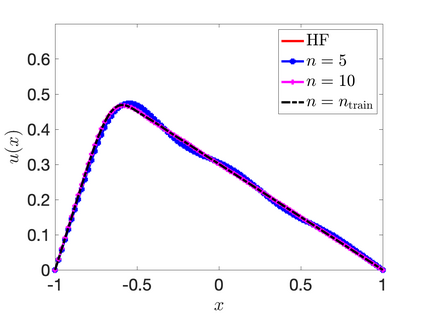
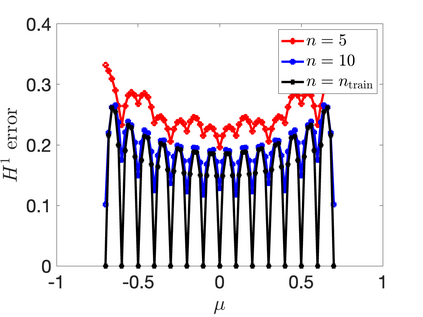
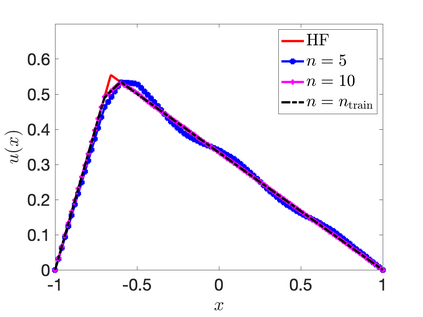
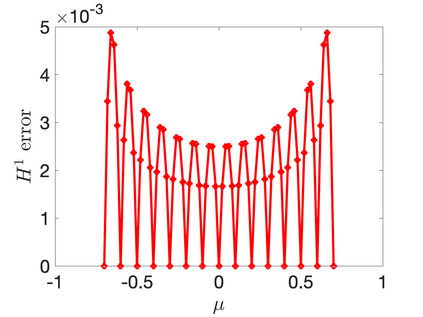
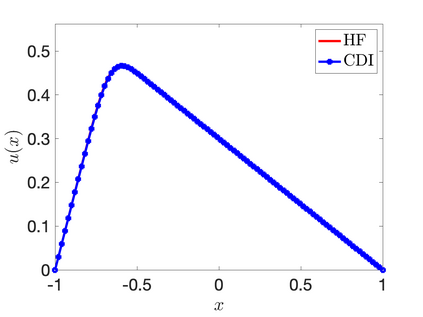
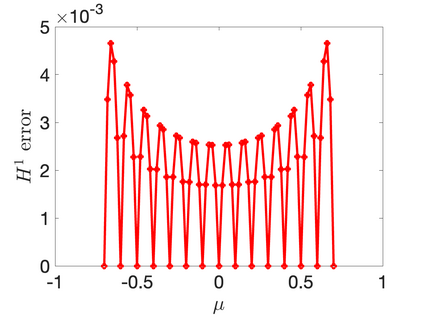
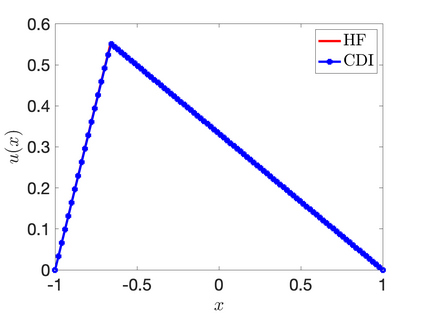
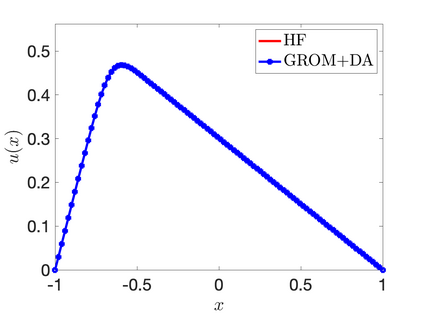
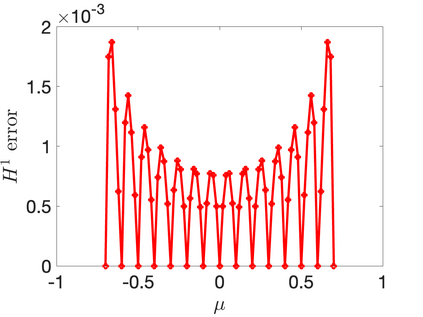
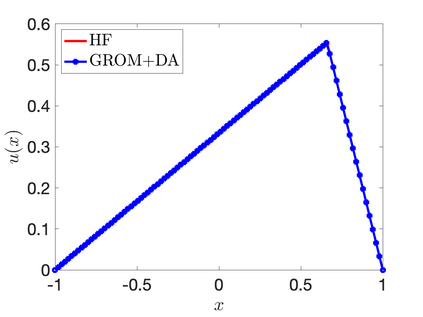
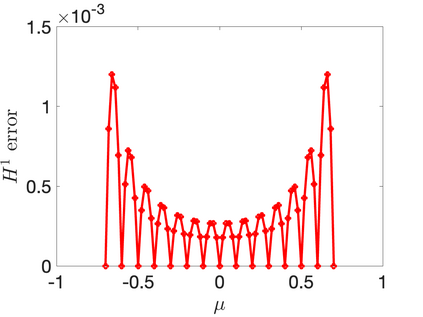
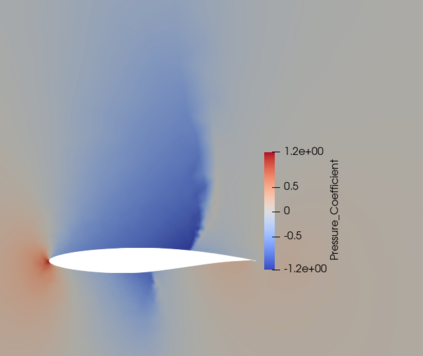
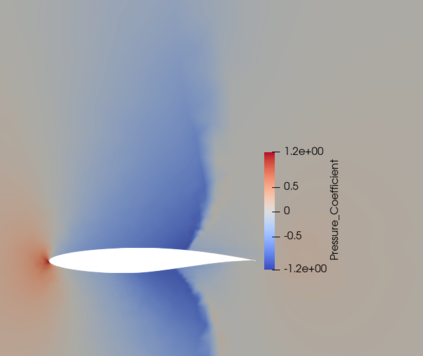
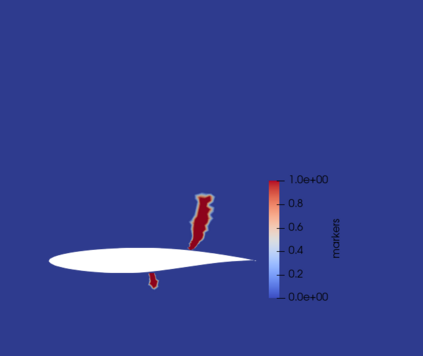
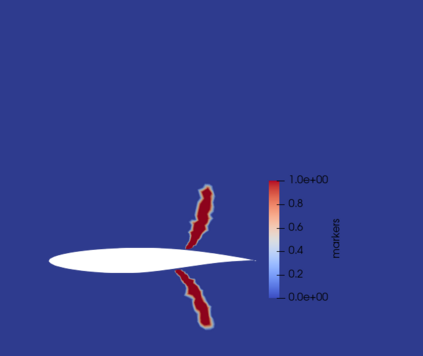
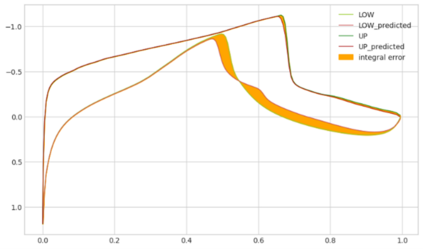
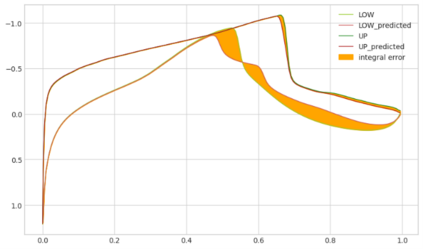
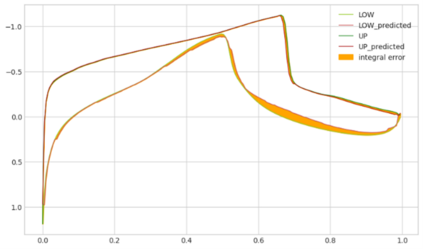
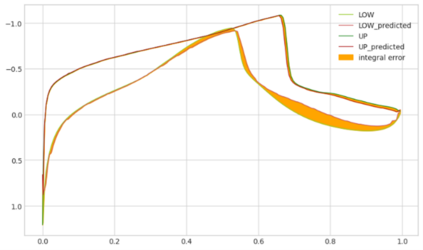
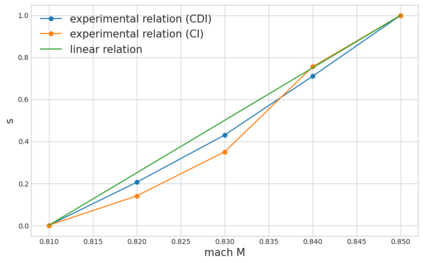
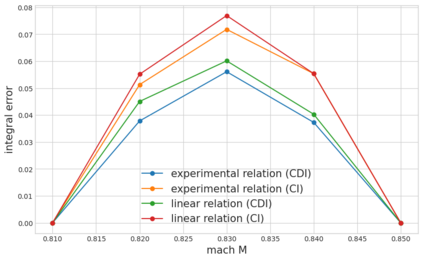
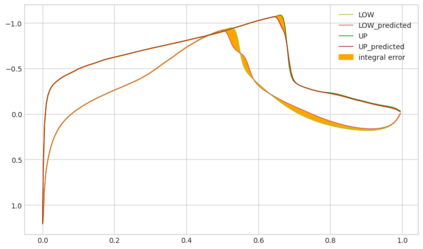
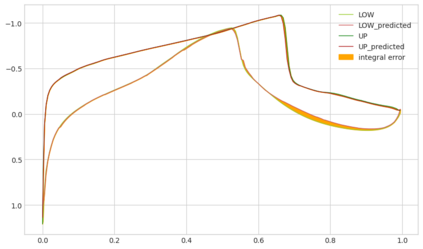
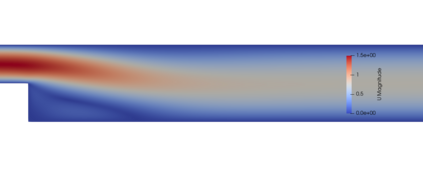
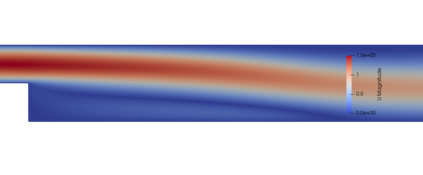
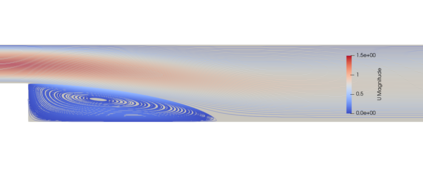
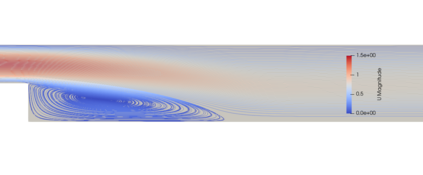
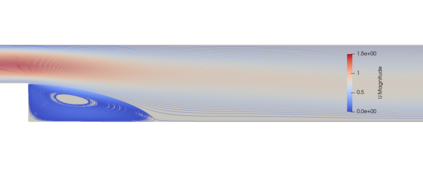
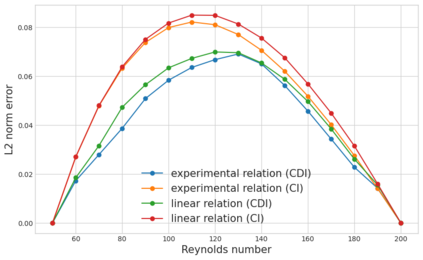
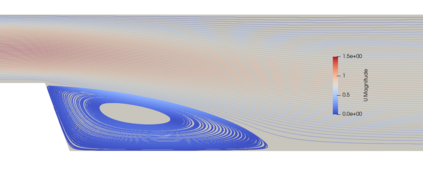
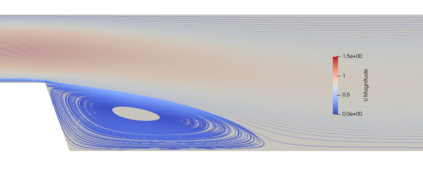
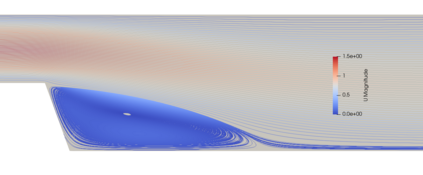
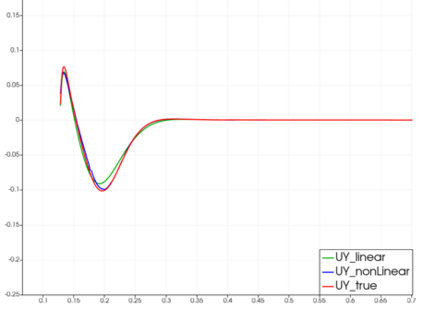
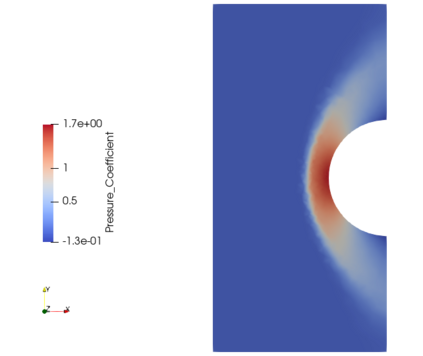
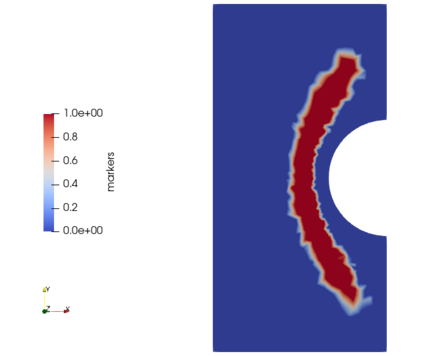
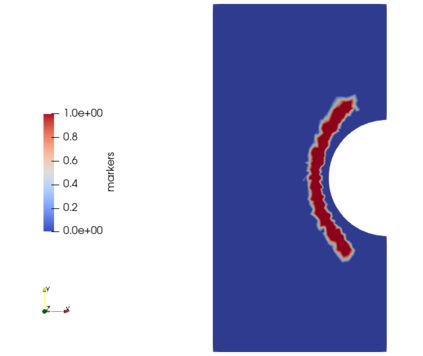
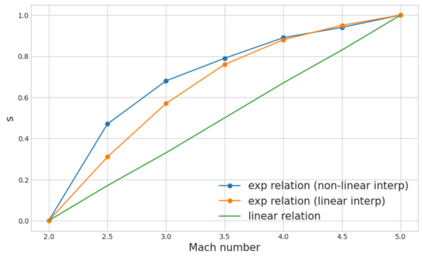
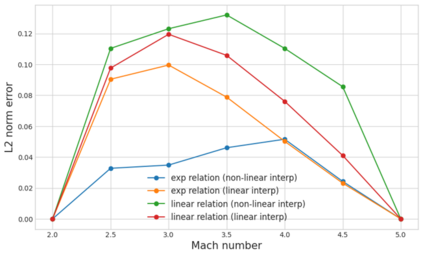
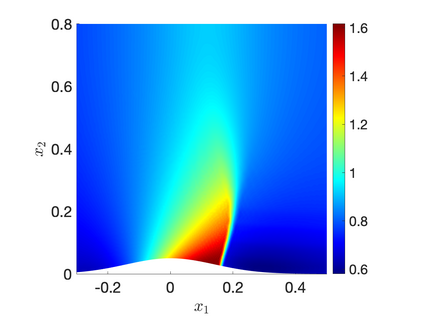
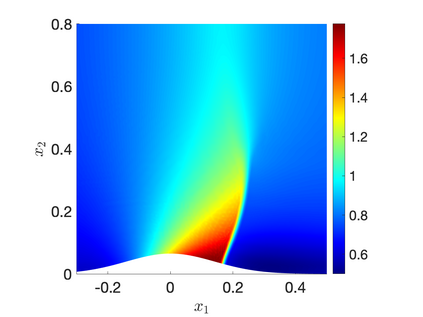
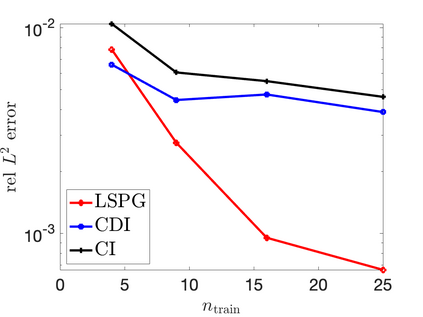
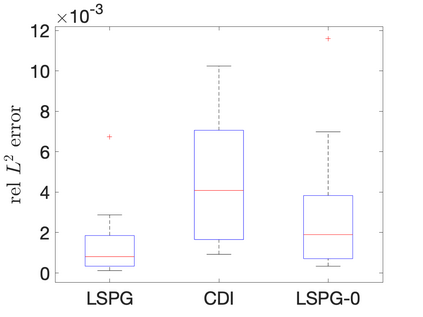
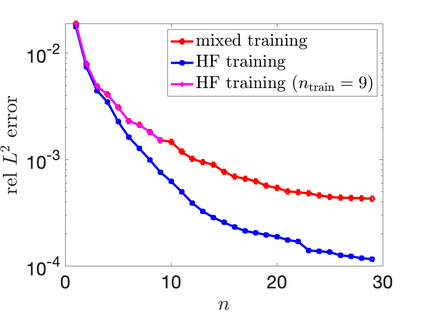
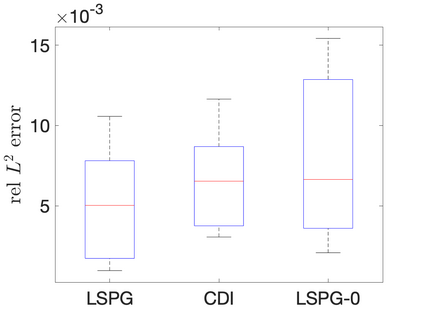
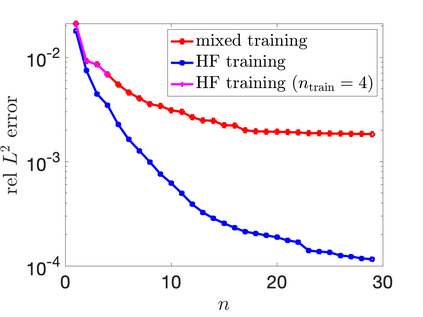
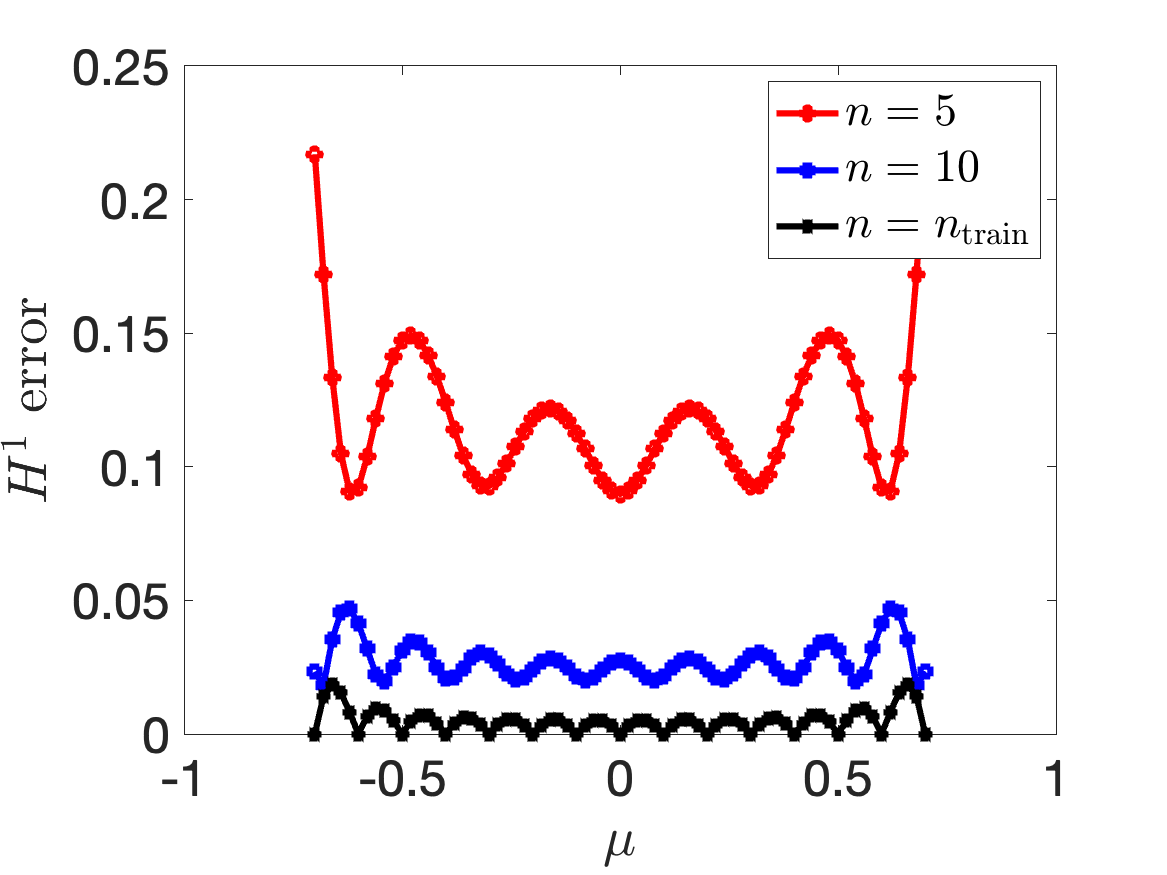
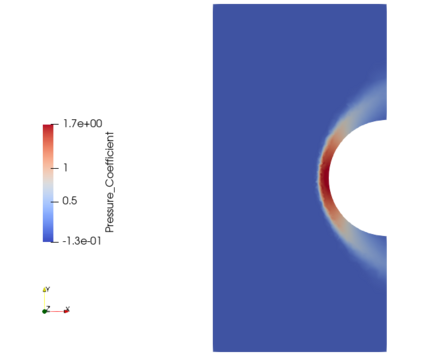We present a nonlinear interpolation technique for parametric fields that exploits optimal transportation of coherent structures of the solution to achieve accurate performance. The approach generalizes the nonlinear interpolation procedure introduced in [Iollo, Taddei, J. Comput. Phys., 2022] to multi-dimensional parameter domains and to datasets of several snapshots. Given a library of high-fidelity simulations, we rely on a scalar testing function and on a point set registration method to identify coherent structures of the solution field in the form of sorted point clouds. Given a new parameter value, we exploit a regression method to predict the new point cloud; then, we resort to a boundary-aware registration technique to define bijective mappings that deform the new point cloud into the point clouds of the neighboring elements of the dataset, while preserving the boundary of the domain; finally, we define the estimate as a weighted combination of modes obtained by composing the neighboring snapshots with the previously-built mappings. We present several numerical examples for compressible and incompressible, viscous and inviscid flows to demonstrate the accuracy of the method. Furthermore, we employ the nonlinear interpolation procedure to augment the dataset of simulations for linear-subspace projection-based model reduction: our data augmentation procedure is designed to reduce offline costs -- which are dominated by snapshot generation -- of model reduction techniques for nonlinear advection-dominated problems.
翻译:暂无翻译