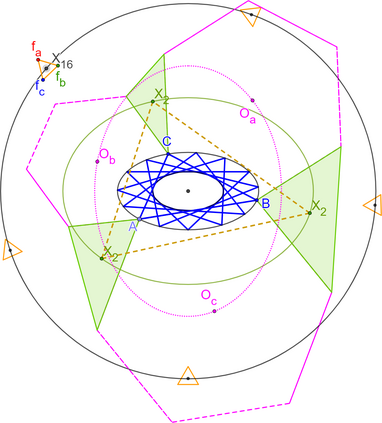
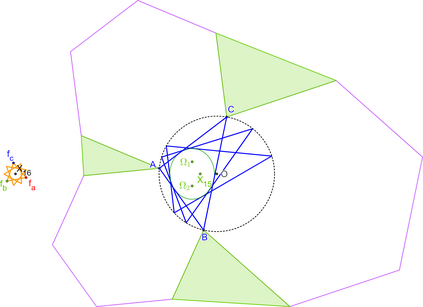
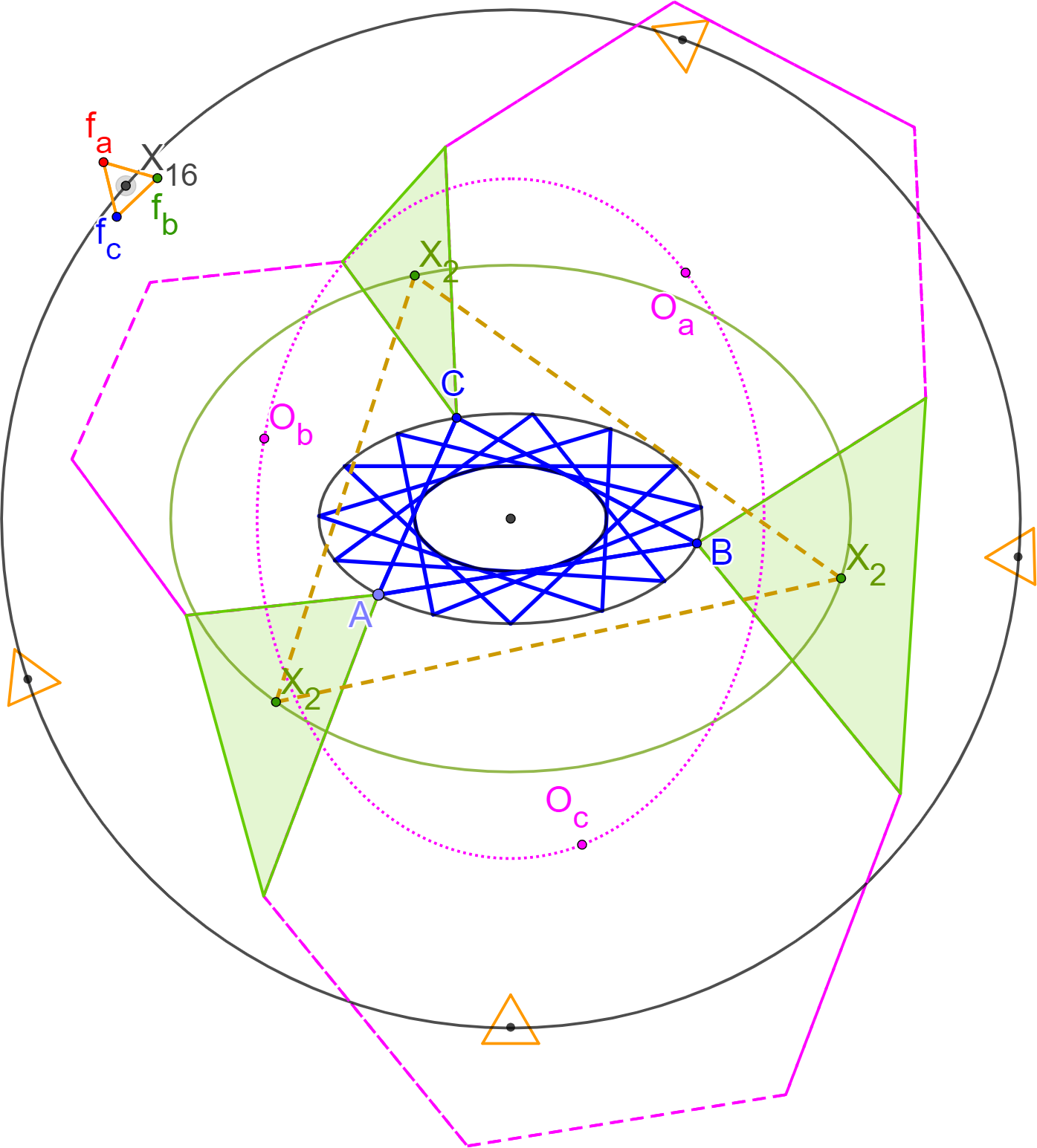
If one erects regular hexagons upon the sides of a triangle $T$, several surprising properties emerge, including: (i) the triangles which flank said hexagons have an isodynamic point common with $T$, (ii) the construction can be extended iteratively, forming an infinite grid of regular hexagons and flank triangles, (iii) a web of confocal parabolas with only three distinct foci interweaves the vertices of hexagons in the grid. Finally, (iv) said foci are the vertices of an equilateral triangle.
翻译:如果一个人在三角形的两侧架设正常的六边形,就会出现若干惊人的特性,包括:(一) 侧翼的三角形,上面说六边形有一个与美元相同的等动力点,(二) 构造可以迭代延伸,形成一个由普通六边形和侧翼三角形组成的无限网格,(三) 圆形的圆柱形网,只有三个不同的方形交织,在网格中将六边形的顶端交织在一起。最后,(四) 上面说方形是等边三角形的顶端。
相关内容
专知会员服务
78+阅读 · 2022年3月15日
Arxiv
0+阅读 · 2022年7月14日
Arxiv
0+阅读 · 2022年7月13日
Arxiv
0+阅读 · 2022年7月12日