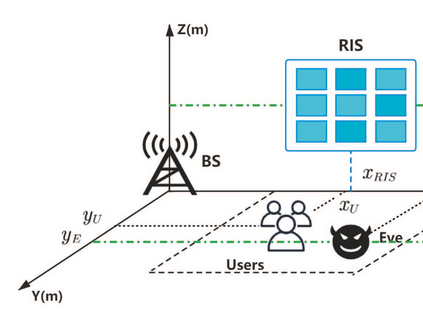
This paper investigates reconfigurable intelligent surface (RIS)-assisted secure multiuser communication systems subject to hardware impairments (HIs). We jointly optimize the beamforming vectors at the base station (BS) and the phase shifts of the reflecting elements at the RIS so as to maximize the weighted minimum secrecy rate (WMSR), subject to both transmission power constraints at the BS and unit-modulus constraints at the RIS. To address the formulated optimization problem, we first decouple it into two tractable subproblems and then use the block coordinate descent (BCD) method to alternately optimize the subproblems. Two different methods are proposed to solve the two obtained subproblems. The first method transforms each subproblem into a second order cone programming (SOCP) problem, which can be directly solved using CVX. The second method leverages the Minorization- Maximization (MM) algorithm. Specifically, we first derive a concave approximation function, which is a lower bound of the original objective function, and then the two subproblems are transformed into two simple surrogate problems with closedform solutions. Simulation results verify the performance gains of the proposed robust transmission method over existing nonrobust designs. In addition, the MM algorithm is shown to have much lower complexity than the SOCP-based algorithm.
翻译:本文调查了可重新配置的智能表面(RIS)协助的安全多用户通信系统,这些系统受到硬件缺陷的影响。我们共同优化了基站(BS)的波形矢量,优化了该基站(BS)的反射矢量的分阶段转换,以便最大限度地实现加权最低保密率(WMSR),这取决于BS的传输功率限制和RIS的单位模量限制。为了解决开发的优化问题,我们首先将它分解为两个可移植的子问题,然后使用区块协调下降法(BCD)来替代优化子问题。我们提出了两种不同的方法来解决获得的两个子问题。第一种方法将每个子问题转化为第二个阶式最低保密率(WMSR)问题,这些问题可以通过CVX直接解决。第二种方法利用微小化最大化算法。具体地说,我们首先得出一个基于同系的近似近似近比值函数,然后将两个子相向下优化。两种子问题将解决两个小问题的方法转换成两个获得的子问题。第一个方法将每个子问题转换成两个简单化的变式的变式变式,而后算算算法则显示为不为不甚高的系统变的系统变的系统变式方法。