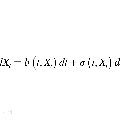带脉冲的随机生物种群模型动力学性质研究

项目名称： 带脉冲的随机生物种群模型动力学性质研究

项目编号： No.11526192

项目类型： 专项基金项目

立项/批准年度： 2016

项目学科： 数理科学和化学

项目作者： 吴瑞华

作者单位： 中国石油大学（华东）

项目金额： 3万元

中文摘要： 在现实生活中，脉冲和随机现象处处存在，生物种群的生长不可避免的会受到随机噪音和脉冲的影响。因此，在生物种群的研究中，考虑脉冲和随机因素的影响是必要的。本项目拟研究带脉冲扰动的随机种群系统的动力学性质，具体内容包括：(1) 把脉冲耦合到随机种群模型中，建立更加符合实际的随机模型。（2) 提出恰当的随机脉冲微分方程解的定义，进而研究其正解的渐近性质，并给出数值模拟。通过对上述问题的研究，能够揭示出脉冲和随机干扰对种群的生存和灭绝阈值、持久性的影响，进一步为一些重要的实际问题的解决提供建设性建议。

中文关键词： 随机微分方程；随机扰动；生存性；灭绝性；周期解

英文摘要： In the real world, stochastic phenomena exist everywhere, and species are inevitably affected by the environmental noise. so it is necessary to consider the effects of random perturbations and impulse on the population systems. This project will focus on studying the persistence of stochastic population system: (1) The three noise (the white noise, telegraph noise and jumping noise) will be considered in these models at the same time, and then more suitable models can be established. (2) We attempt to propose the definition of stochastic permanence and study the stochastic permanence and extiction of our models. Moreover, we give numerical simulations for our results. This project is expected to reveal the effects of stochastic factors on the asymptotic properties of the species, while it will offer some theoretical basis and constructive suggestions for some practical problems, such as how to develop better population protection policy and how to make all kinds of species harmony existence.

英文关键词： stochastic differential equations；random perturbations；persistence；extinction；periodic sulutions