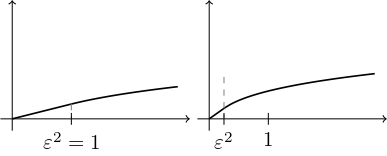
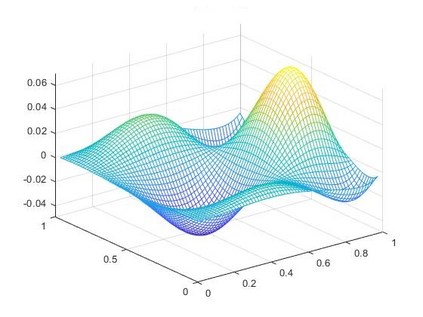
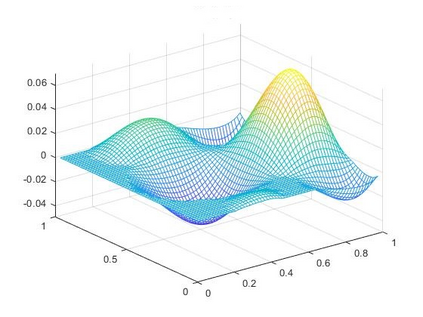
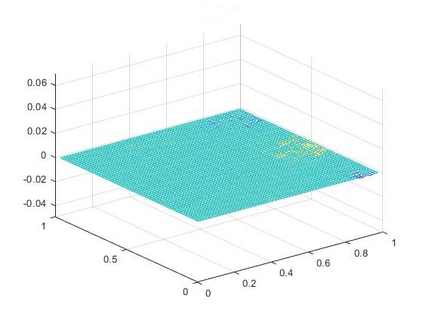
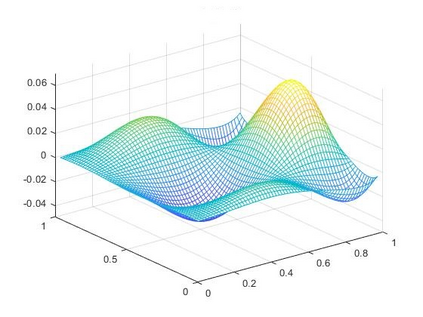
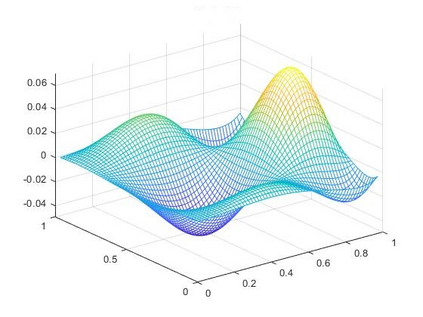
Flexible sparsity regularization means stably approximating sparse solutions of operator equations by using coefficient-dependent penalizations. We propose and analyse a general nonconvex approach in this respect, from both theoretical and numerical perspectives. Namely, we show convergence of the regularization method and establish convergence properties of a couple of majorization approaches for the associated nonconvex problems. We also test a monotone algorithm for an academic example where the operator is an $M$ matrix, and on a time-dependent optimal control problem, pointing out the advantages of employing variable penalties over a fixed penalty.
翻译:灵活宽度规范化意味着通过使用以系数为根据的处罚,稳定地接近操作方程式的少数解决办法。我们从理论和数字角度提出并分析这方面的一般非曲线方法。我们从理论和数字角度展示了规范化方法的趋同性,并为相关的非曲线问题确定了若干主要方法的趋同性。我们还测试了一个学术实例的单调算法,即操作方为1美元矩阵,并且存在一个取决于时间的最佳控制问题,指出对固定刑罚采用可变处罚的优点。