Computational framework for complex flow and transport in heterogeneous porous media

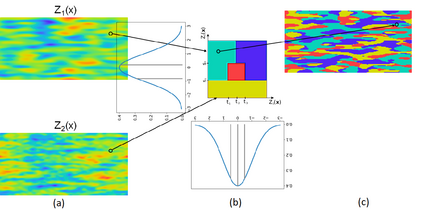
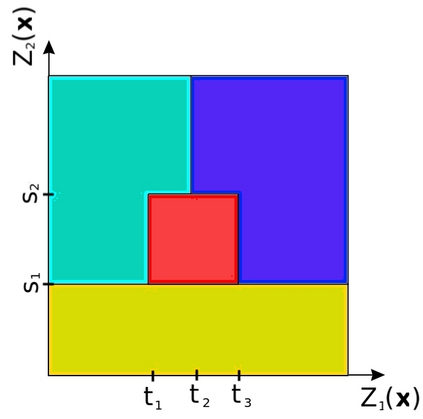
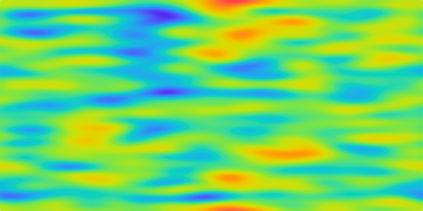
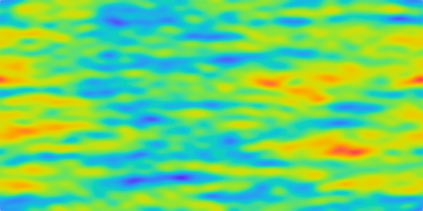
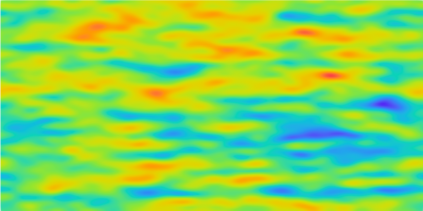
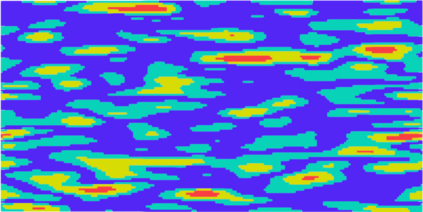
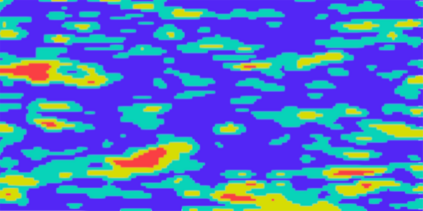
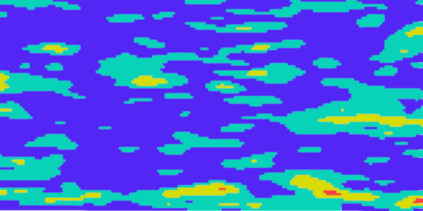
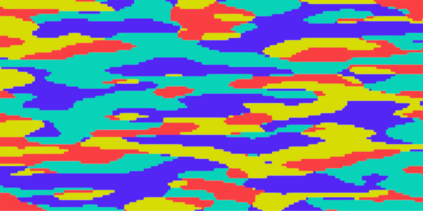
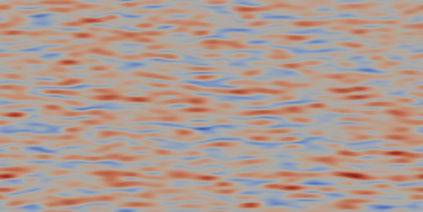
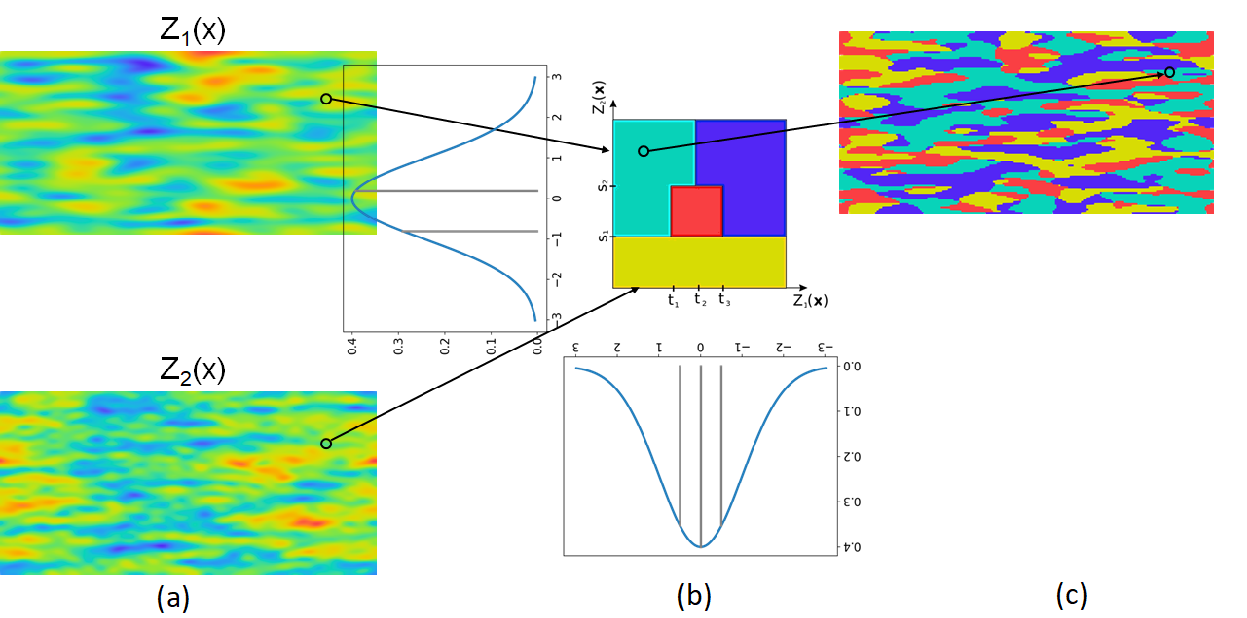
We present a flexible scalable open-source computational framework, named SECUReFoam, based on the finite-volume library OpenFOAM(R), for flow and transport problems in highly heterogeneous geological media and other porous materials. The framework combines geostatistical pre- and post-processing tools with specialised Partial Differential Equations solvers. Random fields, for permeability and other physical properties, are generated by means of continuous or thresholded Gaussian random fields with various covariance/variogram functions. The generation process is based on an explicit spectral Fourier decomposition of the field which, although more computationally intensive than Fast Fourier Transform methods, allows a more flexible choice of statistical parameters and can be used for general geometries and grids. Flow and transport equations are solved for single-phase and variable density problems, with and without the Boussinesq approximation, and for a wide range of density, viscosity, and dispersion models, including dual-continuum (dual permeability or dual porosity) formulations. The mathematical models are here presented in details and the numerical strategies to deal with heterogeneities, equation coupling, and boundary conditions are discussed and benchmarked for the heterogeneous Henry and Horton-Rodgers-Lapwood problems, and other test cases. We show that our framework is capable of dealing with large permeability variances, viscous instabilities, and large-scale three-dimensional transport problems.