Enabling Non-Linear Quantum Operations through Variational Quantum Splines

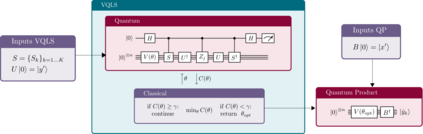
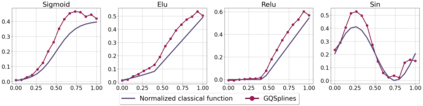
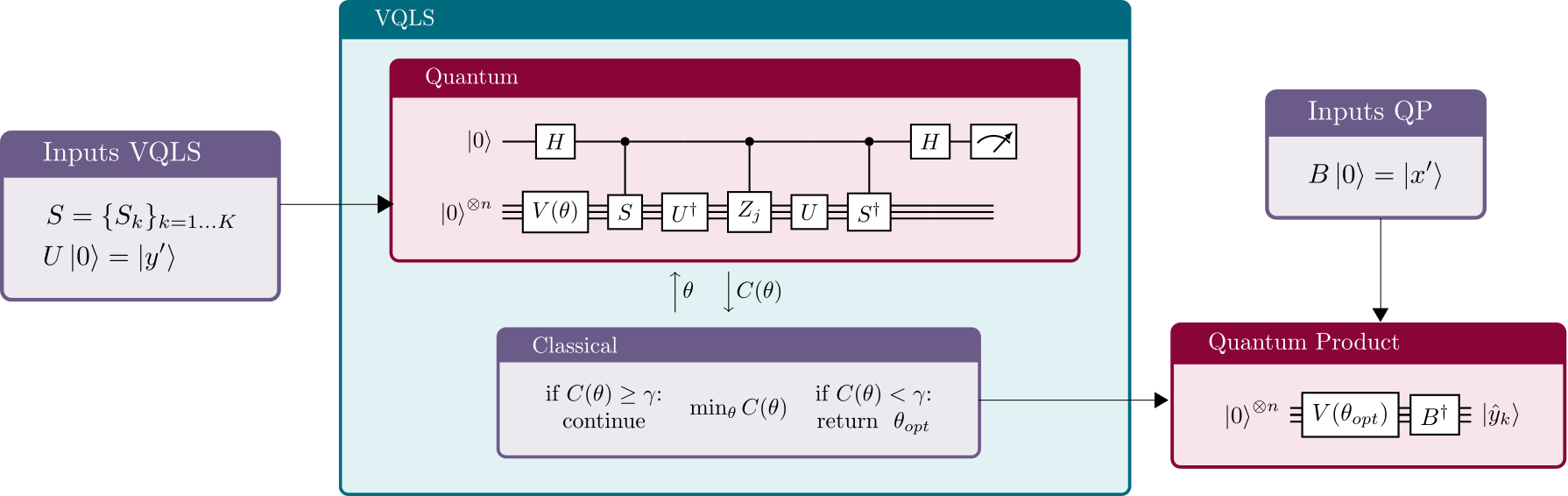
The postulates of quantum mechanics impose only unitary transformations on quantum states, which is a severe limitation for quantum machine learning algorithms. Quantum Splines (QSplines) have recently been proposed to approximate quantum activation functions to introduce non-linearity in quantum algorithms. However, QSplines make use of the HHL as a subroutine and require a fault-tolerant quantum computer to be correctly implemented. This work proposes the Generalised QSplines (GQSplines), a novel method for approximating non-linear quantum activation functions using hybrid quantum-classical computation. The GQSplines overcome the highly demanding requirements of the original QSplines in terms of quantum hardware and can be implemented using near-term quantum computers. Furthermore, the proposed method relies on a flexible problem representation for non-linear approximation and it is suitable to be embedded in existing quantum neural network architectures. In addition, we provide a practical implementation of GQSplines using Pennylane and show that our model outperforms the original QSplines in terms of quality of fitting.