Generalized perron roots and solvability of the absolute value equation

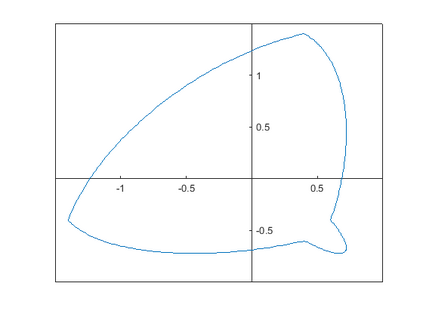
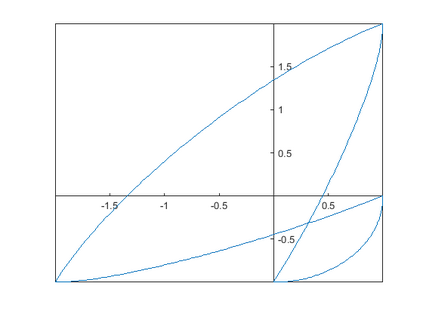
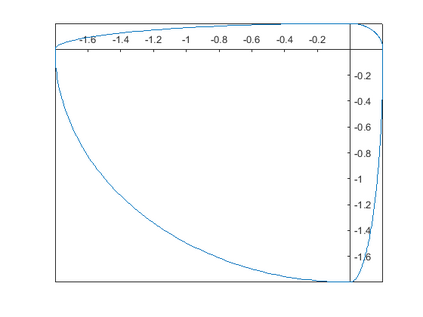
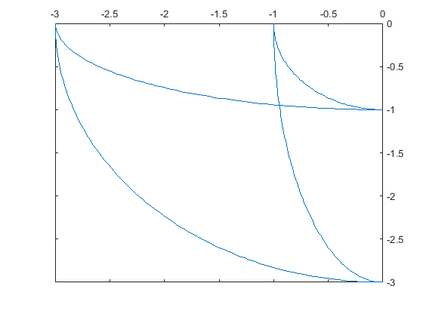
Let $A$ be a real $(n\times n)$-matrix. The piecewise linear equation system $z-A\vert z\vert =b$ is called an absolute value equation (AVE). It is well known to be uniquely solvable for all $b\in\mathbb R^n$ if and only if a quantity called the sign-real spectral radius of $A$ is smaller than one. We construct a quantity similar to the sign-real spectral radius that we call the aligning spectral radius $\rho^a$ of $A$. We prove that the AVE has mapping degree $1$ and thus an odd number of solutions for all $b\in\mathbb R^n$ if the aligning spectral radius of $A$ is smaller than one. Under mild genericity assumptions on $A$ we also manage to prove a converse result. Structural properties of the aligning spectral radius are investigated. Due to the equivalence of the AVE to the linear complementarity problem, a side effect of our investigation are new sufficient and necessary conditions for $Q$-matrices.