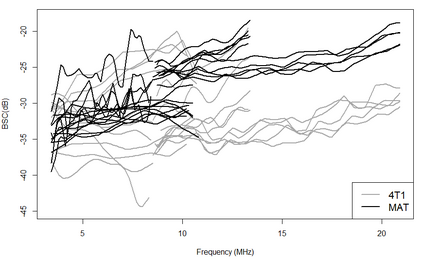
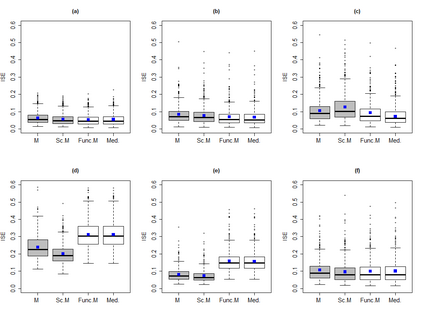
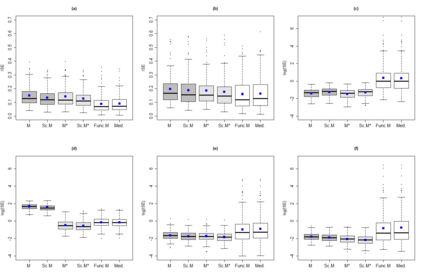
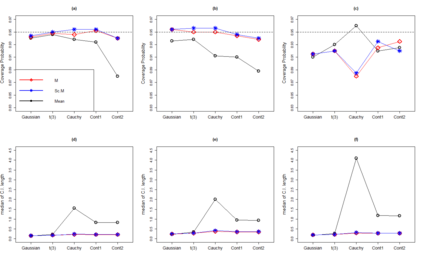
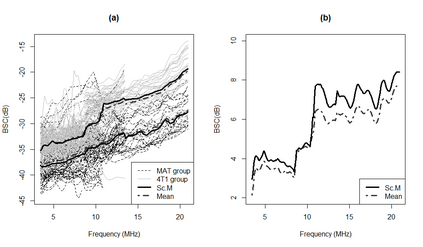
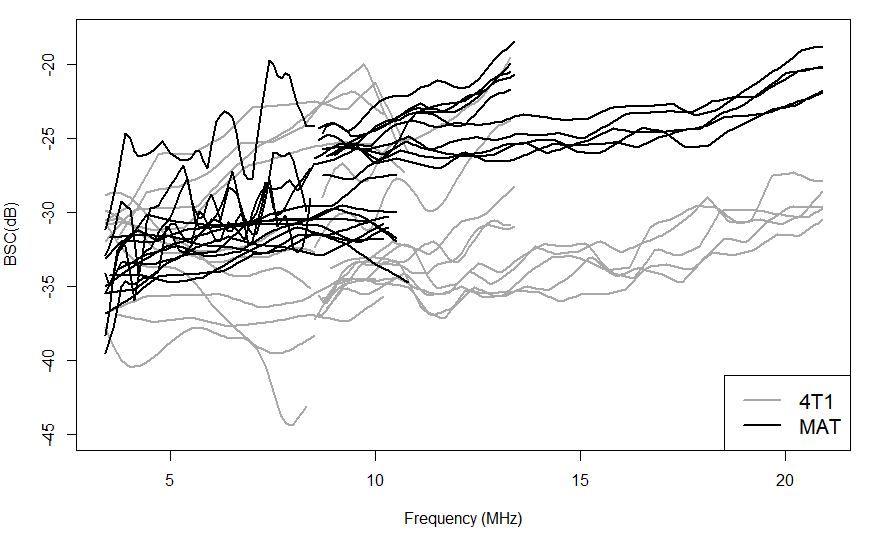
Irregular functional data in which densely sampled curves are observed over different ranges pose a challenge for modeling and inference, and sensitivity to outlier curves is a concern in applications. Motivated by applications in quantitative ultrasound signal analysis, this paper investigates a class of robust M-estimators for partially observed functional data including functional location and quantile estimators. Consistency of the estimators is established under general conditions on the partial observation process. Under smoothness conditions on the class of M-estimators, asymptotic Gaussian process approximations are established and used for large sample inference. The large sample approximations justify a bootstrap approximation for robust inferences about the functional response process. The performance is demonstrated in simulations and in the analysis of irregular functional data from quantitative ultrasound analysis.
翻译:由于应用定量超声波信号分析,本文件对包括功能位置和量度估计器在内的部分观测功能数据进行了一组稳健的M-测算器,在部分观察过程的一般条件下确定了测算器的连贯性。在测算器类的平稳条件下,确定并使用无症状高斯过程近似值进行大样本推断。大量采样近似值证明对功能反应过程进行稳健推断的理由。在模拟和分析定量超声波分析的不规则功能数据时,表现表现表现在模拟中和对定量超声波分析的不规则功能数据的分析中。