Exponential convergence of perfectly matched layers for scattering problems with periodic surfaces

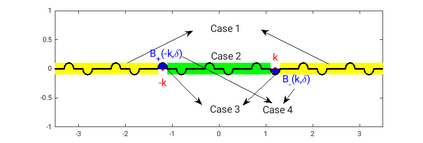
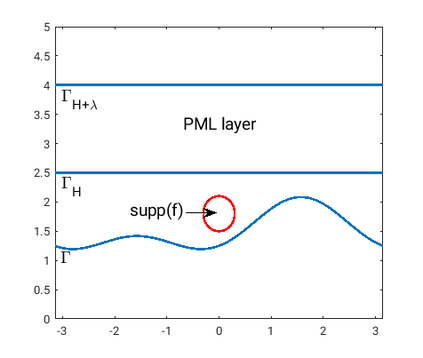
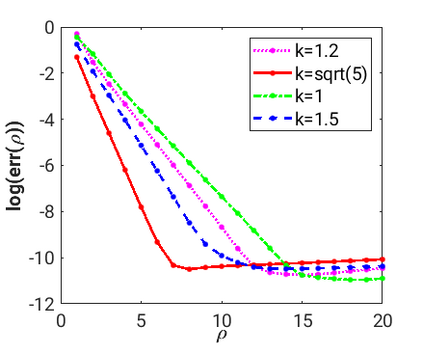
The main task in this paper is to prove that the perfectly matched layers (PML) method converges exponentially with respect to the PML parameter, for scattering problems with periodic surfaces. In [5], a linear convergence is proved for the PML method for scattering problems with rough surfaces. At the end of that paper, three important questions are asked, and the third question is if exponential convergence holds locally. In our paper, we answer this question for a special case, which is scattering problems with periodic surfaces. The result can also be easily extended to locally perturbed periodic surfaces or periodic layers. Due to technical reasons, we have to exclude all the half integer valued wavenumbers. The main idea of the proof is to apply the Floquet-Bloch transform to write the problem into an equivalent family of quasi-periodic problems, and then study the analytic extension of the quasi-periodic problems with respect to the Floquet-Bloch parameters. Then the Cauchy integral formula is applied for piecewise analytic functions to avoid linear convergent points. Finally the exponential convergence is proved from the inverse Floquet-Bloch transform. Numerical results are also presented at the end of this paper.